Hostname: page-component-7f64f4797f-rcs6z Total loading time: 0 Render date: 2025-11-03T23:46:43.714Z Has data issue: false hasContentIssue false
  • English
  • Français

Invariant means on weakly almost periodic functionals with application to quantum groups

Part of: Topological algebras, normed rings and algebras, Banach algebras Abstract harmonic analysis Locally compact groups and their algebras

Published online by Cambridge University Press:  16 January 2023

Ali Ebrahimzadeh Esfahani ,
Mehdi Nemati  and
Mohammad Reza Ghanei
Ali Ebrahimzadeh Esfahani
Affiliation:
Department of Mathematical Sciences, Isfahan Uinversity of Technology, Isfahan 84156-83111, Iran e-mail: ali.ebrahimzadeh@math.iut.ac.ir
Mehdi Nemati*
Affiliation:
Department of Mathematical Sciences, Isfahan Uinversity of Technology, Isfahan 84156-83111, Iran e-mail: ali.ebrahimzadeh@math.iut.ac.ir
Mohammad Reza Ghanei
Affiliation:
Department of Mathematics, Khansar Campus, University of Isfahan, Isfahan, Iran e-mail: mrg.ghanei@gmail.com m.r.ghanei@khc.ui.ac.ir
*
e-mail: m.nemati@iut.ac.ir
Get access Rights & Permissions [Opens in a new window]

Abstract

Let 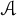${\mathcal A}$ be a Banach algebra, and let 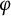$\varphi $ be a nonzero character on ${\mathcal A}$. For a closed ideal I of 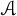${\mathcal A}$ with 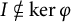$I\not \subseteq \ker \varphi $ such that I has a bounded approximate identity, we show that 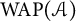$\operatorname {WAP}(\mathcal {A})$, the space of weakly almost periodic functionals on 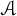${\mathcal A}$, admits a right (left) invariant 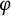$\varphi $-mean if and only if 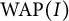$\operatorname {WAP}(I)$ admits a right (left) invariant 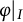$\varphi |_I$-mean. This generalizes a result due to Neufang for the group algebra 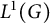$L^1(G)$ as an ideal in the measure algebra 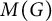$M(G)$, for a locally compact group G. Then we apply this result to the quantum group algebra 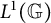$L^1({\mathbb G})$ of a locally compact quantum group 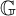${\mathbb G}$. Finally, we study the existence of left and right invariant 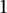$1$-means on 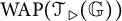$ \operatorname {WAP}(\mathcal {T}_{\triangleright }({\mathbb G}))$.

Keywords

Closed ideallocally compact quantum groupinvariant meanweakly almost periodic functional

MSC classification

Primary: 22D15: Group algebras of locally compact groups 43A07: Means on groups, semigroups, etc.; amenable groups 46H10: Ideals and subalgebras

Information

Type
Article
Information
Canadian Mathematical Bulletin , Volume 66 , Issue 3 , September 2023 , pp. 927 - 936
Check for updates
Copyright
© The Author(s), 2023. Published by Cambridge University Press on behalf of The Canadian Mathematical Society

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

References

Bédos, E. and Tuset, L., Amenability and co-amenability for locally compact quantum groups . Internat. J. Math. 14 (2003), 865–884.CrossRefGoogle Scholar
Dales, H. G. and Lau, A. T.-M., The second duals of Beurling algebras . Mem. Amer. Math. Soc. 177 (2005), 836.Google Scholar
Dales, H. G., Lau, A. T.-M., and Strauss, D., Second duals of measure algebras . Dissertationes Math. 481 (2012), 1–121.CrossRefGoogle Scholar
Desmedt, P., Quaegebeur, J., and Vaes, S., Amenability and the bicrossed product construction . Ill inois J. Math. 46 (2002), 1259–1277.Google Scholar
Forrest, B., Arens regularity and discrete groups . Pacific J. Math. 151 (1991), 217–227.CrossRefGoogle Scholar
Hu, Z., Neufang, M., and Ruan, Z.-J., Completely bounded multipliers over locally compact quantum groups . Proc. Lond. Math. Soc. 103 (2011), 1–39.CrossRefGoogle Scholar
Kaniuth, E., Lau, A. T.-M., and Pym, J., On character amenability of Banach algebras . J. Math. Anal. Appl. 344 (2008), 942–955.CrossRefGoogle Scholar
Kustermans, J. and Vaes, S., Locally compact quantum groups . Ann. Sci. Éc. Norm. Supér. (4) 33 (2000), 837–934.CrossRefGoogle Scholar
Kustermans, J. and Vaes, S., Locally compact quantum groups in the von Neumann algebraic setting . Math. Scand. 92 (2003), 68–92.CrossRefGoogle Scholar
Lau, A. T.-M., Analysis on a class of Banach algebras with applications to harmonic analysis on locally compact groups and semigroups . Fund. Math. 118 (1983), 161–175.Google Scholar
Neufang, M., Abstrakte harmonische analyse und Modulhomomorphismen $\ddot{{u}}$ ber von Neumann-algebren. Ph.D. thesis, Universität des Saarlande, 2000.Google Scholar
Neufang, M., Topological invariant means on almost periodic functionals: solution to problems by Dales–Lau–Strauss and daws . Proc. Amer. Math. Soc. 145 (2017), 3595–3598.CrossRefGoogle Scholar
Runde, V., Characterizations of compact and discrete quantum groups through second duals . J. Operator Theory 60 (2008), 415–428.Google Scholar
Runde, V., Amenable Banach algebras: a panorama. Springer Monographs in Mathematics, Springer, New York, 2020.CrossRefGoogle Scholar
Takesaki, M., Theory of operator algebras. Vol. 1, Springer, Berlin, 1979.CrossRefGoogle Scholar
Ulger, A., Arens regularity sometimes implies R.N.P . Pacific J. Math. 143 (1990), 377–399.CrossRefGoogle Scholar
Wong, J. C. S., Topologically stationary locally compact groups and amenability . Trans. Amer. Math. Soc. 144 (1969), 351–363.CrossRefGoogle Scholar
Young, N. J., Periodicity of functionals and representations of normed algebras on reflexive spaces . Proc. Edinb. Math. Soc. 20 (1976/77), 99–120.CrossRefGoogle Scholar