Article contents
Lattice Paths with Diagonal Steps
Published online by Cambridge University Press: 20 November 2018
Extract
The André-Poincaré "probléme du scrutin" [9] can be stated as follows: In an election between two candidates A polls m votes, B polls n, m > n. If the votes are counted one by one what is the probability that A leads B throughout the counting? Many derivations and interpretations of the solution 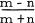 have been given and a convenient summary of methods till 1956 can be found in Feller [1]. So numerous are the generalizations of ballot problems and their applications since this date that we do not even attempt an enumeration here.
have been given and a convenient summary of methods till 1956 can be found in Feller [1]. So numerous are the generalizations of ballot problems and their applications since this date that we do not even attempt an enumeration here.
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 1969
References
- 8
- Cited by


