Hostname: page-component-78c5997874-t5tsf Total loading time: 0 Render date: 2024-11-10T15:30:43.945Z Has data issue: false hasContentIssue false
  • English
  • Français

Maps on Quantum States in 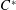$C^{\ast }$-algebras Preserving von Neumann Entropy or Schatten 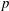$p$-norm of Convex Combinations

Part of: Special classes of linear operators

Published online by Cambridge University Press:  09 January 2019

Marcell Gaál
Marcell Gaál*
Affiliation:
Functional Analysis Research Group, University of Szeged, H-6720 Szeged, Hungary Email: marcell.gaal.91@gmail.com
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Very recently, Karder and Petek completely described maps on density matrices (positive semidefinite matrices with unit trace) preserving certain entropy-like convex functionals of any convex combination. As a result, maps could be characterized that preserve von Neumann entropy or Schatten 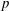$p$-norm of any convex combination of quantum states (whose mathematical representatives are the density matrices). In this note we consider these latter two problems on the set of invertible density operators, in a much more general setting, on the set of positive invertible elements with unit trace in a 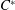$C^{\ast }$-algebra.

Keywords

density operatorentropySchatten p-normC∗ -algebranormalized tracepreserver

MSC classification

Primary: 47B49: Transformers, preservers (operators on spaces of operators)
Type
Article
Information
Canadian Mathematical Bulletin , Volume 62 , Issue 1 , March 2019 , pp. 75 - 80
Copyright
© Canadian Mathematical Society 2018 

Footnotes

This work was partially supported by the Hungarian National Research, Development and Innovation Office – NKFIH Reg.No. K115383.

References

Beneduci, R. and Molnár, L., On the standard K-loop structure of positive invertible elements in a C ∗-algebra . J. Math. Anal. Appl. 420(2014), 551–562. https://doi.org/10.1016/j.jmaa.2014.05.009.Google Scholar
Dixmier, J., Von Neumann algebras. North-Holland Mathematical Library, 27, North-Holland Publishing Company, Amsterdam-New York, 1981.Google Scholar
Fack, T. and Kosaki, H., Generalized s-numbers of 𝜏-measurable operators . Pacific J. Math. 123(1986), 269–300. https://doi.org/10.2140/pjm.1986.123.269.Google Scholar
Farenick, D., Jaques, S., and Rahaman, M., The fidelity of density operators in an operator-algebraic framework . J. Math. Phys. 57(2016), 102202. https://doi.org/10.1063/1.4965876.Google Scholar
Herstein, I. N., Jordan homomorphisms . Trans. Amer. Math. Soc. 81(1956), 331–341. https://doi.org/10.1090/S0002-9947-1956-0076751-6.Google Scholar
Jenčová, A., Geodesic distances on density matrices . J. Math. Phys. 45(2004), 1787–1794. https://doi.org/10.1063/1.1689000.Google Scholar
Kadison, R. V. and Ringrose, J. R., Fundamentals of the theory of operator algebras . Vol II., Pure and Applied Mathematics, 100, Academic Press, Orlando, FL, 1986.Google Scholar
Karder, M. and Petek, T., Maps on quaternion states preserving generalized entropy of convex combination . Linear Algebra Appl. 532(2017), 86–98. https://doi.org/10.1016/j.laa.2017.06.003.Google Scholar
Molnár, L., Maps on the positive definite cone of a C*-algebra preserving certain quasi-entropies . J. Math. Anal. Appl. 447(2017), 206–221. https://doi.org/10.1016/j.jmaa.2016.09.067.Google Scholar
Molnár, L. and Nagy, G., Transformations on density operators that leave the Holevo bound invariant . Int. J. Theor. Phys. 53(2014), 3273–3278. https://doi.org/10.1007/s10773-013-1638-8.Google Scholar
Palmer, T. W., Banach algebras and the general theory of ∗-algebras . Vol. I. Encyclopedia of Mathematics and its Applications, 49, Cambridge University Press, Cambridge, 1994. https://doi.org/10.1017/CBO9781107325777.Google Scholar