No CrossRef data available.
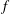 $f$-Ideals
$f$-IdealsPublished online by Cambridge University Press: 15 October 2018
A square-free monomial ideal 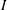 $I$ of
$I$ of 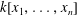 $k[x_{1},\ldots ,x_{n}]$ is said to be an
$k[x_{1},\ldots ,x_{n}]$ is said to be an 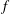 $f$-ideal if the facet complex and non-face complex associated with
$f$-ideal if the facet complex and non-face complex associated with 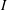 $I$ have the same
$I$ have the same 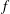 $f$-vector. We show that
$f$-vector. We show that  $I$ is an
$I$ is an 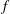 $f$-ideal if and only if its Newton complementary dual
$f$-ideal if and only if its Newton complementary dual 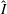 $\widehat{I}$ is also an
$\widehat{I}$ is also an  $f$-ideal. Because of this duality, previous results about some classes of
$f$-ideal. Because of this duality, previous results about some classes of  $f$-ideals can be extended to a much larger class of
$f$-ideals can be extended to a much larger class of 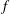 $f$-ideals. An interesting by-product of our work is an alternative formulation of the Kruskal–Katona theorem for
$f$-ideals. An interesting by-product of our work is an alternative formulation of the Kruskal–Katona theorem for 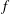 $f$-vectors of simplicial complexes.
$f$-vectors of simplicial complexes.
Current address for Budd: 2061 Oliver Road, Thunder Bay, ON, P7G 1P7
Parts of this paper appeared in the first author’s MSc project [4]. The second author’s research was supported in part by NSERC Discovery Grant 2014-03898.