Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
MOHANTY, GOPAL
1979.
Lattice Path Counting and Applications.
p.
63.

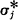 respectively denote the cyclic permutation (
respectively denote the cyclic permutation ( . Also denote by
. Also denote by 