Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Dowd, Martin
1991.
An Integer Program for Codes.
SIAM Journal on Discrete Mathematics,
Vol. 4,
Issue. 2,
p.
293.
Brink, David
Moree, Pieter
and
Osburn, Robert
2011.
Principal forms X 2+nY 2 representing many integers.
Abhandlungen aus dem Mathematischen Seminar der Universität Hamburg,
Vol. 81,
Issue. 2,
p.
129.

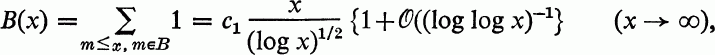
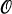 ((log x)
((log x)