Hostname: page-component-78c5997874-94fs2 Total loading time: 0 Render date: 2024-11-14T22:03:02.819Z Has data issue: false hasContentIssue false
  • English
  • Français

On Exceptional Polynomials

Published online by Cambridge University Press:  20 November 2018

Kenneth S. Williams
Kenneth S. Williams*
Affiliation:
Carleton University Ottawa
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let f(x) be a polynomial of degree d ≥ 2 defined over the finite field kq with q = pn elements. Let

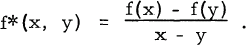
If f*(x, y) has no irreducible factor over kq which is absolutely irreducible, f is called an exceptional polynomial [1]. Davenport and Lewis have noted that when d is small compared with p, a permutation (substitution) polynomial is necessarily an exceptional polynomial. It is the purpose of this paper to prove the converse; that is, we will show the existence of a constant a(d), depending only on d, such that if f(x.) is an exceptional polynomial over kq, where p ≥ a(d), then f(x) is a permutation polynomial.

Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 11 , Issue 2 , June 1968 , pp. 279 - 282
Copyright
Copyright © Canadian Mathematical Society 1968

References

1. Davenport, H. and Lewis, D.J., Notes on congruences (I), Quart. J. Math. Oxford (2) 14 (1963), 51-60.Google Scholar
2. Williams, K.S., On extremal polynomials. Canad. Math. Bull. 10 (1967),585-594.Google Scholar