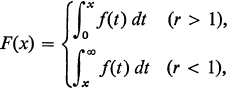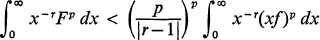Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Shum, D. T.
1974.
A General and Sharpened form of Opial's Inequality.
Canadian Mathematical Bulletin,
Vol. 17,
Issue. 3,
p.
385.
Shum, Daniel T.
1975.
On a class of new inequalities.
Transactions of the American Mathematical Society,
Vol. 204,
Issue. 0,
p.
299.
Imoru, Christopher Olutunde
1977.
On Some Integral Inequalities Related to Hardy's.
Canadian Mathematical Bulletin,
Vol. 20,
Issue. 3,
p.
307.
Chan, Ling-Yau
1979.
Some Extensions of Hardy's Inequality.
Canadian Mathematical Bulletin,
Vol. 22,
Issue. 2,
p.
165.
Lee, Cheng-Shyong
1980.
On Some Generalization of Inequalities of Opial, Yang and Shum.
Canadian Mathematical Bulletin,
Vol. 23,
Issue. 1,
p.
71.
Chan, Ling-Yau
1981.
Some Further Extensions of Hardy's Inequality.
Canadian Mathematical Bulletin,
Vol. 24,
Issue. 4,
p.
393.
Pachpatte, B.G
1987.
On some variants of Hardy's inequality.
Journal of Mathematical Analysis and Applications,
Vol. 124,
Issue. 2,
p.
495.
Pachpatte, B.G.
1987.
On a new class of Hardy type inequalities.
Proceedings of the Royal Society of Edinburgh: Section A Mathematics,
Vol. 105,
Issue. 1,
p.
265.
Pachpatte, B.G.
and
Love, E.R.
1990.
On some new inequalities related to Hardy's integral inequality.
Journal of Mathematical Analysis and Applications,
Vol. 149,
Issue. 1,
p.
17.
Mitrinović, D. S.
Pečarić, J. E.
and
Fink, A. M.
1991.
Inequalities Involving Functions and Their Integrals and Derivatives.
p.
143.
Mitrinović, D. S.
Pečarić, J. E.
and
Fink, A. M.
1991.
Inequalities Involving Functions and Their Integrals and Derivatives.
p.
66.
Li, Horng Jaan
and
Yeh, Cheh Chih
1995.
Inequalities for a function involving its integral and derivative.
Proceedings of the Royal Society of Edinburgh: Section A Mathematics,
Vol. 125,
Issue. 1,
p.
133.
Yang, Gou-Sheng
and
Hwang, Dah-Yan
1999.
Generalizations of Some Reverse Integral Inequalities.
Journal of Mathematical Analysis and Applications,
Vol. 233,
Issue. 1,
p.
193.
Cheung, Wing-Sum
Hanjš, Željko
and
Pečarić, Josip
2000.
Some Hardy-Type Inequalities.
Journal of Mathematical Analysis and Applications,
Vol. 250,
Issue. 2,
p.
621.
2005.
Mathematical Inequalities.
Vol. 67,
Issue. ,
p.
565.
2005.
Mathematical Inequalities.
Vol. 67,
Issue. ,
p.
485.
Oguntuase, James Adedayo
and
Persson, Lars-Erik
2008.
Refinement of Hardy's inequalities via superquadratic and subquadratic functions.
Journal of Mathematical Analysis and Applications,
Vol. 339,
Issue. 2,
p.
1305.
Abramovich, Shoshana
Krulić, Kristina
Pečarić, Josip
and
Persson, Lars-Erik
2010.
Some new refined Hardy type inequalities with general kernels and measures.
Aequationes mathematicae,
Vol. 79,
Issue. 1-2,
p.
157.
Adeleke, EO
Čižmešija, A
Oguntuase, JA
Persson, L-E
and
Pokaz, D
2012.
On a new class of Hardy-type inequalities.
Journal of Inequalities and Applications,
Vol. 2012,
Issue. 1,
Nasibullin, R.
2016.
Hardy type inequalities with weights dependent on the bessel functions.
Lobachevskii Journal of Mathematics,
Vol. 37,
Issue. 3,
p.
274.