Article contents
On L1-Convergence of Fourier Series under the MVBV Condition
Published online by Cambridge University Press: 20 November 2018
Abstract
Let 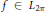 $f\in \,{{L}_{2\pi }}$ be a real-valued even function with its Fourier series
$f\in \,{{L}_{2\pi }}$ be a real-valued even function with its Fourier series 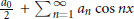 $\frac{{{a}_{0}}}{2}\,+\,\sum _{n=1}^{\infty }\,{{a}_{n}}\,\cos \,nx$, and let
$\frac{{{a}_{0}}}{2}\,+\,\sum _{n=1}^{\infty }\,{{a}_{n}}\,\cos \,nx$, and let 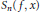 ${{S}_{n}}\left( f,x \right)$,
${{S}_{n}}\left( f,x \right)$, 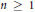 $n\,\,\ge \,\,1$, be the
$n\,\,\ge \,\,1$, be the  $n$-th partial sum of the Fourier series. It is well known that if the nonnegative sequence
$n$-th partial sum of the Fourier series. It is well known that if the nonnegative sequence 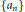 $\{{{a}_{n}}\}$ is decreasing and
$\{{{a}_{n}}\}$ is decreasing and  ${{\lim }_{n\to \infty }}\,{{a}_{n}}\,=\,0$, then
${{\lim }_{n\to \infty }}\,{{a}_{n}}\,=\,0$, then
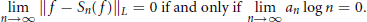 $$\underset{n\to \infty }{\mathop{\lim }}\,{{\left\| f-{{S}_{n}}\left( f \right) \right\|}_{L}}=0\text{ifanyonlyif}\underset{n\to \infty }{\mathop{\lim }}\,{{a}_{n}}\log n=0.$$
$$\underset{n\to \infty }{\mathop{\lim }}\,{{\left\| f-{{S}_{n}}\left( f \right) \right\|}_{L}}=0\text{ifanyonlyif}\underset{n\to \infty }{\mathop{\lim }}\,{{a}_{n}}\log n=0.$$
We weaken the monotone condition in this classical result to the so-called mean value bounded variation (MVBV) condition. The generalization of the above classical result in real-valued function space is presented as a special case of the main result in this paper, which gives the 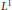 ${{L}^{1}}$-convergence of a function
${{L}^{1}}$-convergence of a function 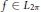 $f\in {{L}_{2\pi }}$ in complex space. We also give results on
$f\in {{L}_{2\pi }}$ in complex space. We also give results on 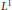 ${{L}^{1}}$-approximation of a function
${{L}^{1}}$-approximation of a function 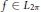 $f\in {{L}_{2\pi }}$ under the MVBV condition.
$f\in {{L}_{2\pi }}$ under the MVBV condition.
Keywords
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 2009
References
- 4
- Cited by


