Hostname: page-component-cd9895bd7-p9bg8 Total loading time: 0 Render date: 2024-12-26T15:35:03.137Z Has data issue: false hasContentIssue false
  • English
  • Français

On Projectively Flat (α, β)-metrics

Published online by Cambridge University Press:  20 November 2018

Zhongmin Shen
Zhongmin Shen*
Affiliation:
Center for Mathematical Sciences, Zhejiang University, Hangzhou, Zhejiang Province 310027, P.R. China e-mail: zshen@math.iupui.edu
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

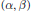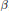The solutions to Hilbert's Fourth Problem in the regular case are projectively flat Finsler metrics. In this paper, we consider the so-called $\left( \alpha ,\,\beta \right)$-metrics defined by a Riemannian metric $\alpha$ and a 1-form $\beta$, and find a necessary and sufficient condition for such metrics to be projectively flat in dimension $n\,\ge \,3$.

Keywords

53B4053C60
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 52 , Issue 1 , 01 March 2009 , pp. 132 - 144
Copyright
Copyright © Canadian Mathematical Society 2009

References

[1] Chern, S.-S. and Shen, Z., Riemann-Finsler geometry. Nankai Tracts in Mathematics 6, World Scientific Publishing, Hackensack, NJ, 2005.Google Scholar
[2] Cheng, X. and Li, M., On a class of projectively flat (α, β)-metrics. Publ. Math. Debrecen 71(2007), no. 1–2, 195–205.Google Scholar
[3] Bácsó, S. and Matsumoto, M., On Finsler spaces of Douglas type–a generalization of the notion of Berwald space. Publ. Math. Debrecen 51(1997), no. 3–4, 385–406.Google Scholar
[4] Hamel, G., Über die Geometrieen in denen die Geraden die Kürzesten sind. Math. Ann. 57(1903), no. 2, 231–264.Google Scholar
[5] Li, B., Projectively flat Matsumoto metric and its approximation. ActaMath. Sci. Ser. B Engl. Ed. 27(2007), no. 4, 781–789.Google Scholar
[6] Mo, X., Shen, Z. and Yang, C., Some constructions of projectively flat Finsler metrics. Sci. China Ser. A, 49(2006), mp. 5, 703–714.Google Scholar
[7] Shen, Y. and Zhao, L., Some projectively flat (α, β)-metrics.. Sci. China Ser. A 49(2006), no. 6, 838–851.Google Scholar
[8] Shen, Z., Projectively flat Randers metrics with constant flag curvature. Math. Ann. 325(2003), no. 1, 19–30.Google Scholar
[9] Shen, Z. and Yildirim, G. C., On a class of projectively flat metrics with constant flag curvature. Canad. J. Math. 60(2008), no. 2, 443–456.Google Scholar
[10] Yu, Y., Projectively flat exponential Finsler metric. J. Zhejiang Univ. Science A, 7(2006), no. 6, 1068–1076.Google Scholar
[11] Yu, Y., Projectively flat arctangent Finsler metric. J. Zhejiang Univ. Science A, 7(2006), no. 12, 2097–2103.Google Scholar