Hostname: page-component-78c5997874-g7gxr Total loading time: 0 Render date: 2024-11-15T06:36:23.118Z Has data issue: false hasContentIssue false
  • English
  • Français

On Stability of Solutions of Certain Differential Equations of the Third Order

Published online by Cambridge University Press:  20 November 2018

B.S. Lalli
B.S. Lalli*
Affiliation:
University of Saskatchewan, Saskatoon and Canadian Mathematical Congress, Summer Research Institute
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

The purpose of this paper is to obtain a set of sufficient conditions for “global asymptotic stability” of the trivial solution x = 0 of the differential equation

1.1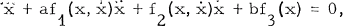

using a Lyapunov function which is substantially different from similar functions used in [2], [3] and [4], for similar differential equations. The functions f1, f2 and f3 are real - valued and are smooth enough to ensure the existence of the solutions of (1.1) on [0, ∞). The dot indicates differentiation with respect to t. We are taking a and b to be some positive parameters.

Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 10 , Issue 5 , December 1967 , pp. 681 - 688
Copyright
Copyright © Canadian Mathematical Society 1967

References

1. Barbasin, E.A., On the stability of certain nonlinear equations of the third order. Priklad. Mat. Mech. 16. 629-632 (1955).Google Scholar
2. Ezeiolo, J. O. C., On the stability of solutions of certain differential equations of the third order. Quart. J. Math. Oxfor. (2), 11 (1966), 64-69.Google Scholar
3. Haas, V., A stability result for a third order nonlinear differential equation. J. London Math. Soc. 40 (1966), 31-33.Google Scholar
4. Simanov, S. N., On stability of solutions of a nonlinear differential equation of third order. Priklad. Mat. Mech., 17 (1955), 369-37Z.Google Scholar