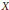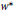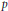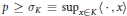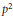Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Cheng, Lixin
Cheng, Qingjin
and
Zhang, Jichao
2015.
On super fixed point property and super weak compactness of convex subsets in Banach spaces.
Journal of Mathematical Analysis and Applications,
Vol. 428,
Issue. 2,
p.
1209.
Cheng, Lixin
Tu, Kun
and
Zhang, Wen
2016.
On weak stability of ε-isometries on wedges and its applications.
Journal of Mathematical Analysis and Applications,
Vol. 433,
Issue. 2,
p.
1673.
Ablet, Ehmet
Cheng, Lixin
Cheng, Qingjin
and
Zhang, Wen
2019.
Every Banach space admits a homogenous measure of non-compactness not equivalent to the Hausdorff measure.
Science China Mathematics,
Vol. 62,
Issue. 1,
p.
147.
Shen, Qinrui
2020.
A Viewpoint to Measure of Non-Compactness of Operators in Banach Spaces.
Acta Mathematica Scientia,
Vol. 40,
Issue. 3,
p.
603.
Cheng, Li Xin
Cheng, Qing Jin
and
Wang, Jian Jian
2021.
On Absolute Uniform Retracts, Uniform Approximation Property and Super Weakly Compact Sets of Banach Spaces.
Acta Mathematica Sinica, English Series,
Vol. 37,
Issue. 5,
p.
731.
Sun, Longfa
2021.
A note on stability of non-surjective $$\varepsilon$$-isometries between the positive cones of $$L^p$$-spaces.
Indian Journal of Pure and Applied Mathematics,
Vol. 52,
Issue. 4,
p.
1085.
Sun, Longfa
2021.
A note on the stability of isometries on the positive cones of continuous function spaces.
Indian Journal of Pure and Applied Mathematics,
Vol. 52,
Issue. 1,
p.
265.
Vestfrid, Igor A.
2023.
ε-isometries between the positive cones of continuous functions spaces.
Israel Journal of Mathematics,
Vol. 253,
Issue. 2,
p.
989.
Chen, Xiaoling
and
Cheng, Lixin
2023.
Representation of measures of noncompactness and its applications related to an initial value problem in Banach spaces.
Science China Mathematics,
Vol. 66,
Issue. 4,
p.
745.