No CrossRef data available.
Published online by Cambridge University Press: 20 November 2018
Let Y be a linear space over the complex plane C, and let F be a mapping on the complex linear space Y ⊕ C into subsets of C with the following properties: for y ∊ Y, λ and μ ∊ C, F(y + μ) is a nonempty and bounded subset of C, F(λy + μ) = λF(y) + μ and F(μ) = {μ}. We shall write f(y + μ) = sup{|λ + μ|: λ ∊ F(y)}, the radius of F(y + μ), y ∊ Y and μ ∊ C. The convex hull (resp. the closure) of a subset M of C is denoted by conv M (resp. 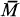 ).
).