Hostname: page-component-cd9895bd7-gxg78 Total loading time: 0 Render date: 2024-12-27T21:20:36.707Z Has data issue: false hasContentIssue false
  • English
  • Français

On the Comaximal Graph of a Commutative Ring

Published online by Cambridge University Press:  20 November 2018

Karim Samei
Karim Samei*
Affiliation:
Department of Mathematics, Bu Ali Sina University, Hamedan, Iran and School of Mathematics, Institute for Research in Fundamental Science (IPM), Tehran, Iran e-mail: samei@ipm.ir
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

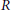Let $R$ be a commutative ring with 1. In a 1995 paper in J. Algebra, Sharma and Bhatwadekar defined a graph on 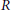$R$, 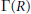$\Gamma \left( R \right)$, with vertices as elements of 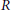$R$, where two distinct vertices $a$ and $b$ are adjacent if and only if 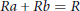$Ra\,+\,Rb\,=\,R$. In this paper, we consider a subgraph ${{\Gamma }_{2}}\left( R \right)$ of 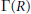$\Gamma \left( R \right)$ that consists of non-unit elements. We investigate the behavior of ${{\Gamma }_{2}}\left( R \right)$ and 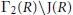${{\Gamma }_{2}}\left( R \right)\backslash \text{J}\left( R \right)$, where 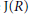$\text{J}\left( R \right)$ is the Jacobson radical of 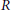$R$. We associate the ring properties of 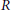$R$, the graph properties of ${{\Gamma }_{2}}\left( R \right)$, and the topological properties of 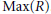$\text{Max}\left( R \right)$. Diameter, girth, cycles and dominating sets are investigated, and algebraic and topological characterizations are given for graphical properties of these graphs.

Keywords

13A99comaximaldiametergirthcyclesdominating set
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 57 , Issue 2 , 14 June 2014 , pp. 413 - 423
Copyright
Copyright © Canadian Mathematical Society 2014

Footnotes

This research was in part supported by a grant from IPM (No 89130043).

References

[1] Akbari, S., Kiani, D., Mohammadi, F., and Moradi, S., The total graph and regular graph of commutative ring. J. Pure Appl. Algebra 213 (2009, no. 12, 2224–2228.http://dx.doi.org/10.1016/j.jpaa.2009.03.013 Google Scholar
[2] Anderson, D. F. and Badawi, A. The total graph of commutative ring. J. Algebra 320 (2008, no. 7, 2706–2719.http://dx.doi.org/10.1016/j.jalgebra.2008.06.028 Google Scholar
[3] Anderson, D. F., Levy, R. and Shapiro, J. Zero-divisor graphs, von Neumann regular rings, and Boolean algebras. J. Pure Appl. Algebra 180 (2003, no. 3, 221–241.http://dx.doi.org/10.1016/S0022-4049(02)00250-5 Google Scholar
[4] Anderson, D. F. and Livingston, P. S., The zero-divisor graph of commutative ring. J. Algebra 217 (1999, no. 2, 434–447.http://dx.doi.org/10.1006/jabr.1998.7840 Google Scholar
[5] Beck, I., Coloring of commutative rings. J. Algebra 116 (1988, no. 1, 208–226.http://dx.doi.org/10.1016/0021-8693(88)90202-5 CrossRefGoogle Scholar
[6] De Marco, G. and Orsatti, A., Commutative rings in which every prime ideal is contained in a unique maximal ideal. Proc. Amer. Math. Soc 30 (1971, 459–466.http://dx.doi.org/10.1090/S0002-9939-1971-0282962-0 Google Scholar
[7] Diestel, R., Graph theory. Second ed., Graduate Texts in Mathematics, 173, Springer-Verlag, New York, 2000.Google Scholar
[8] Engelking, R., General topology. Second ed., Sigma Series in Pure Mathematics, 6, Heldermann Verlag, Berlin, 1989.Google Scholar
[9] Gillman, L. and Jerison, M., Rings of continuous functions. Graduate Texts in Mathematics, 43, Springer-Verlag, New York-Heidelberg, 1976.Google Scholar
[10] Maimani, H. R., Salimi, M., Sattari, A., and Yassemi, S., Comaximal graph of commutative rings. J. Algebra 319 (2008, no. 4, 1801–1808. http://dx.doi.org/10.1016/j.jalgebra.2007.02.003 Google Scholar
[11] K. Samei, z0-ideals and some special commutative rings. Fund. Math 189 (2006, no. 2, 99–109. http://dx.doi.org/10.4064/fm189-2-1Google Scholar
[12] Maimani, H. R., Salimi, M., Sattari, A., and Yassemi, S., The zero-divisor graph of a reduced ring. J. Pure Appl. Algebra 209 (2007, no. 3, 813–821. http://dx.doi.org/10.1016/j.jpaa.2006.08.008 Google Scholar
[13] Maimani, H. R., Salimi, M., Sattari, A., and Yassemi, S., On the maximal spectrum of semiprimitive multiplication modules. Canad. Math. Bull 51 (2008, no. 3, 439–447.http://dx.doi.org/10.4153/CMB-2008-044-8 Google Scholar
[14] Sharma, P. K. and Bhatwadekar, S. M., A note on graphical representation of rings. J. Algebra 176 (1995, no. 1, 124–127.http://dx.doi.org/10.1006/jabr.1995.1236 Google Scholar
[15] Wang, H.-J., Graphs associated to co-maximal ideals of commutative rings. J. Algebra 320 (2008, no. 7, 2917–2933.http://dx.doi.org/10.1016/j.jalgebra.2008.06.020 Google Scholar
[16] Wang, H.-J., Co-maximal graph of non-commutative rings. Linear Algebra Appl. 430 (2009, no. 2–3, 633–641.http://dx.doi.org/10.1016/j.laa.2008.08.026 Google Scholar