No CrossRef data available.
Published online by Cambridge University Press: 29 May 2023
In 2005, N. Nikolski proved among other things that for any 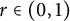 $r\in (0,1)$ and any
$r\in (0,1)$ and any 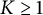 $K\geq 1$, the condition number
$K\geq 1$, the condition number 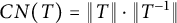 $CN(T)=\Vert T\Vert \cdot \Vert T^{-1}\Vert $ of any invertible n-dimensional complex Banach space operators T satisfying the Kreiss condition, with spectrum contained in
$CN(T)=\Vert T\Vert \cdot \Vert T^{-1}\Vert $ of any invertible n-dimensional complex Banach space operators T satisfying the Kreiss condition, with spectrum contained in 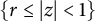 $\left \{ r\leq |z|<1\right \}$, satisfies the inequality
$\left \{ r\leq |z|<1\right \}$, satisfies the inequality 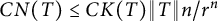 $CN(T)\leq CK(T)\Vert T \Vert n/r^{n}$ where
$CN(T)\leq CK(T)\Vert T \Vert n/r^{n}$ where 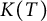 $K(T)$ denotes the Kreiss constant of T and
$K(T)$ denotes the Kreiss constant of T and 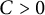 $C>0$ is an absolute constant. He also proved that for
$C>0$ is an absolute constant. He also proved that for 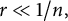 $r\ll 1/n,$ the latter bound is asymptotically sharp as
$r\ll 1/n,$ the latter bound is asymptotically sharp as 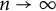 $n\rightarrow \infty $. In this note, we prove that this bound is actually achieved by a family of explicit
$n\rightarrow \infty $. In this note, we prove that this bound is actually achieved by a family of explicit 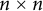 $n\times n$ Toeplitz matrices with arbitrary singleton spectrum
$n\times n$ Toeplitz matrices with arbitrary singleton spectrum 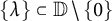 $\{\lambda \}\subset \mathbb {D}\setminus \{0\}$ and uniformly bounded Kreiss constant. Independently, we exhibit a sequence of Jordan blocks with Kreiss constants tending to
$\{\lambda \}\subset \mathbb {D}\setminus \{0\}$ and uniformly bounded Kreiss constant. Independently, we exhibit a sequence of Jordan blocks with Kreiss constants tending to 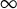 $\infty $ showing that Nikolski’s inequality is still asymptotically sharp as K and n go to
$\infty $ showing that Nikolski’s inequality is still asymptotically sharp as K and n go to 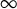 $\infty $.
$\infty $.
Charpentier was partly supported by the grant ANR-17-CE40-0021 of the Agence Nationale pour la Recherche ANR. Zarouf acknowledges financial support by the Agence Nationale pour la Recherche grant ANR-18-CE40-0035.