Hostname: page-component-cd9895bd7-q99xh Total loading time: 0 Render date: 2024-12-25T17:28:34.308Z Has data issue: false hasContentIssue false
  • English
  • Français

On the Maximal Spectrum of Semiprimitive Multiplication Modules

Published online by Cambridge University Press:  20 November 2018

Karim Samei
Karim Samei*
Affiliation:
Department of Mathematics, Bu Ali Sina University, Hamedan, Iran. e-mail: samei@ipm.ir Institute for Studies in Theoretical Physics and Mathematics (IPM), Tehran, Iran
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

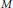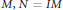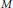An $R$-module $M$ is called a multiplication module if for each submodule $N$ of $M,\,N\,=\,IM$ for some ideal $I$ of $R$. As defined for a commutative ring $R$, an $R$-module $M$ is said to be semiprimitive if the intersection of maximal submodules of 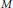$M$ is zero. The maximal spectra of a semiprimitive multiplication module 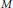$M$ are studied. The isolated points of 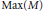$\text{Max}\left( M \right)$ are characterized algebraically. The relationships among the maximal spectra of 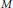$M$, 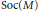$\text{Soc}\left( M \right)$ and 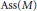$\text{Ass}\left( M \right)$ are studied. It is shown that 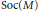$\text{Soc}\left( M \right)$ is exactly the set of all elements of 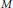$M$ which belongs to every maximal submodule of 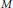$M$ except for a finite number. If 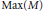$\text{Max}\left( M \right)$ is infinite, 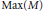$\text{Max}\left( M \right)$ is a one-point compactification of a discrete space if and only if 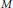$M$ is Gelfand and for some maximal submodule $K$, 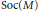$\text{Soc}\left( M \right)$ is the intersection of all prime submodules of 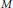$M$ contained in $K$. When 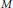$M$ is a semiprimitive Gelfand module, we prove that every intersection of essential submodules of 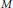$M$ is an essential submodule if and only if 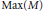$\text{Max}\left( M \right)$ is an almost discrete space. The set of uniform submodules of 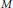$M$ and the set of minimal submodules of 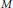$M$ coincide. 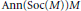$\text{Ann}\left( \text{Soc}\left( M \right) \right)M$ is a summand submodule of 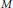$M$ if and only if 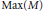$\text{Max}\left( M \right)$ is the union of two disjoint open subspaces 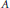$A$ and $N$, where 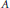$A$ is almost discrete and $N$ is dense in itself. In particular, 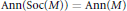$\text{Ann}\left( \text{Soc}\left( M \right) \right)\,=\,\text{Ann}\left( M \right)$ if and only if 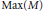$\text{Max}\left( M \right)$ is almost discrete.

Keywords

13C13multiplication modulesemiprimitive moduleGelfand moduleZariski topology
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 51 , Issue 3 , 01 September 2008 , pp. 439 - 447
Copyright
Copyright © Canadian Mathematical Society 2008

References

[1] Anderson, D. D. and Al-Shania, Y., Multiplication modules and the ideal θ(M). Comm. Algebra 30(2002), no. 7, 3383–3390.Google Scholar
[2] Azarpanah, F., Algebraic properties of some compact spaces. Real Anal. Exchange 25(1999/00), no. 1, 317–327.Google Scholar
[3] De Marco, G. and Orsatti, A., Commutative rings in which every prime ideal is contained in a unique maximal ideal. Proc. Amer. Math. Soc 30(1971), 459–466.Google Scholar
[4] El-Bast, Z. and Smith, P. F., Multiplication modules. Comm. Algebra 16(1988), no. 4, 755–779.Google Scholar
[5] Gillman, L. and Jerison, M., Rings of Continuous Functions. Graduate Texts in Mathematics 43, Springer-Verlag, New York, 1976.Google Scholar
[6] Lu, C. P., Spectra of modules. Comm. Algebra 23(1995), no. 10, 3741–3752.Google Scholar
[7] McCasland, R. L. and Moore, M. E., On radicals of submodules of finitely generated modules. Canad. Math. Bull 29(1986), no. 1, 37–39.Google Scholar
[8] McCasland, R. L., Moore, M. E., and Smith, P. F., On the spectrum of a module over a commutative ring. Comm. Algebra 25(1997), no. 1, 79–103.Google Scholar
[9] Samei, K., On the maximal spectrum of commutative semiprimitive rings. Coll. Math 83(2000), no. 1, 5–13.Google Scholar
[10] Samei, K., Reduced multiplication modules. J. Austral. Math. Soc (to appear).Google Scholar
[11] Smith, P. F., Some remarks on multiplication modules. Arch. Math (Basel) 50(1988), no. 3, 223–235.Google Scholar
[12] Zhang, G., Wang, F., and Tong, W.,Multiplication modules in which every prime submodule is contained in a unique maximal submodule. Comm. Algebra 32(2004), no. 5, 1945–1959.Google Scholar