Hostname: page-component-cd9895bd7-8ctnn Total loading time: 0 Render date: 2024-12-27T05:16:16.413Z Has data issue: false hasContentIssue false
  • English
  • Français

On the Notion of Visibility of Torsors

Published online by Cambridge University Press:  20 November 2018

Amod Agashe
Amod Agashe*
Affiliation:
Department of Mathematics, Florida State University, Tallahassee, FL, U.S.A. e-mail: agashe@math.fsu.edu
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let $J$ be an abelian variety and $A$ be an abelian subvariety of $J$ , both defined over $Q$. Let 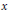$x$ be an element of 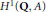${{H}^{1}}\left( Q,\,A \right)$. Then there are at least two definitions of 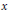$x$ being visible in $J$: one asks that the torsor corresponding to 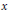$x$ be isomorphic over $Q$ to a subvariety of $J$, and the other asks that 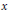$x$ be in the kernel of the natural map 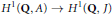$ {{H}^{1}}\left( Q,\,A \right)\,\to \,{{H}^{1}}\left( \text{Q},\,J \right)$. In this article, we clarify the relation between the two definitions.

Keywords

11G3514G25torsorsprincipal homogeneous spacesvisibilityShafarevich-Tate group
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 56 , Issue 2 , 01 June 2013 , pp. 225 - 228
Copyright
Copyright © Canadian Mathematical Society 2013

References

[1] Agashe, A., On invisible elements of the Tate-Shafarevich group. C. R. Acad. Sci. Paris Sér. I Math. 328(1999), no. 5, 369–374. Google Scholar
[2] Agashe, A., A visible factor of the Heegner index. Math. Res. Lett. 17(2010), no. 6, 1065–1077. Google Scholar
[3] Agashe, A., Visibility and the Birch and Swinnerton-Dyer conjecture for analytic rank zero. arxiv:0908.3823.Google Scholar
[4] Agashe, A., Visibility and the Birch and Swinnerton-Dyer conjecture for analytic rank one. Int. Math. Res. Not. (IMRN) 15(2009), 2899–2913. Google Scholar
[5] Agashe, A., A visible factor of the special L-value. J. Reine Angew. Math. 644(2010), 159–187. http://dx.doi.org/10.1515/CRELLE.2010.055 Google Scholar
[6] Agashe, A., Ribet, K., and Stein, W. A., The modular degree, congruence primes, and multiplicity one. To appear in a special Springer volume in honor of Serge Lang, http://www.math.fsu.edu/_agashe/moddeg3.html Google Scholar
[7] Agashe, A. and Stein, W., Visible evidence for the Birch and Swinnerton-Dyer conjecture for modular abelian varieties of analytic rank zero. Math. Comp. 74(2005), no. 249, 455–484. http://dx.doi.org/10.1090/S0025-5718-04-01644-8 Google Scholar
[8] Cremona, J. E. and Mazur, B., Visualizing elements in the Shafarevich-Tate group. Experiment. Math. 9(2000), no. 1, 13–28. Google Scholar
[9] Mazur, B., Three lectures about the arithmetic of elliptic curves. Handout at the ArizonaWinter School, http://swc.math.arizona.edu/notes/NotesAll.html. Google Scholar
[10] Milne, J. S., Etale cohomology. Princeton Mathematical Series, 33, Princeton University Press,Google Scholar