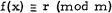Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
McCann, K.
and
Williams, K. S.
1968.
Cubic polynomials with the same residues (mod p).
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 64,
Issue. 3,
p.
655.
Williams, Kenneth S.
1968.
Note on Pairs of Consecutive Residues of Polynomials.
Canadian Mathematical Bulletin,
Vol. 11,
Issue. 1,
p.
79.
Williams, Kenneth S.
1969.
On Two Conjectures of Chowla.
Canadian Mathematical Bulletin,
Vol. 12,
Issue. 5,
p.
545.
Mit'kin, D. A.
1985.
Polynomials with minimal set of values and the equationf (x) = f (y) in a finite prime field.
Mathematical Notes of the Academy of Sciences of the USSR,
Vol. 38,
Issue. 1,
p.
513.
Sun, Zhi-Hong
2006.
On the number of incongruent residues of x4+ax2+bx modulo p.
Journal of Number Theory,
Vol. 119,
Issue. 2,
p.
210.
Bialostocki, Arie
and
Luong, Tran Dinh
2014.
Cubic symmetric polynomials yielding variations of the Erdős–Ginzburg–Ziv theorem.
Acta Mathematica Hungarica,
Vol. 142,
Issue. 1,
p.
152.