Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Kuo, Chung-Cheng
2004.
One-sided multiple resonance for quasilinear elliptic partial differential equations.
Applicable Analysis,
Vol. 83,
Issue. 9,
p.
897.

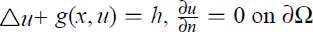 in which the non-linearity
in which the non-linearity 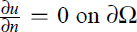 in which the nonlinear term g may grow superlinearly in
in which the nonlinear term g may grow superlinearly in  are arbitrarily nonnegative constants,
are arbitrarily nonnegative constants,  . The proofs are based upon degree theoretic arguments.
. The proofs are based upon degree theoretic arguments.