Hostname: page-component-78c5997874-mlc7c Total loading time: 0 Render date: 2024-11-10T21:23:09.865Z Has data issue: false hasContentIssue false
  • English
  • Français

On the Structure of the Schild Group in Relativity Theory

Published online by Cambridge University Press:  20 November 2018

Gerd Jensen  and
Christian Pommerenke
Gerd Jensen
Affiliation:
Sensburger Allee 22 a, D–14055 Berlin e-mail: cg.jensen@arcor.de
Christian Pommerenke
Affiliation:
Institut für Mathematik, Technische Universität, D–10623 Berlin e-mail: pommeren@math.tu-berlin.de
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Alfred Schild has established conditions that Lorentz transformationsmap world-vectors 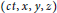$(ct,x,y,z)$ with integer coordinates onto vectors of the same kind. These transformations are called integral Lorentz transformations.

This paper contains supplements to our earlier work with a new focus on group theory. To relate the results to the familiar matrix group nomenclature, we associate Lorentz transformations with matrices in 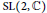$\text{SL}(2,\mathbb{C})$. We consider the lattice of subgroups of the group originated in Schild's paper and obtain generating sets for the full group and its subgroups.

Keywords

22E4320H9983A05Lorentz transformationinteger latticeGaussian integersSchild groupsubgroup
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 60 , Issue 4 , 01 December 2017 , pp. 774 - 790
Copyright
Copyright © Canadian Mathematical Society 2017

References

[1] Baker, A., Matrix groups. An introduction to Lie group theory. Springer-Verlag, London, 2002. http://dx.doi.org/10.1007/978-1-4471-0183-3 Google Scholar
[2] Fine, B. and M. Newman, The normal subgroup structure of the Picard group. Trans. Amer. Math. Soc. 302(1987), no. 2, 769–786. http://dx.doi.org/10.1090/S0002-9947-1987-0891646-3 Google Scholar
[3] Goldstein, H., Classical mechanics. 2nd edition. Addison-Wesley, Reading, MA, 1980. Google Scholar
[4] Jensen, G. and C. Pommerenke, Discrete space-time and Lorentz transformations. Canad Math. Bull. 59(2016), no. 2, 123–135. http://dx.doi.org/10.41 53/CMB-2O1 5-066-4 Google Scholar
[5] LorenteandP, M.. Kramer, Representations of the discrete inhomogeneous Lorentz group and Dirac wave equation on the lattice. J. Phys. A 32(1999), 2481–2497. http://dx.doi.org/1 0.1088/0305-4470/32/12/01 9 Google Scholar
[6] Louck, J. D., A new parametrization and all integral realizations of the Lorentz group. J. Math. Phys. 43(2002), no. 10, 5108–5134. http://dx.doi.org/10.1063/1.1505124 Google Scholar
[7] Penrose, R. and W. Rindler, Spinors and space-time. Volume I. Cambridge University Press, Cambridge, 1984. http://dx.doi.org/10.1 01 7/CBO9780511 564048 Google Scholar
[8] Schild, A., Discrete space-time and integral Lorentz transformations. Canad. J. Math. 1(1949), 29–47. http://dx.doi.org/! 0.41 53/CJM-1 949-003-4 Google Scholar