Hostname: page-component-78c5997874-ndw9j Total loading time: 0 Render date: 2024-11-11T00:17:31.949Z Has data issue: false hasContentIssue false
  • English
  • Français

Periodic and Nil Polynomials in Rings

Published online by Cambridge University Press:  20 November 2018

Bernardo Felzenszwalb  and
Antonino Giambruno
Bernardo Felzenszwalb
Affiliation:
Instituto de Matematica, Universidade Federal do Rio de Janeiro, C.P. 1835 ZC-00 20.000 Rio de Janeiro, R. J. Brazil
Antonino Giambruno
Affiliation:
Istituto di Matematica, Università di Palermo, Via Archirafi 34 90100 Palermo, Italy
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let R be an associative ring and f(x1,…, xd) a polynomial in noncommuting variables. We say that f is periodic or nil in R if for all r1,…, rd ∈ R we have that f(r1,…, rd) is periodic, respectively nilpotent (recall that a ∈ R is periodic if for some integer ).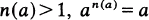

Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 23 , Issue 4 , 01 December 1980 , pp. 473 - 476
Copyright
Copyright © Canadian Mathematical Society 1980

References

1. Felzenszwalb, B. and Giambruno, A., Centralizers and multilinear polynomials in noncommutative rings, J. London Math. Soc. (2), 19 (1979), 417-428.Google Scholar
2. Herstein, I. N., Procesi, C. and Schacher, M., Algebraic valued functions on noncommutative rings, J. Algebra, 36 (1975), 128-150.Google Scholar
3. Leron, U., Nil and power-central polynomials in rings, Trans. Amer. Math. Soc, 202 (1975), 97-103.Google Scholar
4. Martindale, W. S. 3rd, Prime rings satisfying a generalized polynomial identity, J. Algebra, 12 (1969), 186-194.Google Scholar
5. Smith, M., Rings with an integral element whose centralizer satisfies a polynomial identity, Duke Math. J., 42 (1975), 137-149.Google Scholar