No CrossRef data available.
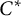 $C^*$-algebras induced by equivalence bimodules
$C^*$-algebras induced by equivalence bimodulesPublished online by Cambridge University Press: 06 July 2021
For two  $\sigma $
-unital
$\sigma $
-unital 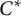 $C^*$
-algebras, we consider two equivalence bimodules over them, respectively. Then, by taking the crossed products by the equivalence bimodules, we get two inclusions of
$C^*$
-algebras, we consider two equivalence bimodules over them, respectively. Then, by taking the crossed products by the equivalence bimodules, we get two inclusions of 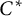 $C^*$
-algebras. Furthermore, we suppose that one of the inclusions of
$C^*$
-algebras. Furthermore, we suppose that one of the inclusions of  $C^*$
-algebras is irreducible, that is, the relative commutant of one of the
$C^*$
-algebras is irreducible, that is, the relative commutant of one of the  $\sigma $
-unital
$\sigma $
-unital 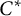 $C^*$
-algebras in the multiplier
$C^*$
-algebras in the multiplier 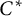 $C^*$
-algebra of the crossed product is trivial. We will give a sufficient and necessary condition that the two inclusions are strongly Morita equivalent. Applying this result, we will compute the Picard group of a unital inclusion of unital
$C^*$
-algebra of the crossed product is trivial. We will give a sufficient and necessary condition that the two inclusions are strongly Morita equivalent. Applying this result, we will compute the Picard group of a unital inclusion of unital 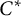 $C^*$
-algebras induced by an equivalence bimodule over the unital
$C^*$
-algebras induced by an equivalence bimodule over the unital 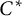 $C^*$
-algebra under the assumption that the unital inclusion of unital
$C^*$
-algebra under the assumption that the unital inclusion of unital 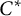 $C^*$
-algebras is irreducible.
$C^*$
-algebras is irreducible.