No CrossRef data available.
Published online by Cambridge University Press: 20 November 2018
Let τ:[0, 1] → [0, 1] be defined by τ(x) = 2x on [0, 1/2,] and τ(JC) = 2(1 - x) on [5, 1], and let T:[0, 1] x [0, 1] → [0, 1] x [0, 1] be defined by T(x,y) = (τ(x), τ(y))- Let
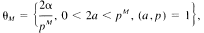
where p is a prime > 2, and a and M are integers. Consider T restricted to θM x θN, 1 < M < N. Let X = ((2a)/(pM), (2b)/(pN)) ∈ θM x θN and let per(X) denote the length of the period of X.
Then,
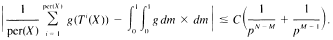
where m is Lebesque measure on [0, 1], and C is independent of p, N, M, a and b. Thus, as p → or as N - M and M→ →,