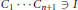Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Hollowood, Timothy J.
Miramontes, J. Luis
and
Schmidtt, David M.
2013.
The structure of non-abelian kinks.
Journal of High Energy Physics,
Vol. 2013,
Issue. 10,
Kramer, Linus
2016.
On small abstract quotients of Lie groups and locally compact groups.
Journal of Geometry,
Vol. 107,
Issue. 2,
p.
403.
Radjavi, Heydar
and
Sourour, Ahmed Ramzi
2021.
Groups and semigroups generated by a single unitary orbit.
Semigroup Forum,
Vol. 102,
Issue. 1,
p.
274.