Hostname: page-component-78c5997874-94fs2 Total loading time: 0 Render date: 2024-11-13T06:54:20.466Z Has data issue: false hasContentIssue false
  • English
  • Français

The Right Regular Representation of a Compact Right Topological Group

Published online by Cambridge University Press:  20 November 2018

Alan Moran
Alan Moran*
Affiliation:
School of Mathematics and Statistics Section Pure Mathematics Sheffield University Sheffield S3 7RH England
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We show that for certain compact right topological groups, 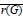$\overline{r(G)}$, the strong operator topology closure of the image of the right regular representation of 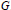$G$ in 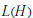$L(H)$, where $H\,=\,{{L}^{2}}\,(G)$, is a compact topological group and introduce a class of representations, $R$ , which effectively transfers the representation theory of 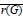$\overline{r(G)}$ over to 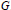$G$. Amongst the groups for which this holds is the class of equicontinuous groups which have been studied by Ruppert in [10].We use familiar examples to illustrate these features of the theory and to provide a counter-example. Finally we remark that every equicontinuous group which is at the same time a Borel group is in fact a topological group.

Keywords

22D99
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 41 , Issue 4 , 01 December 1998 , pp. 463 - 472
Copyright
Copyright © Canadian Mathematical Society 1998

References

1. Berglund, J. F., Junghenn, H. D. and Milnes, P., Analysis on Semigroups: Function Spaces, Compactifications and Representations. Wiley, New York, 1989.Google Scholar
2. Ellis, R., Locally compact transformation groups. Duke Math. J. 24 (1957), 119–126.Google Scholar
3. Halmos, P., Measure Theory. Springer-Verlag, New York, 1974.Google Scholar
4. Milnes, P., Representations of Compact Right Topological Groups. Canad. Math. Bull. (3) 36 (1993), 314–323.Google Scholar
5. Milnes, P., Distal Compact Right Topological Groups. Acta Math. Hungar. (2) 65 (1994), 149–163.Google Scholar
6. Milnes, P. and Pym, J. S., Haar Measure for Compact Right Topological Groups. Proc. Amer.Math. Soc. 114 (1992), 387–393.Google Scholar
7. Milnes, P. and Pym, J. S., Homomorphisms of Minimal and Distal Flows. J. Nigerian Math. Soc. 11 (1992), 63–80.Google Scholar
8. Moran, A., Minimal Normal Systems in Compact Right Topological Groups. Math. Proc. Cambridge Philos. Soc., to appear.Google Scholar
9. Namioka, I., Right Topological Groups, Distal Flows, and a Fixed Point Theorem.Math. Systems Theory 6 (1972), 193–209.Google Scholar
10. Ruppert, W., Über kompakte rechtstopologische Gruppen mit gleichgradig stetigen Linkstranslationen. Österreich. Akad. Wiss. Math.-Natur. Kl. Sitzungsber. II, 184 (1975), 159–169.Google Scholar