Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Sukochev, F. A.
2000.
Operator Estimates for Fredholm Modules.
Canadian Journal of Mathematics,
Vol. 52,
Issue. 4,
p.
849.
Carey, A.L.
Phillips, J.
and
Sukochev, F.A.
2000.
On Unbounded p-Summable Fredholm Modules.
Advances in Mathematics,
Vol. 151,
Issue. 2,
p.
140.
Booss-Bavnbek, Bernhelm
Lesch, Matthias
and
Phillips, John
2002.
Spectral flow of paths of self-adjoint Fredholm operators.
Nuclear Physics B - Proceedings Supplements,
Vol. 104,
Issue. 1-3,
p.
177.
FURUTANI, Kenro
and
OTSUKI, Nobukazu
2002.
Maslov index in the infinite dimension and a splitting formula for a spectral flow.
Japanese journal of mathematics. New series,
Vol. 28,
Issue. 2,
p.
215.
Booss-Bavnbek, B.
Furutani, K.
and
Wojciechowski, K. P.
2003.
Jean Leray ’99 Conference Proceedings.
p.
321.
Carey, Alan
Phillips, John
and
Sukochev, Fyodor
2003.
Spectral flow and Dixmier traces.
Advances in Mathematics,
Vol. 173,
Issue. 1,
p.
68.
Savin, Anton
and
Sternin, Boris
2004.
Aspects of Boundary Problems in Analysis and Geometry.
p.
170.
Furutani, Kenro
2004.
Fredholm–Lagrangian–Grassmannian and the Maslov index.
Journal of Geometry and Physics,
Vol. 51,
Issue. 3,
p.
269.
Giambò, Roberto
Piccione, Paolo
and
Portaluri, Alessandro
2004.
Computation of the Maslov index and the spectral flow via partial signatures.
Comptes Rendus. Mathématique,
Vol. 338,
Issue. 5,
p.
397.
Bleecker, David
and
Booss—Bavnbek, Bernhelm
2004.
Aspects of Boundary Problems in Analysis and Geometry.
p.
1.
Kirk, Paul
and
Lesch, Matthias
2004.
The [eta]-invariant, Maslov index, and spectral flow for Dirac-type operators on manifolds with boundary.
Forum Mathematicum,
Vol. 16,
Issue. 4,
Piccione, Paolo
and
Portaluri, Alessandro
2005.
A bifurcation result for semi-Riemannian trajectories of the Lorentz force equation.
Journal of Differential Equations,
Vol. 210,
Issue. 2,
p.
233.
Eidam, J.C.C.
and
Piccione, P.
2006.
The essential Lagrangian–Grassmannian and the homotopy type of the Fredholm Lagrangian–Grassmannian.
Topology and its Applications,
Vol. 153,
Issue. 15,
p.
2782.
Кери, Ален Л.
Carey, Alan L.
Сукочев, Федор Анатольевич
and
Sukochev, Fedor Anatol'evich
2006.
Следы Диксмье и некоторые приложения в некоммутативной геометрии.
Успехи математических наук,
Vol. 61,
Issue. 6,
p.
45.
Carey, Alan L.
Phillips, John
Rennie, Adam
and
Sukochev, Fyodor A.
2006.
The local index formula in semifinite Von Neumann algebras I: Spectral flow.
Advances in Mathematics,
Vol. 202,
Issue. 2,
p.
451.
Savin, Anton
and
Sternin, Boris
2006.
C*-algebras and Elliptic Theory.
p.
247.
Azamov, N. A.
Carey, A. L.
and
Sukochev, F. A.
2007.
The Spectral Shift Function and Spectral Flow.
Communications in Mathematical Physics,
Vol. 276,
Issue. 1,
p.
51.
Javaloyes, Miguel Angel
and
Piccione, Paolo
2008.
Spectral flow and iteration of closed semi-Riemannian geodesics.
Calculus of Variations and Partial Differential Equations,
Vol. 33,
Issue. 4,
p.
439.
Wahl, Charlotte
2008.
C*-algebras and Elliptic Theory II.
p.
297.
Javaloyes, Miguel Angel
de Lima, Levi Lopes
and
Piccione, Paolo
2008.
Iteration of closed geodesics in stationary Lorentzian manifolds.
Mathematische Zeitschrift,
Vol. 260,
Issue. 2,
p.
277.
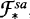 , of self-adjoint Fredholm operators on a separable Hilbert space. In particular, if {Bt } is a path of such operators, we can associate to {Bt } an integer, sf({Bt }), called the spectral flow of the path. This notion, due to M. Atiyah and G. Lusztig, assigns to the path {Bt } the net number of eigenvalues (counted with multiplicities) which pass through 0 in the positive direction. There are difficulties in making this precise — the usual argument involves looking at the graph of the spectrum of the family (after a suitable perturbation) and then counting intersection numbers with y = 0.
, of self-adjoint Fredholm operators on a separable Hilbert space. In particular, if {Bt } is a path of such operators, we can associate to {Bt } an integer, sf({Bt }), called the spectral flow of the path. This notion, due to M. Atiyah and G. Lusztig, assigns to the path {Bt } the net number of eigenvalues (counted with multiplicities) which pass through 0 in the positive direction. There are difficulties in making this precise — the usual argument involves looking at the graph of the spectrum of the family (after a suitable perturbation) and then counting intersection numbers with y = 0.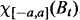 . The spectral flow is then defined as the dimension of the nonnegative eigenspace at the end of this path minus the dimension of the nonnegative eigenspace at the beginning. This leads to an easy proof that spectral flow is a well-defined homomorphism from the homotopy groupoid of
. The spectral flow is then defined as the dimension of the nonnegative eigenspace at the end of this path minus the dimension of the nonnegative eigenspace at the beginning. This leads to an easy proof that spectral flow is a well-defined homomorphism from the homotopy groupoid of 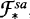 onto Z. For the sake of completeness we also outline the seldom-mentioned proof that the restriction of spectral flow to
onto Z. For the sake of completeness we also outline the seldom-mentioned proof that the restriction of spectral flow to 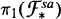 is an isomorphism onto Z.
is an isomorphism onto Z.
