Hostname: page-component-cd9895bd7-mkpzs Total loading time: 0 Render date: 2024-12-26T17:47:27.647Z Has data issue: false hasContentIssue false
  • English
  • Français

Simple Decompositions of the Exceptional Jordan Algebra

Published online by Cambridge University Press:  20 November 2018

M. V. Tvalavadze
M. V. Tvalavadze*
Affiliation:
Department of Mathematics and Statistics, Memorial University of Newfoundland, St. John’s, NL A1C 5S7. e-mail: marina@math.mun.ca
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

This paper presents some results on the simple exceptional Jordan algebra over an algebraically closed field $\Phi$ of characteristic not 2. Namely an example of simple decomposition of $H\left( {{O}_{3}} \right)$ into the sumof two subalgebras of the type 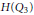$H\left( {{Q}_{3}} \right)$ is produced, and it is shown that this decomposition is the only one possible in terms of simple subalgebras.

Keywords

17C40
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 50 , Issue 3 , 01 September 2007 , pp. 469 - 473
Copyright
Copyright © Canadian Mathematical Society 2007

References

[1] van der Blij, F. and Springer, T. A., The arithmetics of octaves and of the group G 2 . Nederl. Akad. Wetensch. Proc. Ser. A 21(1959), 406–418.Google Scholar
[2] Jacobson, N., General representation theory of Jordan algebra. Trans. Amer. Math. Soc. 70(1951), 509–530.Google Scholar
[3] McCrimmon, K., Malcev's theorem for Jordan algebras.. Comm. Algebra 5(1977), no. 9, 937–967.Google Scholar
[4] Racine, M. L., On maximal subalgebras. J. Algebra 30(1974), 155–180.Google Scholar
[5] Racine, M. L., Maximal subalgebras of exceptional Jordan algebra. J. Algebra 46(1977), no. 1, 12–21.Google Scholar