No CrossRef data available.
Published online by Cambridge University Press: 20 November 2018
Given a complex semisimple Lie algebra  $\mathfrak{g}=\mathfrak{k}+i\mathfrak{k}$ (
$\mathfrak{g}=\mathfrak{k}+i\mathfrak{k}$ ( $\mathfrak{t}$ is a compact real form of
$\mathfrak{t}$ is a compact real form of  $\mathfrak{g}$), let
$\mathfrak{g}$), let  $\text{ }\pi \text{ }\text{:}\mathfrak{g}\to \mathfrak{h}$ be the orthogonal projection (with respect to the Killing form) onto the Cartan subalgebra
$\text{ }\pi \text{ }\text{:}\mathfrak{g}\to \mathfrak{h}$ be the orthogonal projection (with respect to the Killing form) onto the Cartan subalgebra 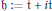 $\mathfrak{h}:=\mathfrak{t}\text{+}i\mathfrak{t}$, where
$\mathfrak{h}:=\mathfrak{t}\text{+}i\mathfrak{t}$, where  $\mathfrak{t}$ is a maximal abelian subalgebra of
$\mathfrak{t}$ is a maximal abelian subalgebra of  $\mathfrak{k}$. Given
$\mathfrak{k}$. Given  $x\,\in \,\mathfrak{g}$, we consider
$x\,\in \,\mathfrak{g}$, we consider 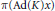 $\text{ }\!\!\pi\!\!\text{ (Ad(}K\text{)}x)$, where
$\text{ }\!\!\pi\!\!\text{ (Ad(}K\text{)}x)$, where 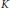 $K$ is the analytic subgroup
$K$ is the analytic subgroup  $G$ corresponding to
$G$ corresponding to  $\mathfrak{k}$, and show that it is star-shaped. The result extends a result of Tsing. We also consider the generalized numerical range
$\mathfrak{k}$, and show that it is star-shaped. The result extends a result of Tsing. We also consider the generalized numerical range 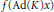 $f(\text{Ad(}K\text{)}x)$, where
$f(\text{Ad(}K\text{)}x)$, where 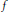 $f$ is a linear functional on
$f$ is a linear functional on  $\mathfrak{g}$. We establish the star-shapedness of
$\mathfrak{g}$. We establish the star-shapedness of 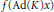 $f(\text{Ad(}K\text{)}x)$ for simple Lie algebras of type
$f(\text{Ad(}K\text{)}x)$ for simple Lie algebras of type  $B$.
$B$.