Article contents
Uniqueness Implies Existence and Uniqueness Conditions for a Class of (k + j)-Point Boundary Value Problems for n-th Order Differential Equations
Published online by Cambridge University Press: 20 November 2018
Abstract
For the  $n$-th order nonlinear differential equation,
$n$-th order nonlinear differential equation, 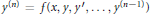 ${{y}^{(n)}}\,=\,f(x,\,y,\,y\prime ,\ldots ,\,{{y}^{(n-1)}})$, we consider uniqueness implies uniqueness and existence results for solutions satisfying certain
${{y}^{(n)}}\,=\,f(x,\,y,\,y\prime ,\ldots ,\,{{y}^{(n-1)}})$, we consider uniqueness implies uniqueness and existence results for solutions satisfying certain 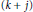 $(k\,+\,j)$-point boundary conditions for
$(k\,+\,j)$-point boundary conditions for 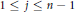 $1\,\le \,j\,\le \,n\,-\,1$ and
$1\,\le \,j\,\le \,n\,-\,1$ and 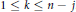 $1\,\le \,k\,\le \,n\,-\,j$. We define
$1\,\le \,k\,\le \,n\,-\,j$. We define 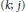 $(k;\,j)$-point unique solvability in analogy to
$(k;\,j)$-point unique solvability in analogy to  $k$-point disconjugacy and we show that
$k$-point disconjugacy and we show that 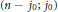 $(n\,-\,{{j}_{0}};\,{{j}_{0}})$-point unique solvability implies
$(n\,-\,{{j}_{0}};\,{{j}_{0}})$-point unique solvability implies 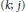 $(k;\,j)$-point unique solvability for
$(k;\,j)$-point unique solvability for  $1\,\le \,j\,\le \,{{j}_{0}}$, and
$1\,\le \,j\,\le \,{{j}_{0}}$, and 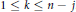 $1\,\le \,k\,\le \,n\,-\,j$. This result is analogous to
$1\,\le \,k\,\le \,n\,-\,j$. This result is analogous to  $n$-point disconjugacy implies
$n$-point disconjugacy implies  $k$-point disconjugacy for
$k$-point disconjugacy for 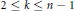 $2\,\le \,k\,\le \,n\,-\,1$.
$2\,\le \,k\,\le \,n\,-\,1$.
Keywords
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 2012
References
- 4
- Cited by


