The virtually generating graph of a profinite group

Abstract

We consider the grap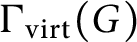h $\Gamma _{\text {virt}}(G)$ whose vertices are the elements of a finitely generated profinite group G and where two vertices x and y are adjacent if and only if they topologically generate an open subgroup of G. We investigate the connectivity of the graph 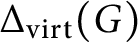$\Delta _{\text {virt}}(G)$ obtained from $\Gamma _{\text {virt}}(G)$ by removing its isolated vertices. In particular, we prove that for every positive integer t, there exists a finitely generated prosoluble group G with the property that 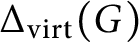$\Delta _{\operatorname {\mathrm {virt}}}(G)$ has precisely t connected components. Moreover, we study the graph 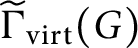$\widetilde \Gamma _{\operatorname {\mathrm {virt}}}(G)$ , whose vertices are again the elements of G and where two vertices are adjacent if and only if there exists a minimal generating set of G containing them. 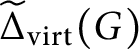In this case, we prove that the subgraph $\widetilde \Delta _{\operatorname {\mathrm {virt}}}(G)$ obtained removing the isolated vertices is connected and has diameter at most 3.