No CrossRef data available.
Article contents
Size Ramsey Number of Bounded Degree Graphs for Games
Published online by Cambridge University Press: 11 June 2013
Abstract
We study Maker/Breaker games on the edges of sparse graphs. Maker and Breaker take turns at claiming previously unclaimed edges of a given graph H. Maker aims to occupy a given target graph G and Breaker tries to prevent Maker from achieving his goal. We show that for every d there is a constant c = c(d) with the property that for every graph G on n vertices of maximum degree d there is a graph H on at most cn edges such that Maker has a strategy to occupy a copy of G in the game on H.
This is a result about a game-theoretic variant of the size Ramsey number. For a given graph G,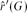 
$\hat{r}'(G)$ is defined as the smallest number M for which there exists a graph H with M edges such that Maker has a strategy to occupy a copy of G in the game on H. In this language, our result yields that for every connected graph G of constant maximum degree, 
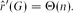$\hat{r}'(G) = \Theta(n)$.
Moreover, we can also use our method to settle the corresponding extremal number for universal graphs: for a constant d and for the class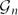 
${\cal G}_{n}$ of n-vertex graphs of maximum degree d, 
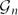$s({\cal G}_{n})$ denotes the minimum number such that there exists a graph H with M edges where, for everyG ∈ ${\cal G}_{n}$, Maker has a strategy to build a copy of G in the game on H. We obtain that 
$s({\cal G}_{n}) = \Theta(n^{2 - \frac{2}{d}})$.
Keywords
- Type
- Paper
- Information
- Copyright
- Copyright © Cambridge University Press 2013
References
[1]Alon, N. and Asodi, V. (2002) Sparse universal graphs. J. Comput. Appl. Math. 142 1–11.Google Scholar
[2]Alon, N. and Capalbo, M. (2007) Sparse universal graphs for bounded degree graphs. Random Struct. Alg. 31 123–133.Google Scholar
[3]Alon, N. and Capalbo, M. (2008) Optimal universal graphs with deterministic embedding. In Proc. 19th Annual ACM–SIAM Symposium on Discrete Algorithms (SODA), pp. 373–378.Google Scholar
[4]Alon, N., Capalbo, M., Kohayakawa, Y., Rödl, V., Ruciński, A. and Szemerédi, E. (2000) Universality and tolerance. In Proc. 41st IEEE Symposium on Foundations of Computer Science (FOCS), pp. 14–21.Google Scholar
[5]Alon, N., Capalbo, M., Kohayakawa, Y., Rödl, V., Ruciński, A. and Szemerédi, E. (2001) Near-optimum universal graphs for graphs with bounded degrees. In RANDOM–APPROX, pp. 170–180.Google Scholar
[6]Alon, N., Krivelevich, M., Spencer, J. and Szabó, T. (2005) Discrepancy games. Electron. J. Combin. 12 R51.CrossRefGoogle Scholar
[7]Babai, L., Chung, F. R. K., Erdős, P., Graham, R. L. and Spencer, J. (1982) On graphs which contain all sparse graphs. Ann. Discrete Math. 12 21–26.Google Scholar
[9]Beck, J. (1983) On size Ramsey number of paths, trees and cycles I. J. Graph Theory 7 115–130.Google Scholar
[10]Beck, J. (1994) Deterministic graph games and a probabilistic intuition. Combin. Probab. Comput. 3 13–26.Google Scholar
[11]Bhatt, S. N., Chung, F., Leighton, F. T. and Rosenberg, A. (1989) Universal graphs for bounded-degree trees and planar graphs. SIAM J. Discrete Math. 2 145–155.Google Scholar
[12]Bhatt, S. N. and Leiserson, C. E. (1984) How to assemble tree machines. In Advances in Computing Research 2, (Preparata, F. P., ed.) JAI Press, Greenwich, CT, pp. 95–114.Google Scholar
[13]Capalbo, M. (1999) A small universal graph for bounded-degree planar graphs. In Proc. 10th Annual ACM–SIAM Symposium on Discrete Algorithms (SODA), pp. 150–154.Google Scholar
[14]Capalbo, M. and Kosaraju, S. R. (1999) Small universal graphs. In Proc. 31st Annual ACM Symposium on Theory of Computing (STOC), pp. 741–749.Google Scholar
[15]Chung, F. R. K. and Graham, R. L. (1978) On graphs which contain all small trees. J. Combin. Theory Ser. B 24 14–23.Google Scholar
[16]Chung, F. R. K. and Graham, R. L. (1979) On universal graphs. Ann. New York Acad. Sci. 319 136–140.Google Scholar
[17]Chung, F. R. K. and Graham, R. L. (1983) On universal graphs for spanning trees. Proc. London Math. Soc. 27 203–211.Google Scholar
[18]Chung, F. R. K., Rosenberg, A. L. and Snyder, L. (1983) Perfect storage representations for families of data structures. SIAM J. Alg. Discrete Methods 4 548–565.Google Scholar
[19]Chung, F. and Graham, R. (1998) Erdős on Graphs: His Legacy of Unsolved Problems, A. K. Peters.CrossRefGoogle Scholar
[20]Chvátal, V., Rödl, V., Szemerédi, E. and Trotter, W. T. (1983) The Ramsey number of a graph with bounded maximum degree. J. Combin. Theory Ser. B 34 239–243.Google Scholar
[21]Erdős, P., Faudree, R. J., Rousseau, C. C. and Schelp, R. H. (1978) The size Ramsey number. Period. Math. Hungar. 9 145–161.Google Scholar
[22]Feldheim, O. N. and Krivelevich, M. (2008) Winning fast in sparse graph construction games. Combin. Probab. Comput. 17 781–791.Google Scholar
[23]Fox, J. and Sudakov, B. (2009) Two remarks on the Burr–Erdőos conjecture. Europ. J. Combin. 30 1630–1645.Google Scholar
[24]Friedman, J. and Pippenger, N. (1987) Expanding graphs contain all small trees. Combinatorica 7 71–76.Google Scholar
[25]Haxell, P. E., Kohayakawa, Y. and Łuczak, T. (1995) The induced size-Ramsey number of cycles. Combin. Probab. Comput. 4 217–239.Google Scholar
[26]Kohayakawa, Y., Rödl, V., Schacht, M. and Szemerédi, E. (2011) Sparse partition universal graphs for graphs of bounded degree. Adv. Math. 226 5041–5065.Google Scholar
[28]Rödl, V. and Szemerédi, E. (2000) On size Ramsey numbers of graphs with bounded degree. Combinatorica 20 257–262.Google Scholar
[29]Székely, L. A. (1984) On two concepts of discrepancy in a class of combinatorial games. In Infinite and Finite Sets, Vol. 37 of Colloquia Mathematica Societatis Janos Bolyai, pp. 679–683.Google Scholar