Article contents
A Pair of Forbidden Subgraphs and 2-Factors
Published online by Cambridge University Press: 02 February 2012
Abstract
In this paper, we consider pairs of forbidden subgraphs that imply the existence of a 2-factor in a graph. For d ≥ 2, let  d be the set of connected graphs of minimum degree at least d. Let F1 and F2 be connected graphs and let
d be the set of connected graphs of minimum degree at least d. Let F1 and F2 be connected graphs and let 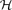 be a set of connected graphs. Then {F1, F2} is said to be a forbidden pair for
be a set of connected graphs. Then {F1, F2} is said to be a forbidden pair for 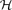 if every {F1, F2}-free graph in
if every {F1, F2}-free graph in 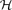 of sufficiently large order has a 2-factor. Faudree, Faudree and Ryjáček have characterized all the forbidden pairs for the set of 2-connected graphs. We first characterize the forbidden pairs for
of sufficiently large order has a 2-factor. Faudree, Faudree and Ryjáček have characterized all the forbidden pairs for the set of 2-connected graphs. We first characterize the forbidden pairs for  2, which is a larger set than the set of 2-connected graphs, and observe a sharp difference between the characterized pairs and those obtained by Faudree, Faudree and Ryjáček. We then consider the forbidden pairs for connected graphs of large minimum degree. We prove that if {F1, F2} is a forbidden pair for
2, which is a larger set than the set of 2-connected graphs, and observe a sharp difference between the characterized pairs and those obtained by Faudree, Faudree and Ryjáček. We then consider the forbidden pairs for connected graphs of large minimum degree. We prove that if {F1, F2} is a forbidden pair for  d, then either F1 or F2 is a star of order at most d + 2. Ota and Tokuda have proved that every
d, then either F1 or F2 is a star of order at most d + 2. Ota and Tokuda have proved that every 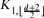 -free graph of minimum degree at least d has a 2-factor. These results imply that for k ≥ d + 2, no connected graphs F except for stars of order at most d + 2 make {K1,k, F} a forbidden pair for
-free graph of minimum degree at least d has a 2-factor. These results imply that for k ≥ d + 2, no connected graphs F except for stars of order at most d + 2 make {K1,k, F} a forbidden pair for  d, while for
d, while for  every connected graph F makes {K1,k, F} a forbidden pair for
every connected graph F makes {K1,k, F} a forbidden pair for  d. We consider the remaining range of
d. We consider the remaining range of 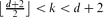 , and prove that only a finite number of connected graphs F make {K1,k, F} a forbidden pair for
, and prove that only a finite number of connected graphs F make {K1,k, F} a forbidden pair for  d.
d.
Information
- Type
- Paper
- Information
- Combinatorics, Probability and Computing , Volume 21 , Issue 1-2: Honouring the Memory of Richard H. Schelp , March 2012 , pp. 149 - 158
- Copyright
- Copyright © Cambridge University Press 2012
References
- 5
- Cited by

