Article contents
Big Galois representations and 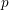 $p$-adic
$p$-adic 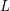 $L$-functions
$L$-functions
Published online by Cambridge University Press: 13 November 2014
Abstract
Let 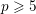 $p\geqslant 5$ be a prime. If an irreducible component of the spectrum of the ‘big’ ordinary Hecke algebra does not have complex multiplication, under mild assumptions, we prove that the image of its Galois representation contains, up to finite error, a principal congruence subgroup
$p\geqslant 5$ be a prime. If an irreducible component of the spectrum of the ‘big’ ordinary Hecke algebra does not have complex multiplication, under mild assumptions, we prove that the image of its Galois representation contains, up to finite error, a principal congruence subgroup 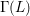 ${\rm\Gamma}(L)$ of
${\rm\Gamma}(L)$ of 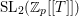 $\text{SL}_{2}(\mathbb{Z}_{p}[[T]])$ for a principal ideal
$\text{SL}_{2}(\mathbb{Z}_{p}[[T]])$ for a principal ideal 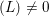 $(L)\neq 0$ of
$(L)\neq 0$ of 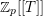 $\mathbb{Z}_{p}[[T]]$ for the canonical ‘weight’ variable
$\mathbb{Z}_{p}[[T]]$ for the canonical ‘weight’ variable 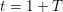 $t=1+T$. If
$t=1+T$. If 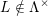 $L\notin {\rm\Lambda}^{\times }$, the power series
$L\notin {\rm\Lambda}^{\times }$, the power series 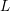 $L$ is proven to be a factor of the Kubota–Leopoldt
$L$ is proven to be a factor of the Kubota–Leopoldt 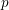 $p$-adic
$p$-adic 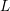 $L$-function or of the square of the anticyclotomic Katz
$L$-function or of the square of the anticyclotomic Katz  $p$-adic
$p$-adic 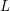 $L$-function or a power of
$L$-function or a power of 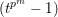 $(t^{p^{m}}-1)$.
$(t^{p^{m}}-1)$.
Information
- Type
- Research Article
- Information
- Copyright
- © The Author 2014
References
- 5
- Cited by

