Article contents
Generalized Euler characteristic in power-bounded T-convex valued fields
Published online by Cambridge University Press: 14 September 2017
Abstract
We lay the groundwork in this first installment of a series of papers aimed at developing a theory of Hrushovski–Kazhdan style motivic integration for certain types of nonarchimedean  $o$ -minimal fields, namely power-bounded
$o$ -minimal fields, namely power-bounded 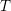 $T$ -convex valued fields, and closely related structures. The main result of the present paper is a canonical homomorphism between the Grothendieck semirings of certain categories of definable sets that are associated with the
$T$ -convex valued fields, and closely related structures. The main result of the present paper is a canonical homomorphism between the Grothendieck semirings of certain categories of definable sets that are associated with the 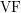 $\text{VF}$ -sort and the
$\text{VF}$ -sort and the  $\text{RV}$ -sort of the language
$\text{RV}$ -sort of the language 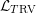 ${\mathcal{L}}_{T\text{RV}}$ . Many aspects of this homomorphism can be described explicitly. Since these categories do not carry volume forms, the formal groupification of the said homomorphism is understood as a universal additive invariant or a generalized Euler characteristic. It admits not just one, but two specializations to
${\mathcal{L}}_{T\text{RV}}$ . Many aspects of this homomorphism can be described explicitly. Since these categories do not carry volume forms, the formal groupification of the said homomorphism is understood as a universal additive invariant or a generalized Euler characteristic. It admits not just one, but two specializations to 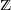 $\unicode[STIX]{x2124}$ . The overall structure of the construction is modeled on that of the original Hrushovski–Kazhdan construction.
$\unicode[STIX]{x2124}$ . The overall structure of the construction is modeled on that of the original Hrushovski–Kazhdan construction.
Information
- Type
- Research Article
- Information
- Copyright
- © The Author 2017
References
- 5
- Cited by

