Article contents
Products of twists, geodesic lengths and Thurston shears
Published online by Cambridge University Press: 09 October 2014
Abstract
Thurston introduced shear deformations (cataclysms) on geodesic laminations–deformations including left and right displacements along geodesics. For hyperbolic surfaces with cusps, we consider shear deformations on disjoint unions of ideal geodesics. The length of a balanced weighted sum of ideal geodesics is defined and the Weil–Petersson (WP) duality of shears and the defined length is established. The Poisson bracket of a pair of balanced weight systems on a set of disjoint ideal geodesics is given in terms of an elementary 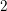 $2$-form. The symplectic geometry of balanced weight systems on ideal geodesics is developed. Equality of the Fock shear coordinate algebra and the WP Poisson algebra is established. The formula for the WP Riemannian pairing of shears is also presented.
$2$-form. The symplectic geometry of balanced weight systems on ideal geodesics is developed. Equality of the Fock shear coordinate algebra and the WP Poisson algebra is established. The formula for the WP Riemannian pairing of shears is also presented.
Keywords
Information
- Type
- Research Article
- Information
- Copyright
- © The Author 2014
References
- 1
- Cited by

