Hostname: page-component-7dd5485656-npwhs Total loading time: 0 Render date: 2025-10-30T18:06:35.316Z Has data issue: false hasContentIssue false
  • English
  • Français

Selmer groups and class groups

Part of: Arithmetic algebraic geometry Algebraic number theory: global fields

Published online by Cambridge University Press:  11 November 2014

Kęstutis Česnavičius
Kęstutis Česnavičius*
Affiliation:
Department of Mathematics, Massachusetts Institute of Technology, Cambridge, MA 02139, USA email kestutis@math.mit.edu
Get access Rights & Permissions [Opens in a new window]

Abstract

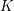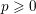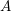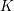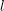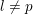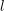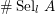Let $A$ be an abelian variety over a global field $K$ of characteristic $p\geqslant 0$. If $A$ has nontrivial (respectively full) $K$-rational $l$-torsion for a prime $l\neq p$, we exploit the fppf cohomological interpretation of the $l$-Selmer group $\text{Sel}_{l}\,A$ to bound $\#\text{Sel}_{l}\,A$ from below (respectively above) in terms of the cardinality of the 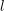$l$-torsion subgroup of the ideal class group of $K$. Applied over families of finite extensions of 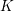$K$, the bounds relate the growth of Selmer groups and class groups. For function fields, this technique proves the unboundedness of $l$-ranks of class groups of quadratic extensions of every 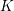$K$ containing a fixed finite field $\mathbb{F}_{p^{n}}$ (depending on $l$). For number fields, it suggests a new approach to the Iwasawa 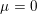${\it\mu}=0$ conjecture through inequalities, valid when $A(K)[l]\neq 0$, between Iwasawa invariants governing the growth of Selmer groups and class groups in a 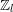$\mathbb{Z}_{l}$-extension.

Keywords

Selmer groupclass groupfppf cohomologyIwasawa theory

MSC classification

Primary: 11G10: Abelian varieties of dimension $> 1$
Secondary: 11R23: Iwasawa theory 11R29: Class numbers, class groups, discriminants 11R58: Arithmetic theory of algebraic function fields

Information

Type
Research Article
Information
Compositio Mathematica , Volume 151 , Issue 3 , March 2015 , pp. 416 - 434
Copyright
© The Author 2014 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

References

Artin, E. and Whaples, G., Axiomatic characterization of fields by the product formula for valuations, Bull. Amer. Math. Soc. N.S. 51 (1945), 469–492; MR 0013145 (7,111f).Google Scholar
Bhargava, M., Kane, D. M., Lenstra, H. W. Jr, Poonen, B. and Rains, E., Modeling the distribution of ranks, Selmer groups, and Shafarevich–Tate groups of elliptic curves, Preprint (2013), arXiv:1304.3971.Google Scholar
Bölling, R., Die Ordnung der Schafarewitsch-Tate-Gruppe kann beliebig groß werden, Math. Nachr. 67 (1975), 157–179 (German); MR 0384812 (52 #5684).CrossRefGoogle Scholar
Bosch, S., Lütkebohmert, W. and Raynaud, M., Néron models, Ergebnisse der Mathematik und ihrer Grenzgebiete. 3 Folge [Results in Mathematics and Related Areas. 3rd Series. A Series of Modern Surveys in Mathematics], vol. 21 (Springer, Berlin, 1990); MR 1045822 (91i:14034).Google Scholar
Česnavičius, K., Selmer groups as flat cohomology groups, Preprint (2014), arXiv:1301.4724.Google Scholar
Conrad, B., Gabber, O. and Prasad, G., Pseudo-reductive groups, New Mathematical Monographs, vol. 17 (Cambridge University Press, Cambridge, 2010); MR 2723571 (2011k:20093).Google Scholar
Cohen, H. and Lenstra, H. W. Jr, Heuristics on class groups, in Number theory (New York, 1982), Lecture Notes in Mathematics, vol. 1052 (Springer, Berlin, 1984), 26–36; doi:10.1007/BFb0071539; MR 750661.Google Scholar
Clark, P. L., The period-index problem in WC-groups II: abelian varieties, Preprint (2004), arXiv:math/0406135.Google Scholar
Creutz, B., Potential Sha for abelian varieties, J. Number Theory 131 (2011), 2162–2174; doi:10.1016/j.jnt.2011.05.013; MR 2825120 (2012h:11089).CrossRefGoogle Scholar
Clark, P. L. and Sharif, S., Period, index and potential. III, Algebra Number Theory 4 (2010), 151–174; doi:10.2140/ant.2010.4.151; MR 2592017 (2011b:11075).Google Scholar
Grothendieck, A. and Dieudonné, J., Éléments de géométrie algébrique. I. Le langage des schémas, Inst. Hautes Études Sci. Publ. Math. 4 (1960), 228; MR 0217083 (36 #177a).Google Scholar
Grothendieck, A. and Dieudonné, J., Éléments de géométrie algébrique. IV. Étude locale des schémas et des morphismes de schémas. II, Publ. Math. Inst. Hautes Études Sci. 32 (1965), 231 (French); MR 0199181 (33 #7330).Google Scholar
Grothendieck, A. and Dieudonné, J., Éléments de géométrie algébrique. IV. Étude locale des schémas et des morphismes de schémas IV, Publ. Math. Inst. Hautes Études Sci. 24 (1967), 361 (French); MR 0238860 (39 #220).Google Scholar
Ferrero, B. and Washington, L. C., The Iwasawa invariant 𝜇p vanishes for abelian number fields, Ann. of Math. (2) 109 (1979), 377–395; doi:10.2307/1971116; MR 528968 (81a:12005).Google Scholar
Gross, B. H. and Harris, J., Real algebraic curves, Ann. Sci. Éc. Norm. Supér. (4) 14 (1981), 157–182; MR 631748 (83a:14028).Google Scholar
Greenberg, R., Iwasawa theory for elliptic curves, in Arithmetic theory of elliptic curves (Cetraro, 1997), Lecture Notes in Mathematics, vol. 1716 (Springer, Berlin, 1999), 51–144; doi:10.1007/BFb0093453; MR 1754686 (2002a:11056).Google Scholar
Greenberg, R., Galois theory for the Selmer group of an abelian variety, Compositio Math. 136 (2003), 255–297; doi:10.1023/A:1023251032273; MR 1977007 (2004c:11097).Google Scholar
Grothendieck, A., Le groupe de Brauer. III. Exemples et compléments, in Dix Exposés sur la Cohomologie des Schémas (North-Holland, Amsterdam, 1968), 88–188 (French); MR 0244271 (39 #5586c).Google Scholar
Iwasawa, K., On some infinite Abelian extensions of algebraic number fields, in Actes du congrès international des mathématiciens (Nice 1970) (Gauthier-Villars, Paris, 1971), 391–394; MR 0422205 (54 #10197).Google Scholar
Iwasawa, K., On the 𝜇-invariants of Z l-extensions, in Number theory, algebraic geometry and commutative algebra, in Honor of Yasuo Akizuki (Kinokuniya, Tokyo, 1973), 1–11; MR 0357371 (50 #9839).Google Scholar
Iwasawa, K., Local class field theory, Oxford Mathematical Monographs (Oxford Science Publications, The Clarendon Press, Oxford University Press, New York, 1986); MR 863740 (88b:11080).Google Scholar
Kato, K., p-adic Hodge theory and values of zeta functions of modular forms, in Cohomologies p-adiques et applications arithmétiques. III, Astérisque, 295, (2004), ix, 117–290 (English, with English and French summaries); MR 2104361 (2006b:11051).Google Scholar
Klagsbrun, Z., Selmer Ranks of Quadratic Twists of Elliptic Curves, PhD Thesis, University of California, Irvine, ProQuest LLC, Ann Arbor, MI (2011) 53; MR 2890124.Google Scholar
Madan, M. L., Class groups of global fields, J. Reine Angew. Math. 252 (1972), 171–177; MR 0296049 (45 #5110).Google Scholar
Mattuck, A., Abelian varieties over p-adic ground fields, Ann. of Math. (2) 62 (1955), 92–119; MR 0071116 (17,87f).Google Scholar
Mazur, B., Rational points of abelian varieties with values in towers of number fields, Invent. Math. 18 (1972), 183–266; MR 0444670 (56 #3020).Google Scholar
Milne, J. S., Arithmetic duality theorems, second edition (BookSurge, LLC, Charleston, SC, 2006); MR 2261462 (2007e:14029).Google Scholar
Ochiai, T. and Trihan, F., On the Selmer groups of abelian varieties over function fields of characteristic p > 0, Math. Proc. Cambridge Philos. Soc. 146 (2009), 23–43; doi:10.1017/S0305004108001801; MR 2461865 (2009m:11182).CrossRefGoogle Scholar
Quer, J., Corps quadratiques de 3-rang 6 et courbes elliptiques de rang 12, C. R. Acad. Sci. Paris Sér. I Math. 305 (1987), 215–218 (French, with English summary); MR 907945 (88j:11074).Google Scholar
Rohrlich, D. E., On L-functions of elliptic curves and cyclotomic towers, Invent. Math. 75 (1984), 409–423; doi:10.1007/BF01388636; MR 735333 (86g:11038b).Google Scholar
Schoof, R. J., Class groups of complex quadratic fields, Math. Comp. 41 (1983), 295–302; doi:10.2307/2007782; MR 701640 (84h:12005).Google Scholar
Schaefer, E. F., Class groups and Selmer groups, J. Number Theory 56 (1996), 79–114; doi:10.1006/jnth.1996.0006; MR 1370197 (97e:11068).CrossRefGoogle Scholar
Serre, J.-P., Classes des corps cyclotomiques (d’après K. Iwasawa), Séminaire Bourbaki, vol. 5, Exp. No. 174 (Société Mathématique de France, Paris, 1995), 83–93 (French); MR 1603459.Google Scholar
Serre, J.-P, Local fields, Graduate Texts in Mathematics, vol. 67 (Springer, New York, 1979); Translated from the French by Marvin Jay Greenberg; MR 554237 (82e:12016).Google Scholar
Deligne, P., Cohomologie étale, séminaire de géométrie algébrique du Bois-Marie SGA 4 ${\textstyle \frac{1}{2}}$, Lecture Notes in Mathematics, vol. 569 (Springer, Berlin, ), avec la collaboration de J. F. Boutot, A. Grothendieck, L. Illusie et J. L. Verdier; MR MR 0463174 (57 #3132).Google Scholar
Tate, J. and Oort, F., Group schemes of prime order, Ann. Sci. Éc. Norm. Supér. (4) 3 (1970), 1–21; MR 0265368 (42 #278).Google Scholar
Tèit, D. T. and Šafarevič, I. R., The rank of elliptic curves, Dokl. Akad. Nauk SSSR 175 (1967), 770–773 (Russian); MR 0237508 (38 #5790).Google Scholar
Ulmer, D., L-functions with large analytic rank and abelian varieties with large algebraic rank over function fields, Invent. Math. 167 (2007), 379–408; doi:10.1007/s00222-006-0018-x; MR 2270458 (2007k:11101).Google Scholar
Waterhouse, W. C., Abelian varieties over finite fields, Ann. Sci. Éc. Norm. Supér. (4) 2 (1969), 521–560; MR 0265369 (42 #279).CrossRefGoogle Scholar