Article contents
Arithmetic purity of strong approximation for semi-simple simply connected groups
Published online by Cambridge University Press: 01 February 2021
Abstract
In this article we establish the arithmetic purity of strong approximation for certain semisimple simply connected linear algebraic groups and their homogeneous spaces over a number field 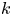 $k$. For instance, for any such group
$k$. For instance, for any such group 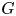 $G$ and for any open subset
$G$ and for any open subset 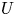 $U$ of
$U$ of  $G$ with
$G$ with 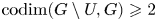 ${\mathrm {codim}}(G\setminus U, G)\geqslant 2$, we prove that (i) if
${\mathrm {codim}}(G\setminus U, G)\geqslant 2$, we prove that (i) if 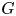 $G$ is
$G$ is 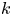 $k$-simple and
$k$-simple and 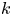 $k$-isotropic, then
$k$-isotropic, then 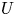 $U$ satisfies strong approximation off any finite number of places; and (ii) if
$U$ satisfies strong approximation off any finite number of places; and (ii) if 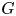 $G$ is the spin group of a non-degenerate quadratic form which is not compact over archimedean places, then
$G$ is the spin group of a non-degenerate quadratic form which is not compact over archimedean places, then 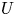 $U$ satisfies strong approximation off all archimedean places. As a consequence, we prove that the same property holds for affine quadratic hypersurfaces. Our approach combines a fibration method with subgroup actions developed for induction on the codimension of
$U$ satisfies strong approximation off all archimedean places. As a consequence, we prove that the same property holds for affine quadratic hypersurfaces. Our approach combines a fibration method with subgroup actions developed for induction on the codimension of 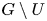 $G\setminus U$, and an affine linear sieve which allows us to produce integral points with almost-prime polynomial values.
$G\setminus U$, and an affine linear sieve which allows us to produce integral points with almost-prime polynomial values.
Information
- Type
- Research Article
- Information
- Copyright
- © The Author(s) 2021
References
- 3
- Cited by


