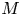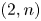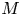Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Krieg, Aloys
and
Schaps, Felix
2022.
Maximal discrete subgroups of 𝑆𝑂⁺(2,𝑛+2).
Proceedings of the American Mathematical Society,
Wang, Haowu
and
Williams, Brandon
2023.
Siegel modular forms of degree two and level five.
The Ramanujan Journal,
Vol. 60,
Issue. 3,
p.
597.
Wang, Haowu
2023.
2-Reflective Lattices of Signature (n, 2) withn≥8.
International Mathematics Research Notices,
Vol. 2023,
Issue. 20,
p.
17953.
Wang, Haowu
and
Williams, Brandon
2023.
Simple lattices and free algebras of modular forms.
Advances in Mathematics,
Vol. 413,
Issue. ,
p.
108835.
Wang, Haowu
and
Williams, Brandon
2023.
Graded rings of Hermitian modular forms with singularities.
manuscripta mathematica,
Vol. 170,
Issue. 1-2,
p.
283.
Duhr, Claude
2025.
Modular forms for three-loop banana integrals.
Journal of High Energy Physics,
Vol. 2025,
Issue. 8,
Wang, Haowu
and
Williams, Brandon
2025.
Free algebras of modular forms on ball quotients.
Research in the Mathematical Sciences,
Vol. 12,
Issue. 3,
Wang, Haowu
and
Williams, Brandon
2025.
Projective Spaces as Orthogonal Modular Varieties.
Transformation Groups,
Vol. 30,
Issue. 2,
p.
933.
Wang, Haowu
2025.
On the classification of reflective modular forms.
European Journal of Mathematics,
Vol. 11,
Issue. 3,
Wang, Haowu
and
Williams, Brandon
2025.
The fake monster algebra and singular Borcherds products.
Advances in Mathematics,
Vol. 461,
Issue. ,
p.
110083.