Correlations of multiplicative functions and applications

Abstract

We give an asymptotic formula for correlations

$$\begin{eqnarray}\mathop{\sum }_{n\leqslant x}f_{1}(P_{1}(n))f_{2}(P_{2}(n))\cdots f_{m}(P_{m}(n)),\end{eqnarray}$$

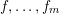where $f,\ldots ,f_{m}$ are bounded ‘pretentious’ multiplicative functions, under certain natural hypotheses. We then deduce several desirable consequences. First, we characterize all multiplicative functions 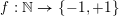$f:\mathbb{N}\rightarrow \{-1,+1\}$ with bounded partial sums. This answers a question of Erdős from $1957$ in the form conjectured by Tao. Second, we show that if the average of the first divided difference of the multiplicative function is zero, then either $f(n)=n^{s}$ for 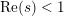$\operatorname{Re}(s)<1$ or 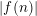$|f(n)|$ is small on average. This settles an old conjecture of Kátai. Third, we apply our theorem to count the number of representations of 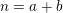$n=a+b$, where 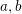$a,b$ belong to some multiplicative subsets of 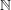$\mathbb{N}$. This gives a new ‘circle method-free’ proof of a result of Brüdern.

1 Introduction

Let $\mathbb{U}$ denote the unit disc and let $\mathbb{T}$ be the unit circle. It is of current interest in analytic number theory to understand the correlations

$$\begin{eqnarray}\mathop{\sum }_{n\leqslant x}f_{1}(P_{1}(n))f_{2}(P_{2}(n))\cdot \cdots \cdot f_{m}(P_{m}(n))\end{eqnarray}$$

for arbitrary multiplicative functions $f_{1},\ldots ,f_{m}:\mathbb{N}\rightarrow \mathbb{U}$ and arbitrary polynomials $P_{1},\ldots ,P_{m}\in \mathbb{Z}[x]$ . For example, Chowla’s conjecture says that, for any distinct natural numbers $h_{1},\ldots ,h_{k}$ ,

$$\begin{eqnarray}\mathop{\sum }_{n\leqslant x}\unicode[STIX]{x1D706}(n+h_{1})\cdots \unicode[STIX]{x1D706}(n+h_{k})=o(x),\end{eqnarray}$$

where $\unicode[STIX]{x1D706}(n)$ is a Liouville function. These problems are still wide open in general, though spectacular progress has been made recently due to the breakthrough of Matomäki and Radziwiłł [MR16] and subsequent work of Matomäki et al. [MRT15]. In particular, this led Tao [Tao16b] to establish a weighted version of Chowla’s conjecture in the form

$$\begin{eqnarray}\mathop{\sum }_{n\leqslant x}\frac{\unicode[STIX]{x1D706}(n)\unicode[STIX]{x1D706}(n+h)}{n}=o(\log x)\end{eqnarray}$$

for all $h\geqslant 1$ . Combining this with ideas from the Polymath5 project, and a new ‘entropy decrement argument’, led to the resolution of the Erdős discrepancy problem.

Following Granville and Soundararajan [GS07a], we define the ‘distance’ between two multiplicative functions $f,g:\mathbb{N}\rightarrow \mathbb{U}$ :

$$\begin{eqnarray}\mathbb{D}(f,g;y;x)=\biggl(\mathop{\sum }_{y\leqslant p\leqslant x}\frac{1-\text{Re}(f(p)\overline{g(p)})}{p}\biggr)^{1/2}\end{eqnarray}$$

and $\mathbb{D}(f,g;x):=\mathbb{D}(f,g;1;x)$ . The crucial feature of this ‘distance’ is that it satisfies the triangle inequality

$$\begin{eqnarray}\mathbb{D}(f,g;y;x)+\mathbb{D}(g,h;y;x)\geqslant \mathbb{D}(f,h;y;x)\end{eqnarray}$$

for any multiplicative functions $f,g,h$ bounded by $1$ .

Halász’s theorem [Hal71, Hal75] implies Wirsing’s theorem that for multiplicative $f:\mathbb{N}\rightarrow [-1,1]$ , the mean value satisfies a decomposition into local factors,

(1) $$\begin{eqnarray}\frac{1}{x}\mathop{\sum }_{n\leqslant x}f(n)=\mathop{\prod }_{p}M_{p}(f)+o(1)\end{eqnarray}$$

when $x\rightarrow \infty$ , where we define the multiplicative function $f_{p}$ for each prime $p$ to be

(2) $$\begin{eqnarray}f_{p}(q^{k})=\left\{\begin{array}{@{}ll@{}}f(q^{k})\quad & \text{if }q=p,\\ 1\quad & \text{if }q\neq p\end{array}\right.\end{eqnarray}$$

for all $k\geqslant 1$ and

$$\begin{eqnarray}M_{p}(f):=\lim _{x\rightarrow \infty }\frac{1}{x}\mathop{\sum }_{n\leqslant x}f_{p}(n)=\biggl(1-\frac{1}{p}\biggr)\mathop{\sum }_{k\geqslant 0}\frac{f(p^{k})}{p^{k}}.\end{eqnarray}$$

This last equality, evaluating $M_{p}(f)$ , is an easy exercise. Substituting this into (1), one finds that the mean value there is $\asymp \exp (-\mathbb{D}(f,1;\infty ))^{2}$ and so is non-zero if and only if $\mathbb{D}(f,1;\infty )<\infty$ and each $M_{p}(f)\neq 0$ . Moreover, using our explicit evaluation of $M_{p}(f)$ , we see that $M_{p}(f)=0$ if and only if $p=2$ and $f(2^{k})=-1$ for all $k\geqslant 1$ . We also note that one can truncate the product in (1) to the primes $p\leqslant x$ and retain the same qualitative result.

1.1 Mean values of multiplicative functions acting on polynomials

Our first goal is to prove the analog of (1) for the mean value of $f(P(n))$ for any given polynomial $P(x)\in \mathbb{Z}[x]$ . This is not difficult for linear polynomials $P$ but, as the following example shows, it is not so straightforward for higher degree polynomials.

Proposition 1.1. There exists a multiplicative function $f:\mathbb{N}\rightarrow [-1,1]$ such that $\mathbb{D}^{2}(1,f;x)=2\log \log x+O(1)$ for all $x\geqslant 2$ and

$$\begin{eqnarray}\limsup _{x\rightarrow \infty }\biggl|\frac{1}{x}\mathop{\sum }_{n\leqslant x}f(n^{2}+1)\biggr|\geqslant \frac{1}{2}+o(1).\end{eqnarray}$$

In the proof of Proposition 1.1 (see § 2), the choice of $f(p)$ for certain primes $p\geqslant x$ has a significant impact on the mean value of $f(n^{2}+1)$ up to $x$ . In order to tame this effect, we introduce the set

$$\begin{eqnarray}N_{P}(x)=\{p^{k},p\geqslant x\mid \exists n\leqslant x,p^{k}\Vert P(n)\}\end{eqnarray}$$

for any given $P\in \mathbb{Z}[x]$ and modify the ‘distance’ to

$$\begin{eqnarray}\mathbb{D}_{P}(f,g;y;x)=\biggl(\mathop{\sum }_{y\leqslant p\leqslant x}\frac{1-\text{Re}(f(p)\overline{g(p)})}{p}+\mathop{\sum }_{p^{k}\in N_{P}(x)}\frac{1-\text{Re}(f(p^{k})\overline{g(p^{k})})}{x}\biggr)^{1/2}\end{eqnarray}$$

and $\mathbb{D}_{P}(f,g;x):=\mathbb{D}_{P}(f,g;1;x)$ . Moreover, we define

$$\begin{eqnarray}M_{p}(f(P))=\lim _{x\rightarrow \infty }\frac{1}{x}\mathop{\sum }_{n\leqslant x}f_{p}(P(n))\end{eqnarray}$$

and one easily shows that

$$\begin{eqnarray}M_{p}(f(P))=\mathop{\sum }_{k\geqslant 0}f(p^{k})\biggl(\frac{\unicode[STIX]{x1D714}_{P}(p^{k})}{p^{k}}-\frac{\unicode[STIX]{x1D714}_{P}(p^{k+1})}{p^{k+1}}\biggr),\end{eqnarray}$$

where $\unicode[STIX]{x1D714}_{P}(m):=\#\{n\hspace{0.6em}({\rm mod}\hspace{0.2em}m):P(n)\equiv 0\hspace{0.6em}({\rm mod}\hspace{0.2em}m)\}$ for every integer $m$ (and note that $\unicode[STIX]{x1D714}_{P}(.)$ is a multiplicative function by the Chinese remainder theorem). We establish the following analog of (1).

Corollary 1.2. Let $f:\mathbb{N}\rightarrow \mathbb{U}$ be a multiplicative function and let $P(x)\in \mathbb{Z}[x]$ be a polynomial. Then

$$\begin{eqnarray}\frac{1}{x}\mathop{\sum }_{n\leqslant x}f(P(n))=\mathop{\prod }_{p\leqslant x}M_{p}(f(P))+O\biggl(\mathbb{D}_{P}(1,f;\log x;x)+\frac{1}{\log \log x}\biggr).\end{eqnarray}$$

This implies that if $\mathbb{D}(1,f;x)<\infty$ and

$$\begin{eqnarray}\mathop{\sum }_{p^{k}\in N_{P}(x)}1-\operatorname{Re}(f(p^{k}))=o(x),\end{eqnarray}$$

then

$$\begin{eqnarray}\frac{1}{x}\mathop{\sum }_{n\leqslant x}f(P(n))=\mathop{\prod }_{p\leqslant x}M_{p}(f(P))+o(1)=\mathop{\prod }_{p\geqslant 1}M_{p}(f(P))+o(1)\end{eqnarray}$$

when $x\rightarrow \infty$ .

1.2 Mean values of correlations of multiplicative functions

We now move on to correlations. For $P,Q\in \mathbb{Z}[x]$ , we define the local correlation

(3) $$\begin{eqnarray}M_{p}(f(P),g(Q))=\lim _{x\rightarrow \infty }\frac{1}{x}\mathop{\sum }_{n\leqslant x}f_{p}(P(n))g_{p}(Q(n)).\end{eqnarray}$$

Evaluating these local factors is also easy yet can be technically complicated, as we shall see below in the case that $P$ and $Q$ are both linear.

More generally, we establish the following result.

Theorem 1.3. Let $f,g:\mathbb{N}\rightarrow \mathbb{U}$ be multiplicative functions. Let $P,Q\in \mathbb{Z}[x]$ be two polynomials such that $\text{res}(P,Q)\neq 0$ . Then

$$\begin{eqnarray}\frac{1}{x}\mathop{\sum }_{n\leqslant x}f(P(n))g(Q(n))=\mathop{\prod }_{p\leqslant x}M_{p}(f(P),g(Q))+\text{Error}(f(P),g(Q),x),\end{eqnarray}$$

where

$$\begin{eqnarray}\text{Error}(f(P),g(Q),x)\ll \mathbb{D}_{P}(1,f;\log x;x)+\mathbb{D}_{Q}(1,g;\log x;x)+\frac{1}{\log \log x}.\end{eqnarray}$$

Theorem 1.3 implies that if $\mathbb{D}(1,f;x),\mathbb{D}(1,g;x)<\infty$ and $\sum _{p\in N_{P}(x)}1-\operatorname{Re}(f(p^{k}))=o(x)$ and $\sum _{p\in N_{Q}(x)}1-\operatorname{Re}(g(p^{k}))=o(x)$ , then

$$\begin{eqnarray}\frac{1}{x}\mathop{\sum }_{n\leqslant x}f(P(n))g(Q(n))=\mathop{\prod }_{p\leqslant x}M_{p}(f(P),g(Q))+o(1)=\mathop{\prod }_{p\geqslant 1}M_{p}(f(P),g(Q))+o(1).\end{eqnarray}$$

If $\mathbb{D}_{P}(f,n^{it};\infty ),\mathbb{D}_{P}(g,n^{iu};\infty )<\infty$ , then we let $f_{0}(n)=f(n)/n^{it}$ and $g_{0}(n)=g(n)/n^{iu}$ so that $\mathbb{D}_{P}(1,f_{0};\infty ),\mathbb{D}_{P}(1,g_{0};\infty )<\infty$ . We apply Theorem 1.3 to the mean value of $f_{0}(P(n))g_{0}(Q(n))$ and then proceed by partial summation to obtain

$$\begin{eqnarray}\frac{1}{x}\mathop{\sum }_{n\leqslant x}f(P(n))g(Q(n))=M_{i}(f(P),g(Q),x)\mathop{\prod }_{p\leqslant x}M_{p}(f_{0}(P),g_{0}(Q))+\text{Error}(f_{0}(P),g_{0}(Q),x),\end{eqnarray}$$

where, if $P(x)=ax^{D}+\cdots \,$ and $Q(x)=bx^{d}+\cdots \,$ , then we define $T=Dt+du$ and

$$\begin{eqnarray}M_{i}(f(P),g(Q),x):=\frac{1}{x}\mathop{\sum }_{n\leqslant x}P(n)^{it}Q(n)^{iu}=a^{it}b^{iu}\frac{x^{iT}}{1+iT}+o(1).\end{eqnarray}$$

Here, the $o(1)$ term depends on the polynomials $P,Q\in \mathbb{Z}[x]$ and

$$\begin{eqnarray}\text{Error}(f_{0}(P),g_{0}(Q),x)\ll _{t,u}\mathbb{D}_{P}(1,f_{0};\log x;x)+\mathbb{D}_{Q}(1,g_{0};\log x;x)+\frac{1}{\log \log x},\end{eqnarray}$$

where the implied constant depends on $t,u$ . The same method works for $m$ -point correlations

$$\begin{eqnarray}\mathop{\sum }_{n\leqslant x}f_{1}(P_{1}(n))f_{2}(P_{2}(n))\cdot \cdots \cdot f_{m}(P_{m}(n))\end{eqnarray}$$

for multiplicative functions $f_{j}:\mathbb{N}\rightarrow \mathbb{U}$ and polynomials $P_{j}$ with each $\mathbb{D}_{P_{j}}(n^{it_{j}},f_{j},\infty )<\infty$ . We give a more explicit version of our results in the case that $P$ and $Q$ are linear polynomials.

Corollary 1.4. Let $f,g:\mathbb{N}\rightarrow \mathbb{U}$ be multiplicative functions with $\mathbb{D}(f,n^{it},\infty )$ , $\mathbb{D}(g,n^{iu},\infty )<\infty$ and write $f_{0}(n)=f(n)/n^{it}$ and $g_{0}(n)=g(n)/n^{iu}$ . Let $a,b\geqslant 1$ , $c,d$ be integers with $(a,c)=(b,d)=1$ and $ad\neq bc$ . As above we have

$$\begin{eqnarray}\frac{1}{x}\mathop{\sum }_{n\leqslant x}f(an+c)g(bn+d)=M_{i}(f(P),g(Q),x)\mathop{\prod }_{p\leqslant x}M_{p}(f_{0}(P),g_{0}(Q))+o(1)\end{eqnarray}$$

when $x\rightarrow \infty$ and the $o(1)$ term depends on the variables $a,b,c,d,t,u$ .

We have

$$\begin{eqnarray}M_{i}(f(P),g(Q),x)=\frac{a^{it}b^{iu}x^{i(t+u)}}{1+i(t+u)}+o(1)\end{eqnarray}$$

when $x\rightarrow \infty$ and the $o(1)$ term depends on $a,b,t,u$ .

If $p|(a,b)$ , then $M_{p}(f_{0}(P),g_{0}(Q))=1$ . If $p\nmid ab(ad-bc)$ , then

$$\begin{eqnarray}M_{p}(f_{0}(P),g_{0}(Q))=M_{p}(f_{0}(P))+M_{p}(g_{0}(Q))-1=1+\biggl(1-\frac{1}{p}\biggr)\biggl(\mathop{\sum }_{j\geqslant 1}\frac{f_{0}(p^{j})}{p^{j}}+\mathop{\sum }_{j\geqslant 1}\frac{g_{0}(p^{j})}{p^{j}}\biggr).\end{eqnarray}$$

In general, if $p\nmid (a,b)$ , we have a more complicated formula

$$\begin{eqnarray}\displaystyle M_{p}(f_{0}(P),g_{0}(Q))=\mathop{\sum }_{\substack{ 0\leqslant i\leqslant k, \\ k\geqslant 0, \\ p^{k}\Vert ad-bc}}\biggl(\frac{\unicode[STIX]{x1D703}(p^{i})\unicode[STIX]{x1D6FE}(p^{i})}{p^{i}}+\unicode[STIX]{x1D6FF}_{b}\mathop{\sum }_{j>i}\frac{\unicode[STIX]{x1D703}(p^{i})\unicode[STIX]{x1D6FE}(p^{j})}{p^{j}}+\unicode[STIX]{x1D6FF}_{a}\mathop{\sum }_{j>i}\frac{\unicode[STIX]{x1D6FE}(p^{i})\unicode[STIX]{x1D703}(p^{j})}{p^{j}}\biggr) & & \displaystyle \nonumber\end{eqnarray}$$

and $\unicode[STIX]{x1D6FF}_{l}=0$ when $p|l$ and $\unicode[STIX]{x1D6FF}_{l}=1$ otherwise. Here, $f_{0}=1\ast \unicode[STIX]{x1D703}$ and $g_{0}=1\ast \unicode[STIX]{x1D6FE}$ .

For $t=u=0$ , some version of Corollary 1.4 also appeared in Hildebrand [Hil88a], Elliott [Ell92] and Stepanauskas [Ste02].

Next we apply Theorem 1.3 to obtain a number of consequences. The key idea for our applications is that one expands

$$\begin{eqnarray}\displaystyle \frac{1}{x}\mathop{\sum }_{n\leqslant x}\biggl|\mathop{\sum }_{k=n+1}^{n+H+1}f(k)\biggr|^{2}=\mathop{\sum }_{|h|\leqslant H}(H-|h|)\mathop{\sum }_{n\leqslant x}f(n)\overline{f(n+h)}+O\biggl(\frac{H^{2}}{x}\biggr) & & \displaystyle \nonumber\end{eqnarray}$$

and then one observes that the $h=0$ term equals $H$ if each $|f(n)|=1$ . Therefore, if the above sum is small, then

$$\begin{eqnarray}\frac{1}{x}\mathop{\sum }_{n\leqslant x}f(n)\overline{f(n+h)}\gg 1\end{eqnarray}$$

for some $h$ , $1\leqslant |h|\leqslant H$ . As Tao showed, if some weighted version of this is true, then $\mathbb{D}(f(n),\unicode[STIX]{x1D712}(n)n^{it};x)\ll 1$ for some primitive character $\unicode[STIX]{x1D712}$ . Therefore, to understand the above better, we need to give a version of Theorem 1.3 for functions $f$ with $\mathbb{D}(f(n),\unicode[STIX]{x1D712}(n)n^{it};x)\ll 1$ .

1.3 Correlations with characters

Now we will suppose that $\mathbb{D}(f(n),n^{it}\unicode[STIX]{x1D712}(n),\infty )<\infty$ for some $t\in \mathbb{R}$ , where $\unicode[STIX]{x1D712}$ is a primitive character of conductor $q$ . We define $F$ to be the multiplicative function such that

$$\begin{eqnarray}\displaystyle F(p^{k})=\left\{\begin{array}{@{}ll@{}}f(p^{k})\overline{\unicode[STIX]{x1D712}(p^{k})}p^{-ikt}\quad & \text{if }p\nmid q,\\ 1\quad & \text{if }p\mid q\end{array}\right. & & \displaystyle \nonumber\end{eqnarray}$$

and

$$\begin{eqnarray}M_{p}(F,\overline{F};d)=\lim _{x\rightarrow \infty }\frac{1}{x}\mathop{\sum }_{n\leqslant x}F_{p}(n)\overline{F_{p}(n+d)}.\end{eqnarray}$$

In § 3, we prove the following result.

Theorem 1.5. Let $f:\mathbb{N}\rightarrow \mathbb{U}$ be a multiplicative function such that $\mathbb{D}(f(n),n^{it}\unicode[STIX]{x1D712}(n);\infty )<\infty$ for some $t\in \mathbb{R}$ and $\unicode[STIX]{x1D712}$ be a primitive character of conductor $q$ . Then, for any non-zero integer $d$ , we have

$$\begin{eqnarray}\frac{1}{x}\mathop{\sum }_{n\leqslant x}f(n)\overline{f(n+d)}=\mathop{\prod }_{\substack{ p\leqslant x \\ p\nmid q}}M_{p}(F,\overline{F};d)\mathop{\prod }_{p^{l}\Vert q}M_{p^{l}}(f,\overline{f},d)+o(1)\end{eqnarray}$$

when $x\rightarrow \infty$ . Here, the $o(1)$ term depends on $d,\unicode[STIX]{x1D712},t$ and

$$\begin{eqnarray}M_{p^{l}}(f,\overline{f},d)=\left\{\begin{array}{@{}ll@{}}0\quad & \text{if }p^{l-1}\nmid d,\\ \displaystyle 1-\frac{1}{p}\quad & \text{if }p^{l-1}\Vert d,\\ \displaystyle \biggl(1-\frac{1}{p}\biggr)\mathop{\sum }_{j=0}^{k}\frac{|f(p^{j})|^{2}}{p^{j}}-\frac{|f(p^{k})|^{2}}{p^{k}}\quad & \text{if }p^{l+k}\Vert d\end{array}\right.\end{eqnarray}$$

for any $k\geqslant 0$ and, if $p^{n}\Vert d$ for some $n\geqslant 0$ , then

$$\begin{eqnarray}M_{p}(F,\overline{F},d)=1-\frac{2}{p^{n+1}}+\biggl(1-\frac{1}{p}\biggr)\mathop{\sum }_{j>n}\biggl(\frac{F(p^{n})\overline{F(p^{j})}}{p^{j}}+\frac{\overline{F(p^{n})}F(p^{j})}{p^{j}}\biggr).\end{eqnarray}$$

In particular, the mean value is $o(1)$ if $q\nmid d\prod _{p|q}p$ .

The same method works for correlations:

$$\begin{eqnarray}\mathop{\sum }_{n\leqslant x}f(n)g(n+m),\end{eqnarray}$$

where $\mathbb{D}(f(n),n^{it}\unicode[STIX]{x1D712}(n);\infty ),\mathbb{D}(g(n),n^{iu}\unicode[STIX]{x1D713}(n);\infty )<\infty$ .

1.4 The Erdős discrepancy problem for multiplicative functions

The Polymath5 project showed, using Fourier analysis, that the Erdős discrepancy problem can be reduced to a statement about completely multiplicative functions. In particular, Tao [Tao16a] established that for any completely multiplicative $f:\mathbb{N}\rightarrow \{-1,1\}$ ,

$$\begin{eqnarray}\limsup _{x\rightarrow \infty }\biggl|\mathop{\sum }_{n\leqslant x}f(n)\biggr|=\infty .\end{eqnarray}$$

In [Erd57, Erd85a, Erd85b], Erdős, along with the Erdős discrepancy problem, asked to classify all multiplicative $f:\mathbb{N}\rightarrow \{-1,1\}$ such that

(4) $$\begin{eqnarray}\limsup _{x\rightarrow \infty }\biggl|\mathop{\sum }_{n\leqslant x}f(n)\biggr|<\infty .\end{eqnarray}$$

In [Tao16a], Tao, partially answering this question, proved that if for a multiplicative $f:\mathbb{N}\rightarrow \{-1,1\}$ , (4) holds, then $f(2^{j})=-1$ for all $j$ and

(5) $$\begin{eqnarray}\mathop{\sum }_{p}\frac{1-f(p)}{p}<\infty .\end{eqnarray}$$

In § 4, we resolve this question completely by proving the following result.

Theorem 1.6 (Erdős–Coons–Tao conjecture).

Let $f:\mathbb{N}\rightarrow \{-1,1\}$ be a multiplicative function. Then (4) holds if and only if there exists an integer $m\geqslant 1$ such that $f(n+m)=f(n)$ for all $n\geqslant 1$ and $\sum _{n=1}^{m}f(n)=0$ .

There are examples known with bounded sums, such as the multiplicative function $f$ for which $f(n)=+1$ when $n$ is odd and $f(n)=-1$ when $n$ is even. One can easily show that $f$ satisfies the above hypotheses if and only if $m$ is even, $f(2^{k})=-1$ for all $k\geqslant 1$ and $f(p^{k})=f((p^{k},m))$ for all odd prime powers $p^{k}$ . In particular, if $p$ does not divide $m$ , then $f(p^{k})=1$ .

It would be interesting to classify all complex-valued multiplicative $f:\mathbb{N}\rightarrow \mathbb{T}$ for which (4) holds. Using Theorem 1.5, it easy to prove the following result.

Theorem 1.7. Suppose for a multiplicative $f:\mathbb{N}\rightarrow \mathbb{T}$ that (15) holds. Then there exists a primitive character $\unicode[STIX]{x1D712}$ of an odd conductor $q$ and $t\in \mathbb{R}$ such that $\mathbb{D}(f(n),\unicode[STIX]{x1D712}(n)n^{it};\infty )<\infty$ and $f(2^{k})=-\unicode[STIX]{x1D712}^{k}(2)2^{-ikt}$ for all $k\geqslant 1$ .

1.5 Distribution of $(f(n),f(n+1))$

Let $f:\mathbb{N}\rightarrow \mathbb{C}$ be a multiplicative function and $\triangle f(n)=f(n+1)-f(n)$ . Kátai conjectured and Wirsing proved (first in a letter to Kátai and then in a joint paper with Tang and Shao [WTS96]) that if a unimodular multiplicative function $f$ satisfies $\triangle f(n)\rightarrow 0$ , then $f(n)=n^{it}$ (see also a nice paper of Wirsing and Zagier [WZ01] for a simpler proof). One would naturally expect that if $\triangle f(n)\rightarrow 0$ in some averaged sense, then the similar conclusion must hold. Kátai [Kát83] made the following conjecture, which we prove in § 5.

Theorem 1.8 (Kátai’s conjecture, 1983).

If $f:\mathbb{N}\rightarrow \mathbb{C}$ is a multiplicative function and

$$\begin{eqnarray}\displaystyle \lim _{x\rightarrow \infty }\frac{1}{x}\mathop{\sum }_{n\leqslant x}|\triangle f(n)|=0, & & \displaystyle \nonumber\end{eqnarray}$$

then either

$$\begin{eqnarray}\lim _{x\rightarrow \infty }\frac{1}{x}\mathop{\sum }_{n\leqslant x}|f(n)|=0\end{eqnarray}$$

or $f(n)=n^{s}$ for some $\operatorname{Re}(s)<1$ .

Since $f(n)=\text{e}^{h(n)}$ is multiplicative, where $h(n):\mathbb{N}\rightarrow \mathbb{R}$ is an additive function, one may compare Theorem 1.8 with the following statement about additive functions, first conjectured by Erdős [Erd46] and proved later by Kátai [Kát70] (and independently by Wirsing): if $h:\mathbb{N}\rightarrow \mathbb{C}$ is an additive function and

$$\begin{eqnarray}\lim _{x\rightarrow \infty }\frac{1}{x}\mathop{\sum }_{n\leqslant x}|h(n+1)-h(n)|=0,\end{eqnarray}$$

then $h(n)=c\log n$ .

The conjecture attracted considerable attention of several authors including Kátai, Hildebrand, Phong and others. See, for example, [Hil88b], [Pho14], [Pho00] and [Kát91] for some of the results and the survey paper [Kát00] with an extensive list of the related references.

1.6 Binary additive problems

A sequence $A$ of positive integers is called multiplicative if its characteristic function, $1_{A}$ , is multiplicative. We define

$$\begin{eqnarray}\unicode[STIX]{x1D70C}_{A}(d)=\lim _{x\rightarrow \infty }\frac{1}{x/d}\mathop{\sum }_{k\leqslant x/d}\text{I}_{A}(kd)\end{eqnarray}$$

with $\unicode[STIX]{x1D70C}_{A}=\unicode[STIX]{x1D70C}_{A}(1)$ , which is the density of $A$ . Note that these constants all exist by Wirsing’s theorem.

Binary additive problems, which involve estimating quantities like

$$\begin{eqnarray}r(n)=|\{(a,b)\in A\times B:a+b=n\}|,\end{eqnarray}$$

are considered difficult. However, using a variant of a circle method, Brüdern [Brü09], among other things, established the following theorem, which we will deduce from Theorem 1.3 in § 6.

Theorem 1.9 (Brüdern, 2008).

Suppose that $A$ and $B$ are multiplicative sequences of positive densities $\unicode[STIX]{x1D70C}_{A}$ and $\unicode[STIX]{x1D70C}_{B}$ , respectively. For $k\geqslant 1$ , let

$$\begin{eqnarray}a(p^{k})=\unicode[STIX]{x1D70C}_{A}(p^{k})/p^{k}-\unicode[STIX]{x1D70C}_{A}(p^{k-1})/p^{k-1}.\end{eqnarray}$$

Define $b(p^{k})$ in the same fashion. Then

$$\begin{eqnarray}r(n)=\unicode[STIX]{x1D70C}_{A}\unicode[STIX]{x1D70C}_{B}\unicode[STIX]{x1D70E}(n)n+o(n)\end{eqnarray}$$

when $n\rightarrow \infty$ , where

$$\begin{eqnarray}\unicode[STIX]{x1D70E}(n)=\mathop{\prod }_{p^{m}\Vert n}\biggl(1+\mathop{\sum }_{k=1}^{m}\frac{p^{k-1}a(p^{k})b(p^{k})}{p-1}-\frac{p^{m}a(p^{m+1})b(p^{m+1})}{(p-1)^{2}}\biggr).\end{eqnarray}$$

2 Multiplicative functions of polynomials

For any given polynomial $P(x)\in \mathbb{Z}[x]$ , we define $\unicode[STIX]{x1D714}_{P}(p^{k})$ to be the number of solutions of $P(x)=0~(\text{mod}(p^{k}))$ . Clearly, $\unicode[STIX]{x1D714}_{P}(p^{k})\leqslant \deg P$ for all but finitely many primes $p$ . We begin by showing that the mean value of $f(P(n))$ in general significantly depends on the large primes. We restrict ourselves to the case $P(x)=x^{2}+1$ but the same arguments work for all polynomials $P(x)\in \mathbb{Z}[x]$ that are not products of linear factors.

Lemma 2.1. Let $P(x)=x^{2}+1$ . For any $x\geqslant 2$ and any complex numbers $g(p^{k})\in \mathbb{T}$ , $p\leqslant 2x$ and $k\geqslant 1$ , there exists a multiplicative function $f:\mathbb{N}\rightarrow \mathbb{T}$ such that $f(p^{k})=g(p^{k})$ for all $p\leqslant 2x$ and

$$\begin{eqnarray}\biggl|\frac{1}{x}\mathop{\sum }_{n\leqslant x}f(P(n))\biggr|\geqslant \frac{1}{2}+o(1).\end{eqnarray}$$

Proof. Let

$$\begin{eqnarray}\mathfrak{M}(x)=\{n_{p}\leqslant x\mid \exists p\in N_{P}(x),p|P(n_{p})\}.\end{eqnarray}$$

We note that for each $p\geqslant 2x$ , there exists at most one element $n_{p}\in \mathfrak{M}(x)$ such that $p|P(n_{p})$ and moreover all prime factors of $P(n_{p})/p$ are smaller than $x$ . We have

$$\begin{eqnarray}\displaystyle 2x\log x+O(x) & = & \displaystyle \mathop{\sum }_{n\leqslant x}\log P(n)=\mathop{\sum }_{n\leqslant x}\mathop{\sum }_{d|P(n)}\unicode[STIX]{x1D6EC}(d)\nonumber\\ \displaystyle & {\leqslant} & \displaystyle 2\mathop{\sum }_{\substack{ p\leqslant x, \\ p=1\,\text{mod}(4)}}\log p\cdot \frac{x}{p}+\mathop{\sum }_{\substack{ p>2x, \\ p|P(n_{p}), \\ n_{p}\leqslant x}}\log p+O(x)\nonumber\\ \displaystyle & {\leqslant} & \displaystyle x\log x+2\log x\cdot |\mathfrak{M}(x)|+O(x)\nonumber\end{eqnarray}$$

and therefore

$$\begin{eqnarray}|\mathfrak{M}(x)|\geqslant x({\textstyle \frac{1}{2}}+o(1)).\end{eqnarray}$$

Consider the multiplicative function $f$ defined as follows: $f(p^{k})=g(p^{k})$ for all primes $p\leqslant 2x$ and

$$\begin{eqnarray}f(p)=e^{i\unicode[STIX]{x1D719}}\overline{f\biggl(\frac{P(n_{p})}{p}\biggr)}\end{eqnarray}$$

if $p>2x$ and there exists $n_{p}\in \mathfrak{M}(x)$ such that $p|P(n_{p})$ , where

$$\begin{eqnarray}\unicode[STIX]{x1D719}=\text{arg}\biggl(\mathop{\sum }_{\substack{ n\in \overline{\mathfrak{M}(x)} \\ n\leqslant x}}f(P(n))\biggr).\end{eqnarray}$$

Define $f(p^{k})=1$ for all other primes and all $k\geqslant 1$ . Clearly,

$$\begin{eqnarray}\displaystyle \mathop{\sum }_{n\leqslant x}f(P(n))=\mathop{\sum }_{\substack{ n\in \overline{\mathfrak{M}(x)} \\ n\leqslant x}}f(P(n))+\mathop{\sum }_{n_{p}\in \mathfrak{M}(x)}f(P(n_{p}))=\mathop{\sum }_{\substack{ n\in \overline{\mathfrak{M}(x)}, \\ n\leqslant x}}f(P(n))+e^{i\unicode[STIX]{x1D719}}|\mathfrak{M}(x)|. & & \displaystyle \nonumber\end{eqnarray}$$

Selecting $\unicode[STIX]{x1D719}$ so that the two sums point in the same direction, we deduce that

$$\begin{eqnarray}\biggl|\frac{1}{x}\mathop{\sum }_{n\leqslant x}f(P(n))\biggr|\geqslant \frac{|\mathfrak{M}(x)|}{x}\geqslant \frac{1}{2}+o(1).\square\end{eqnarray}$$

Proposition 1.1 . There exists a multiplicative function $f:\mathbb{N}\rightarrow [-1,1]$ such that $\mathbb{D}^{2}(1,f;x)=2\log \log x+O(1)$ for all $x\geqslant 2$ and

$$\begin{eqnarray}\limsup _{x\rightarrow \infty }\biggl|\frac{1}{x}\mathop{\sum }_{n\leqslant x}f(n^{2}+1)\biggr|\geqslant \frac{1}{2}+o(1).\end{eqnarray}$$

Proof. Take the sequence $x_{k}=2^{2^{k}}$ for $k\geqslant 1$ and define a completely multiplicative function $f$ inductively: $f(p)=-1$ for all primes in $p\in (x_{k},x_{k+1}]$ unless $p\in N_{P}(x_{k})$ , in which case we define the function as in the proof of Lemma 2.1. This guarantees that for all $k\geqslant 1$ ,

$$\begin{eqnarray}\biggl|\frac{1}{x_{k}}\mathop{\sum }_{n\leqslant x_{k}}f(n^{2}+1)\biggr|\geqslant \frac{1}{2}+o(1).\end{eqnarray}$$

Since $N_{P}(x)$ contains at most $x$ elements, we have

$$\begin{eqnarray}\mathop{\sum }_{p\in N_{P}(x)}1/p\leqslant \mathop{\sum }_{x<p\leqslant 2x\log x}1/p\ll (\log \log x)/\text{log}\,x\end{eqnarray}$$

so that $\sum _{k\geqslant 1}\sum _{p\in N_{P}(x_{k})}1/p\ll \sum _{k\geqslant 1}k/2^{k}\ll 1$ . Therefore,

$$\begin{eqnarray}\mathbb{D}^{2}(1,f;x)\geqslant \mathop{\sum }_{\substack{ p\leqslant x \\ p\notin \cup _{k\geqslant 1}N_{P}(x_{k})}}\frac{2}{p}\geqslant 2\log \log x-O(1).\square\end{eqnarray}$$

For technical reasons, we define an equivalent distance

$$\begin{eqnarray}\mathbb{D}^{\ast }(f,g;x)=\biggl(\mathop{\sum }_{p^{k}\leqslant x}\frac{1-\operatorname{Re}(f(p^{k})\overline{g(p^{k})})}{p^{k}}\biggr)^{1/2}.\end{eqnarray}$$

We thus focus on the class of functions such that $f(p)$ is close to $1$ on large primes $p\geqslant x$ where the distance is given by $\mathbb{D}_{P}(1,f;x)$ , where

$$\begin{eqnarray}\mathbb{D}_{P}^{2}(1,f;x)\asymp \mathop{\sum }_{p}(1-\operatorname{Re}f(p^{k}))\cdot \frac{1}{x}\mathop{\sum }_{\substack{ n\leqslant x, \\ p^{k}\Vert P(n)}}1,\end{eqnarray}$$

which generalizes $\mathbb{D}(1,f;x)$ , where

$$\begin{eqnarray}\mathbb{D}^{2}(1,f;x)\asymp {\mathbb{D}^{\ast }}^{2}(1,f;x)\asymp \mathop{\sum }_{p}(1-\operatorname{Re}f(p^{k}))\cdot \frac{1}{x}\mathop{\sum }_{\substack{ n\leqslant x, \\ p^{k}\Vert n}}1.\end{eqnarray}$$

In order to prove Theorem 1.3, we begin by proving a few auxiliary results. The following lemma is a simple consequence of the Turán–Kubilius-type inequality for the polynomial sequences.

Lemma 2.2. Let $h:\mathbb{N}\rightarrow \mathbb{C}$ be an additive function such that $h(p^{k})=0$ for $p^{k}\geqslant x$ and $|h(p^{k})|\leqslant 2$ for all $p$ and $k\geqslant 1$ . Suppose that $P(x)\in \mathbb{Z}[x]$ is irreducible. Define

$$\begin{eqnarray}\unicode[STIX]{x1D707}_{h,P}=\mathop{\sum }_{p^{k}\leqslant x}\frac{h(p^{k})}{p^{k}}\biggl(\unicode[STIX]{x1D714}_{P}(p^{k})-\frac{\unicode[STIX]{x1D714}_{P}(p^{k+1})}{p}\biggr)\end{eqnarray}$$

and

$$\begin{eqnarray}\unicode[STIX]{x1D70E}_{h,P}^{2}=\mathop{\sum }_{p^{k}\leqslant x}\frac{|h(p^{k})|^{2}}{p^{k}}\biggl(\unicode[STIX]{x1D714}_{P}(p^{k})-\frac{\unicode[STIX]{x1D714}_{P}(p^{k+1})}{p}\biggr).\end{eqnarray}$$

Then

(6) $$\begin{eqnarray}\mathop{\sum }_{n\leqslant x}|h(P(n))-\unicode[STIX]{x1D707}_{h,P}|^{2}\ll x\mathop{\sum }_{p^{k}\leqslant x}\frac{|h(p^{k})|^{2}}{p^{k}}+x\frac{(\log \log x)^{3}}{\log x}.\end{eqnarray}$$

Proof. By multiplicativity, we have

$$\begin{eqnarray}|\{n\leqslant x\mid d|P(n)\}|=\frac{\unicode[STIX]{x1D714}_{P}(d)}{d}x+r_{d},\end{eqnarray}$$

where $r_{d}=O(\unicode[STIX]{x1D714}_{P}(d))$ . Furthermore, by [GS07b, Proposition 4] applied to the additive functions in place of strongly additive ones,

$$\begin{eqnarray}\displaystyle \mathop{\sum }_{n\leqslant x}|h(P(n))-\unicode[STIX]{x1D707}_{h,P}|^{2} & {\leqslant}C_{2}x\unicode[STIX]{x1D70E}_{h,P}^{2}+O\biggl(\Bigl(\max _{p\leqslant y}|h(p^{k})|^{2}\Bigr)\biggl(\mathop{\sum }_{p\leqslant x}\frac{\unicode[STIX]{x1D714}_{P}(p)}{p}\biggr)^{2}\mathop{\sum }_{\substack{ d=p_{1}p_{2}, \\ p_{i}\leqslant x}}|r_{d}|\biggr). & \displaystyle \nonumber\end{eqnarray}$$

The error term is bounded by

$$\begin{eqnarray}\Bigl(\max _{p\leqslant x}|h(p^{k})|^{2}\Bigr)\biggl(\mathop{\sum }_{p\leqslant x}\frac{\unicode[STIX]{x1D714}_{P}(p)}{p}\biggr)^{2}\mathop{\sum }_{\substack{ d=p_{1}p_{2}, \\ p_{i}\leqslant x}}|r_{d}|\ll \max _{p\leqslant x}|h(p^{k})|^{2}(\log \log x)^{2}\cdot \frac{x\log \log x}{\log x}.\end{eqnarray}$$

Combining this observation with the estimate

$$\begin{eqnarray}\unicode[STIX]{x1D70E}_{h,P}^{2}\ll \mathop{\sum }_{p^{k}\leqslant x}\frac{|h(p^{k})|^{2}}{p^{k}},\end{eqnarray}$$

we conclude the proof of (6). ◻

In what follows, we are going to focus on two-point correlations but the same method actually works for $m$ -point correlations with mostly notational modifications. Let

$$\begin{eqnarray}\unicode[STIX]{x1D707}_{h,P}=\mathop{\sum }_{p^{k}\leqslant x}h(p^{k})\biggl(\frac{\unicode[STIX]{x1D714}_{P}(p^{k})}{p^{k}}-\frac{\unicode[STIX]{x1D714}_{P}(p^{k+1})}{p^{k+1}}\biggr)\end{eqnarray}$$

and

$$\begin{eqnarray}\mathfrak{P}(f;P;x)=\mathop{\prod }_{p\leqslant x}\biggl(\mathop{\sum }_{k\geqslant 0}f(p^{k})\biggl(\frac{\unicode[STIX]{x1D714}_{P}(p^{k})}{p^{k}}-\frac{\unicode[STIX]{x1D714}_{P}(p^{k+1})}{p^{k+1}}\biggr)\biggr).\end{eqnarray}$$

We also introduce equivalent distance

$$\begin{eqnarray}\mathbb{D}_{P}^{\ast }(f,g;y;x)=\biggl(\mathop{\sum }_{y\leqslant p^{k}\leqslant x}\frac{1-\text{Re}(f(p^{k})\overline{g(p^{k})})}{p^{k}}+\mathop{\sum }_{p^{k}\in N_{P}(x)}\frac{1-\text{Re}(f(p^{k})\overline{g(p^{k})})}{x}\biggr)^{1/2}.\end{eqnarray}$$

We begin by proving the concentration inequality for the values of a multiplicative function $f:\mathbb{N}\rightarrow \mathbb{U}$ .

Proposition 2.3. Let $f:\mathbb{N}\rightarrow \mathbb{U}$ be a multiplicative function. Let $P(n)\in \mathbb{Z}[x]$ . Then

$$\begin{eqnarray}\mathop{\sum }_{n\leqslant x}|f(P(n))-\mathfrak{P}(f;P;x)|^{2}\ll x{\mathbb{D}^{\ast }}_{P}^{2}(1,f;x)+\frac{x(\log \log x)^{3}}{\log x}.\end{eqnarray}$$

Proof. We begin by proving the proposition for the multiplicative function $f$ such that $f(p^{k})=1$ for all $p^{k}\geqslant x$ . Note that $e^{z-1}=z+O(|z-1|^{2})$ for $|z|\leqslant 1$ . By repeatedly applying the triangle inequality, we have that for all $|z_{i}|,|w_{i}|\leqslant 1$ ,

(7) $$\begin{eqnarray}\biggl|\mathop{\prod }_{1\leqslant i\leqslant n}z_{i}-\mathop{\prod }_{1\leqslant i\leqslant n}w_{i}\biggr|\leqslant \mathop{\sum }_{1\leqslant i\leqslant n}|z_{i}-w_{i}|.\end{eqnarray}$$

Therefore,

$$\begin{eqnarray}\displaystyle \mathop{\prod }_{p^{k}\Vert P(n)}e^{f(p^{k})-1}=\mathop{\prod }_{p^{k}\Vert P(n)}(f(p^{k})+O(|f(p^{k})-1|^{2}))=\mathop{\prod }_{p^{k}\Vert P(n)}f(p^{k})+O\biggl(\mathop{\sum }_{p^{k}\Vert P(n)}|f(p^{k})-1|^{2}\biggr) & & \displaystyle \nonumber\end{eqnarray}$$

and

$$\begin{eqnarray}f(P(n))=\mathop{\prod }_{p^{k}\Vert P(n)}f(p^{k})=\mathop{\prod }_{p^{k}\Vert P(n)}e^{f(p^{k})-1}+O\biggl(\mathop{\sum }_{p^{k}\Vert P(n)}|f(p^{k})-1|^{2}\biggr).\end{eqnarray}$$

We now introduce an additive function $h$ such that $h(p^{k})=f(p^{k})-1$ . Clearly,

$$\begin{eqnarray}\displaystyle \mathop{\sum }_{n\leqslant x}|f(P(n))-e^{h(P(n))}|^{2} & \ll & \displaystyle \mathop{\sum }_{n\leqslant x}|f(P(n))-e^{h(P(n))}|\nonumber\\ \displaystyle & \ll & \displaystyle \mathop{\sum }_{n\leqslant x}\mathop{\sum }_{\substack{ p^{k}\Vert P(n), \\ p^{k}\leqslant x}}|f(p^{k})-1|^{2}\ll x\mathop{\sum }_{p^{k}\leqslant x}\frac{|f(p^{k})-1|^{2}}{p^{k}}\ll x{\mathbb{D}^{\ast }}^{2}(f,1;x).\nonumber\end{eqnarray}$$

Since $|e^{a}-e^{b}|\ll |a-b|$ for $\text{Re}(a),\text{Re}(b)\leqslant 0$ , Lemma 2.2 implies that

$$\begin{eqnarray}\displaystyle \mathop{\sum }_{n\leqslant x}|e^{h(P(n))}-e^{\unicode[STIX]{x1D707}_{h,P}}|^{2} & \ll \mathop{\sum }_{n\leqslant x}|h(P(n))-\unicode[STIX]{x1D707}_{h,P}|^{2}\leqslant x{\mathbb{D}^{\ast }}^{2}(f,1;x)+\frac{x(\log \log x)^{3}}{\log x}. & \displaystyle \nonumber\end{eqnarray}$$

We introduce $\unicode[STIX]{x1D707}_{h,P}=\sum _{p\leqslant x}\unicode[STIX]{x1D707}_{h,p}$ , where

$$\begin{eqnarray}\unicode[STIX]{x1D707}_{h,p}=\mathop{\sum }_{p^{k}\leqslant x}h(p^{k})\biggl(\frac{\unicode[STIX]{x1D714}_{P}(p^{k})}{p^{k}}-\frac{\unicode[STIX]{x1D714}_{P}(p^{k+1})}{p^{k+1}}\biggr)\end{eqnarray}$$

and observe that

$$\begin{eqnarray}e^{\unicode[STIX]{x1D707}_{h,p}}=1+\unicode[STIX]{x1D707}_{h,p}+O(\unicode[STIX]{x1D707}_{h,p}^{2})=\mathop{\sum }_{1\leqslant p^{k}\leqslant x}f(p^{k})\biggl(\frac{\unicode[STIX]{x1D714}_{P}(p^{k})}{p^{k}}-\frac{\unicode[STIX]{x1D714}_{P}(p^{k+1})}{p^{k+1}}\biggr)+O\biggl(\frac{1}{x}+\frac{1}{p}\mathop{\sum }_{p^{k}\leqslant x}\frac{|h(p^{k})|}{p^{k}}\biggr).\end{eqnarray}$$

Note that $|e^{\unicode[STIX]{x1D707}_{h,p}}|\leqslant 1$ . Using (7) and the Cauchy–Schwarz inequality once again yields

$$\begin{eqnarray}\displaystyle |e^{\unicode[STIX]{x1D707}_{h,P}}-\mathfrak{P}(f;P;x)|^{2} & {\leqslant} & \displaystyle \biggl(\mathop{\sum }_{p\leqslant x}\biggl|e^{\unicode[STIX]{x1D707}_{h,p}}-\mathop{\sum }_{1\leqslant p^{k}\leqslant x}f(p^{k})\biggl(\frac{\unicode[STIX]{x1D714}_{P}(p^{k})}{p^{k}}-\frac{\unicode[STIX]{x1D714}_{P}(p^{k+1})}{p^{k+1}}\biggr)+O\biggl(\frac{1}{x}\biggr)\biggr|\biggr)^{2}\nonumber\\ \displaystyle & \ll & \displaystyle \biggl(\mathop{\sum }_{p^{k}\leqslant x}\frac{1}{p}\frac{|f(p^{k})-1|}{p^{k}}+\mathop{\sum }_{p\leqslant x}\frac{1}{x}\biggr)^{2}\ll {\mathbb{D}^{\ast }}^{2}(f,1;x)+\frac{1}{\log ^{2}x},\nonumber\end{eqnarray}$$

which, together with the triangle inequality, completes the proof of the lemma in the special case when $f(p^{k})=1$ for $p^{k}\geqslant x$ .

We now consider any multiplicative function $f$ and decompose $f(n)=f_{s}(n)f_{l}(n)$ , where

$$\begin{eqnarray}f_{s}(p^{k})=\left\{\begin{array}{@{}ll@{}}f(p^{k})\quad & \text{if }p^{k}\leqslant x,\\ 1\quad & \text{if }p^{k}>x\end{array}\right.\end{eqnarray}$$

and

$$\begin{eqnarray}f_{l}(p^{k})=\left\{\begin{array}{@{}ll@{}}1\quad & \text{if }p^{k}\leqslant x,\\ f(p^{k})\quad & \text{if }p^{k}>x.\end{array}\right.\end{eqnarray}$$

Note that for a fixed prime power $p^{k}\in N_{P}(x)$ ,

$$\begin{eqnarray}|\{n\leqslant x\mid p^{k}|P(n)\}|\leqslant \unicode[STIX]{x1D714}_{P}(p^{k})\end{eqnarray}$$

and each $P(n)$ is divisible by $\ll \deg P$ elements of $N_{P}(x)$ . Using the Cauchy–Schwarz inequality yields

$$\begin{eqnarray}\displaystyle \mathop{\sum }_{n\leqslant x}|f(P(n))-f_{s}(P(n))|^{2}\ll \mathop{\sum }_{n\leqslant x}\biggl(\mathop{\sum }_{\substack{ p^{k}\Vert P(n), \\ p^{k}\geqslant x}}|f(p^{k})-1|\biggr)^{2} & \ll x\cdot \mathop{\sum }_{p^{k}\in N_{P}(x)}\frac{|f(p^{k})-1|^{2}}{x}. & \displaystyle \nonumber\end{eqnarray}$$

We are left to collect the error terms and note that

$$\begin{eqnarray}{\mathbb{D}^{\ast }}^{2}(1,f;x)+\mathop{\sum }_{p^{k}\in N_{P}(x)}\frac{1-\operatorname{Re}f(p^{k})}{x}={\mathbb{D}_{P}^{\ast }}^{2}(1,f;x).\square\end{eqnarray}$$

Proposition 2.3 immediately implies the following corollary, which will be used in the proof of Theorem 1.3.

Corollary 2.4. Let $f:\mathbb{N}\rightarrow \mathbb{U}$ be a multiplicative function and let $g:\mathbb{N}\rightarrow \mathbb{U}$ be any function. Let $P(n)\in \mathbb{Z}[x]$ . Then

$$\begin{eqnarray}\mathop{\sum }_{n\leqslant x}f(P(n))g(n)=\mathfrak{P}(f;P;x)\mathop{\sum }_{n\leqslant x}g(n)+O\biggl(x\mathbb{D}_{P}^{\ast }(1,f;x)+\frac{x(\log \log x)^{3/2}}{\sqrt{\log x}}\biggr).\end{eqnarray}$$

Proof. Using Proposition 2.3, the triangle inequality and the Cauchy–Schwarz inequality gives

$$\begin{eqnarray}\displaystyle \mathop{\sum }_{n\leqslant x}f(P(n))g(n)-\mathfrak{P}(f;P;x)\mathop{\sum }_{n\leqslant x}g(n) & \ll & \displaystyle \mathop{\sum }_{n\leqslant x}|f(P(n))-\mathfrak{P}(f;P;x)|\nonumber\\ \displaystyle & \ll & \displaystyle \biggl(x\mathop{\sum }_{n\leqslant x}|f(P(n))-\mathfrak{P}(f;P;x)|^{2}\biggr)^{1/2}\nonumber\\ \displaystyle & \ll & \displaystyle x\mathbb{D}_{P}^{\ast }(1,f;x)+\frac{x(\log \log x)^{3/2}}{\sqrt{\log x}}.\square\nonumber\end{eqnarray}$$

Let $f,g:\mathbb{N}\rightarrow \mathbb{U}$ be multiplicative functions. For any two irreducible polynomials $P,Q\in \mathbb{Z}[x]$ , we define

$$\begin{eqnarray}M(f,g;x)=\frac{1}{x}\mathop{\sum }_{n\leqslant x}f(P(n))g(Q(n)).\end{eqnarray}$$

We define $\unicode[STIX]{x1D714}(p^{k},p^{l})$ to be the quantity such that

$$\begin{eqnarray}\{n\leqslant x\mid p^{k}\Vert P(n),p^{l}\Vert Q(n)\}=x\unicode[STIX]{x1D714}(p^{k},p^{l})+O(1).\end{eqnarray}$$

We note that if $p\nmid \text{res}(P,Q)$ , then $\unicode[STIX]{x1D714}(p^{k},p^{l})=0$ unless $k=0$ or $l=0$ . In the latter case

$$\begin{eqnarray}\unicode[STIX]{x1D714}(p^{k},1)=\frac{\unicode[STIX]{x1D714}_{P}(p^{k})}{p^{k}}-\frac{\unicode[STIX]{x1D714}_{P}(p^{k+1})}{p^{k+1}}\end{eqnarray}$$

and

$$\begin{eqnarray}\unicode[STIX]{x1D714}(1,p^{l})=\frac{\unicode[STIX]{x1D714}_{Q}(p^{l})}{p^{l}}-\frac{\unicode[STIX]{x1D714}_{Q}(p^{l+1})}{p^{l+1}}.\end{eqnarray}$$

Furthermore, by the Chinese remainder theorem, we have

$$\begin{eqnarray}\displaystyle & \{n\leqslant x\mid d_{1}|P(n),d_{2}|Q(n)\}=xF(d_{1},d_{2})+O(\unicode[STIX]{x1D714}_{P}(d_{1})\unicode[STIX]{x1D714}_{Q}(d_{2}))=xF(d_{1},d_{2})+O_{\unicode[STIX]{x1D700}}(x^{\unicode[STIX]{x1D700}}) & \displaystyle \nonumber\end{eqnarray}$$

for some multiplicative function $F(d_{1},d_{2})$ and any $\unicode[STIX]{x1D700}>0$ . Our main goal in this section is to prove that the mean value $M(f,g;x)$ satisfies the ‘local-to-global’ principle. We first evaluate the local correlations.

Lemma 2.5. Let $f,g:\mathbb{N}\rightarrow \mathbb{U}$ be multiplicative functions. Define $f_{p},g_{p}$ as in (2). Let $P,Q\in \mathbb{Z}[x]$ and $\text{res}(P,Q)\neq 0$ . Then

$$\begin{eqnarray}\displaystyle \frac{1}{x}\mathop{\sum }_{n\leqslant x}f_{p}(P(n))g_{p}(Q(n))=\mathop{\sum }_{\substack{ p^{k},p^{l}\geqslant 1}}f(p^{k})g(p^{l})\unicode[STIX]{x1D714}(p^{k},p^{l})+O\biggl(\frac{\log x}{x\log p}\biggr). & & \displaystyle \nonumber\end{eqnarray}$$

In particular, if $p\nmid \text{res}(P,Q)$ , then

$$\begin{eqnarray}\displaystyle & & \displaystyle \displaystyle \frac{1}{x}\mathop{\sum }_{n\leqslant x}f_{p}(P(n))g_{p}(Q(n))\nonumber\\ \displaystyle & & \displaystyle \displaystyle \quad =\biggl(\mathop{\sum }_{k\geqslant 0}f(p^{k})\biggl(\frac{\unicode[STIX]{x1D714}_{P}(p^{k})}{p^{k}}-\frac{\unicode[STIX]{x1D714}_{P}(p^{k+1})}{p^{k+1}}\biggr)+\mathop{\sum }_{k\geqslant 0}g(p^{k})\biggl(\frac{\unicode[STIX]{x1D714}_{Q}(p^{k})}{p^{k}}-\frac{\unicode[STIX]{x1D714}_{Q}(p^{k+1})}{p^{k+1}}\biggr)-1\biggr)+O\biggl(\frac{\log x}{x\log p}\biggr).\nonumber\end{eqnarray}$$

Proof. We first suppose that $p\nmid \text{res}(P,Q)$ . In this case we have

$$\begin{eqnarray}\displaystyle & & \displaystyle \displaystyle \frac{1}{x}\mathop{\sum }_{n\leqslant x}f_{p}(P(n))g_{p}(Q(n))\nonumber\\ \displaystyle & & \displaystyle \displaystyle \quad =\frac{1}{x}\biggl(\mathop{\sum }_{\substack{ p^{k}\leqslant x, \\ p^{k}\Vert P(n)}}f(p^{k})+\mathop{\sum }_{\substack{ p^{l}\leqslant x, \\ p^{l}\Vert Q(n)}}g(p^{l})+\mathop{\sum }_{\substack{ n\leqslant x, \\ p^{0}\Vert P(n)Q(n)}}1\biggr)\nonumber\\ \displaystyle & & \displaystyle \displaystyle \quad =\biggl(\mathop{\sum }_{k\geqslant 0}f(p^{k})\biggl(\frac{\unicode[STIX]{x1D714}_{P}(p^{k})}{p^{k}}-\frac{\unicode[STIX]{x1D714}_{P}(p^{k+1})}{p^{k+1}}\biggr)+\mathop{\sum }_{k\geqslant 0}g(p^{k})\biggl(\frac{\unicode[STIX]{x1D714}_{Q}(p^{k})}{p^{k}}-\frac{\unicode[STIX]{x1D714}_{Q}(p^{k+1})}{p^{k+1}}\biggr)-1\biggr)+O\biggl(\frac{\log x}{x\log p}\biggr).\nonumber\end{eqnarray}$$

More generally,

$$\begin{eqnarray}\displaystyle \frac{1}{x}\mathop{\sum }_{n\leqslant x}f_{p}(P(n))g_{p}(Q(n))=\frac{1}{x}\mathop{\sum }_{\substack{ p^{k},p^{l}\leqslant x, \\ p^{k}\Vert P(n), \\ p^{l}\Vert Q(n)}}f(p^{k})g(p^{l})=\mathop{\sum }_{\substack{ p^{k},p^{l}\geqslant 1}}f(p^{k})g(p^{l})\unicode[STIX]{x1D714}(p^{k},p^{l})+O\biggl(\frac{\log x}{x\log p}\biggr). & & \displaystyle \nonumber\end{eqnarray}$$

This completes the proof of the lemma. ◻

Theorem 1.3 . Let $f,g:\mathbb{N}\rightarrow \mathbb{U}$ be multiplicative functions. Let $P,Q\in \mathbb{Z}[x]$ be two polynomials such that $\text{res}(P,Q)\neq 0$ . Then

$$\begin{eqnarray}\frac{1}{x}\mathop{\sum }_{n\leqslant x}f(P(n))g(Q(n))=\mathop{\prod }_{p\leqslant x}M_{p}(f(P),g(Q))+\text{Error}(f(P),g(Q),x),\end{eqnarray}$$

where

$$\begin{eqnarray}\text{Error}(f(P),g(Q),x)\ll \mathbb{D}_{P}(1,f;\log x;x)+\mathbb{D}_{Q}(1,g;\log x;x)+\frac{1}{\log \log x}.\end{eqnarray}$$

Proof. Choose $y=(1-\unicode[STIX]{x1D700})\log x$ . We begin by decomposing $f(n)=f_{s}(n)f_{l}(n)$ , where

$$\begin{eqnarray}f_{s}(p^{k})=\left\{\begin{array}{@{}ll@{}}f(p^{k})\quad & \text{if }p^{k}\leqslant y,\\ 1\quad & \text{if }p^{k}>y\end{array}\right.\end{eqnarray}$$

and

$$\begin{eqnarray}f_{l}(p^{k})=\left\{\begin{array}{@{}ll@{}}1\quad & \text{if }p^{k}\leqslant y,\\ f(p^{k})\quad & \text{if }p^{k}>y.\end{array}\right.\end{eqnarray}$$

By analogy, we write $g(n)=g_{s}(n)g_{l}(n)$ . We apply Corollary 2.4 to get

$$\begin{eqnarray}\displaystyle \mathop{\sum }_{n\geqslant 1}f_{l}(P(n))f_{s}(P(n))g(Q(n)) & = & \displaystyle \mathfrak{P}(f_{l};P;x)\mathop{\sum }_{n\leqslant x}f_{s}(P(n))g(Q(n))\nonumber\\ \displaystyle & & \displaystyle +\,O\biggl(x\mathbb{D}_{P}^{\ast }(1,f_{l};y;x)+\frac{x(\log \log x)^{3/2}}{\sqrt{\log x}}\biggr).\nonumber\end{eqnarray}$$

We now apply Corollary 2.4 to the inner sum to arrive at

$$\begin{eqnarray}\displaystyle \mathop{\sum }_{n\leqslant x}g_{l}(Q(n))g_{s}(Q(n))f_{s}(P(n)) & = & \displaystyle \mathfrak{P}(g_{l};Q;x)\mathop{\sum }_{n\leqslant x}f_{s}(P(n))g_{s}(Q(n))\nonumber\\ \displaystyle & & \displaystyle +\,O\biggl(x\mathbb{D}_{P}^{\ast }(1,f_{l};y;x)+x\mathbb{D}_{Q}^{\ast }(1,g_{l};y;x)+\frac{x(\log \log x)^{3/2}}{\sqrt{\log x}}\biggr).\nonumber\end{eqnarray}$$

Combining the last two identities, we conclude that

$$\begin{eqnarray}\displaystyle \mathop{\sum }_{n\leqslant x}f(P(n))g(Q(n)) & = & \displaystyle \mathfrak{P}(f_{l};P;x)\mathfrak{P}(g_{l};Q;x)\mathop{\sum }_{n\leqslant x}f_{s}(P(n))g_{s}(Q(n))\nonumber\\ \displaystyle & & \displaystyle +\,O\biggl(x\mathbb{D}_{P}^{\ast }(1,f_{l};y;x)+x\mathbb{D}_{Q}^{\ast }(1,g_{l};y;x)+\frac{x(\log \log x)^{3/2}}{\sqrt{\log x}}\biggr).\nonumber\end{eqnarray}$$

Let $f_{s}=1\ast \unicode[STIX]{x1D703}_{s}$ and $g_{s}=1\ast \unicode[STIX]{x1D6FE}_{s}$ . Then $\unicode[STIX]{x1D703}_{s}(p^{k})=0$ and $\unicode[STIX]{x1D6FE}_{s}(p^{k})=0$ whenever $p^{k}\geqslant y$ . Since $\prod _{p^{k}\leqslant y}p=e^{y+o(y)}\leqslant x$ as long as $y\leqslant (1-\unicode[STIX]{x1D700})\log x$ , the following sums are supported on the integers $d_{1},d_{2}\leqslant x$ . Hence,

$$\begin{eqnarray}\displaystyle \mathop{\sum }_{n\leqslant x}f_{s}(P(n))g_{s}(Q(n)) & = & \displaystyle \mathop{\sum }_{\substack{ d_{1},d_{2}\leqslant x, \\ p|d_{i}\Rightarrow p\leqslant y}}\unicode[STIX]{x1D703}_{s}(d_{1})\unicode[STIX]{x1D6FE}_{s}(d_{2})\mathop{\sum }_{\substack{ n\leqslant x, \\ d_{1}|P(n), \\ d_{2}|Q(n)}}1\nonumber\\ \displaystyle & = & \displaystyle \mathop{\sum }_{\substack{ d\leqslant x, \\ d|\text{res}(P,Q)}}\mathop{\sum }_{\substack{ d_{1},d_{2}\leqslant x, \\ (d_{1},d_{2})=d, \\ p|d_{i}\Rightarrow p\leqslant y}}\unicode[STIX]{x1D703}_{s}(d_{1})\unicode[STIX]{x1D6FE}_{s}(d_{2})F(d_{1},d_{2})x+O\biggl(x^{\unicode[STIX]{x1D700}}\mathop{\sum }_{d_{1},d_{2}\leqslant x}|\unicode[STIX]{x1D703}_{s}(d_{1})\unicode[STIX]{x1D6FE}_{s}(d_{2})|\biggr)\nonumber\\ \displaystyle & = & \displaystyle \mathop{\sum }_{\substack{ d\leqslant x, \\ d|\text{res}(P,Q)}}\mathop{\sum }_{\substack{ d_{1},d_{2}\geqslant 1, \\ (d_{1},d_{2})=d, \\ p|d_{i}\Rightarrow p\leqslant y}}\unicode[STIX]{x1D703}_{s}(d_{1})\unicode[STIX]{x1D6FE}_{s}(d_{2})F(d_{1},d_{2})x+O\biggl(x^{\unicode[STIX]{x1D700}}\mathop{\sum }_{d_{1},d_{2}\leqslant x}|\unicode[STIX]{x1D703}_{s}(d_{1})\unicode[STIX]{x1D6FE}_{s}(d_{2})|\biggr).\nonumber\end{eqnarray}$$

To estimate the error term, we observe that

(8) $$\begin{eqnarray}\displaystyle \mathop{\sum }_{d_{1},d_{2}\leqslant x}|\unicode[STIX]{x1D703}_{s}(d_{1})\unicode[STIX]{x1D6FE}_{s}(d_{2})| & {\leqslant} & \displaystyle x^{1/2}\biggl(\mathop{\sum }_{d\geqslant 1}\frac{|\unicode[STIX]{x1D703}_{s}(d)|}{d^{1/4}}\biggr)\biggl(\mathop{\sum }_{d\geqslant 1}\frac{|\unicode[STIX]{x1D6FE}_{s}(d)|}{d^{1/4}}\biggr)\nonumber\\ \displaystyle & {\leqslant} & \displaystyle x^{1/2}\biggl(\mathop{\prod }_{p\leqslant y}\biggl(\mathop{\sum }_{k\geqslant 0}\frac{|\unicode[STIX]{x1D703}_{s}(p^{k})|}{p^{k/4}}\biggr)\biggr)\biggl(\mathop{\prod }_{p\leqslant y}\biggl(\mathop{\sum }_{k\geqslant 0}\frac{|\unicode[STIX]{x1D6FE}_{s}(p^{k})|}{p^{k/4}}\biggr)\biggr)\nonumber\\ \displaystyle & \ll & \displaystyle x^{1/2}\biggl(\mathop{\prod }_{p\leqslant y}\biggl(1+\frac{2}{p^{1/4}}\biggr)\biggr)^{2}\ll x^{1/2}\exp \biggl(\frac{3y^{3/4}}{\log y}\biggr).\end{eqnarray}$$

The last sum is $O(x^{1/2+\unicode[STIX]{x1D700}})$ for $y\ll \log x$ and $y\rightarrow \infty$ . It easy to see that for $p\leqslant y$ , Lemma 2.5 implies that

$$\begin{eqnarray}M_{p}(f,g)=\mathop{\sum }_{p^{k},p^{l}\geqslant 1}\unicode[STIX]{x1D703}(p^{k})\unicode[STIX]{x1D6FE}(p^{l})F(p^{k},p^{l}),\end{eqnarray}$$

where $M_{p}(f,g)$ is defined as in (3). By multiplicativity, the contribution of small primes is

(9) $$\begin{eqnarray}\displaystyle \mathop{\sum }_{\substack{ d|\text{res}(P,Q)}}\mathop{\sum }_{\substack{ d_{1},d_{2}\geqslant 1, \\ (d_{1},d_{2})=d, \\ p|d_{i}\Rightarrow p\leqslant y}}\unicode[STIX]{x1D703}_{s}(d_{1})\unicode[STIX]{x1D6FE}_{s}(d_{2})F(d_{1},d_{2})=\mathop{\prod }_{p\leqslant y}M_{p}(f,g). & & \displaystyle\end{eqnarray}$$

We are left to estimate $\mathfrak{P}(f_{l};P;x)\mathfrak{P}(g_{l};Q;x)$ . The contribution of primes $p^{k}>y$ and $p\leqslant y$ is

$$\begin{eqnarray}\displaystyle & & \displaystyle \displaystyle \mathop{\prod }_{\substack{ p^{k}\geqslant y, \\ p<y}}\biggl(1+\mathop{\sum }_{i\geqslant k}\frac{\unicode[STIX]{x1D703}_{l}(p^{k})\unicode[STIX]{x1D714}_{P}(p^{k})}{p^{k}}\biggr)\mathop{\prod }_{\substack{ p^{k}\geqslant y, \\ p<y}}\biggl(1+\mathop{\sum }_{i\geqslant k}\frac{\unicode[STIX]{x1D6FE}_{l}(p^{k})\unicode[STIX]{x1D714}_{Q}(p^{k})}{p^{k}}\biggr)\nonumber\\ \displaystyle & & \displaystyle \displaystyle \quad =1+O\biggl(\mathop{\sum }_{\substack{ p^{k}\geqslant y \\ p<y}}\frac{1}{p^{k}}\biggr)=1+O\biggl(\frac{1}{y}\cdot \frac{y}{\log y}\biggr)=1+O\biggl(\frac{1}{\log y}\biggr).\nonumber\end{eqnarray}$$

Furthermore, for $p\geqslant y$ , we clearly have $(p,\text{res}(P,Q))=1$ and

$$\begin{eqnarray}\displaystyle & & \displaystyle \mathfrak{P}(f_{l};P;x)\mathfrak{P}(g_{l};Q;x)\nonumber\\ \displaystyle & & \displaystyle \displaystyle \quad =\biggl(1+O\biggl(\frac{1}{\log y}\biggr)\biggr)\cdot \mathop{\prod }_{y<p\leqslant x}\biggl(1+\mathop{\sum }_{k\geqslant 1}\frac{\unicode[STIX]{x1D703}_{l}(p^{k})\unicode[STIX]{x1D714}_{P}(p^{k})}{p^{k}}\biggr)\mathop{\prod }_{y<p\leqslant x}\biggl(1+\mathop{\sum }_{k\geqslant 1}\frac{\unicode[STIX]{x1D6FE}_{l}(p^{k})\unicode[STIX]{x1D714}_{Q}(p^{k})}{p^{k}}\biggr)\nonumber\\ \displaystyle & & \displaystyle \displaystyle \quad =\biggl(1+O\biggl(\frac{1}{\log y}\biggr)\biggr)\nonumber\\ \displaystyle & & \displaystyle \displaystyle \qquad \times \mathop{\prod }_{y<p\leqslant x}\biggl(1+\mathop{\sum }_{k\geqslant 1}\frac{\unicode[STIX]{x1D703}(p^{k})\unicode[STIX]{x1D714}_{P}(p^{k})}{p^{k}}+\mathop{\sum }_{k\geqslant 1}\frac{\unicode[STIX]{x1D6FE}(p^{k})\unicode[STIX]{x1D714}_{Q}(p^{k})}{p^{k}}+\mathop{\sum }_{k\geqslant 1}\frac{\unicode[STIX]{x1D703}(p^{k})\unicode[STIX]{x1D714}_{P}(p^{k})}{p^{k}}\mathop{\sum }_{k\geqslant 1}\frac{\unicode[STIX]{x1D6FE}(p^{k})\unicode[STIX]{x1D714}_{Q}(p^{k})}{p^{k}}\biggr)\nonumber\\ \displaystyle & & \displaystyle \displaystyle \quad =\biggl(1+O\biggl(\frac{1}{\log y}\biggr)\biggr)\exp \biggl(O\biggl(\mathop{\sum }_{y\leqslant p\leqslant x}\frac{1}{p^{2}}\biggr)\biggr)\mathop{\prod }_{y<p\leqslant x}\biggl(1+\mathop{\sum }_{k\geqslant 1}\frac{\unicode[STIX]{x1D703}(p^{k})\unicode[STIX]{x1D714}_{P}(p^{k})}{p^{k}}+\mathop{\sum }_{k\geqslant 1}\frac{\unicode[STIX]{x1D6FE}(p^{k})\unicode[STIX]{x1D714}_{Q}(p^{k})}{p^{k}}\biggr)\nonumber\\ \displaystyle & & \displaystyle \displaystyle \quad =\biggl(1+O\biggl(\frac{1}{\log y}\biggr)\biggr)\mathop{\prod }_{y<p\leqslant x}\biggl(1+\mathop{\sum }_{k\geqslant 1}\frac{\unicode[STIX]{x1D703}(p^{k})\unicode[STIX]{x1D714}_{P}(p^{k})}{p^{k}}+\mathop{\sum }_{k\geqslant 1}\frac{\unicode[STIX]{x1D6FE}(p^{k})\unicode[STIX]{x1D714}_{Q}(p^{k})}{p^{k}}\biggr)\nonumber\end{eqnarray}$$

and thus

$$\begin{eqnarray}\mathfrak{P}(f_{l};P;x)\mathfrak{P}(g_{l};Q;x)=\mathop{\prod }_{p\geqslant y}M_{p}(f,g)+O\biggl(\frac{1}{\log y}\biggr).\end{eqnarray}$$

We note that $D_{P}^{\ast }(1,f;\log x;x)$ can be replaced with $D_{P}(1,f;\log x;x)$ at a cost of $O(\log \log x/\log x)$ . Combining all of the above, we arrive at the result claimed.◻

Applying Theorem 1.3 and Lemma 2.5 with $g=1$ , we deduce the following corollary.

Corollary 1.2 . Let $f:\mathbb{N}\rightarrow \mathbb{U}$ be a multiplicative function and $P\in \mathbb{Z}[x]$ . Then

$$\begin{eqnarray}\frac{1}{x}\mathop{\sum }_{n\leqslant x}f(P(n))=\mathop{\prod }_{p\leqslant x}M_{p}(f(P))+O\biggl(\mathbb{D}_{P}(1,f;\log x;x)+\frac{1}{\log \log x}\biggr).\end{eqnarray}$$

3 Corollaries required for further applications

To state some corollaries required for our future applications, we introduce a few notations. We fix two integer numbers $a,b\geqslant 1$ . For multiplicative functions $f,g:\mathbb{N}\rightarrow \mathbb{C}$ such that $\mathbb{D}(1,f;\infty )<\infty$ , $\mathbb{D}(1,g;\infty )<\infty$ , we set $f=1\ast \unicode[STIX]{x1D703}$ , $g=1\ast \unicode[STIX]{x1D6FE}$ . For $(r,(a,b))=1$ , we define

(10) $$\begin{eqnarray}G(f;g;r;x)=G(r,x):=\mathop{\prod }_{p^{k}\Vert r,~p\leqslant x}\biggl(\unicode[STIX]{x1D703}(p^{k})\unicode[STIX]{x1D6FE}(p^{k})+\unicode[STIX]{x1D6FF}_{b}\mathop{\sum }_{i>k}\frac{\unicode[STIX]{x1D703}(p^{k})\unicode[STIX]{x1D6FE}(p^{i})}{p^{i-k}}+\unicode[STIX]{x1D6FF}_{a}\mathop{\sum }_{i>k}\frac{\unicode[STIX]{x1D6FE}(p^{k})\unicode[STIX]{x1D703}(p^{i})}{p^{i-k}}\biggr)\end{eqnarray}$$

and $\unicode[STIX]{x1D6FF}_{l}=0$ when $p|l$ and $\unicode[STIX]{x1D6FF}_{l}=1$ otherwise. We remark that in (10), we allow $k=0$ if $p\nmid r$ . For $(r,(a,b))>1$ , we set

$$\begin{eqnarray}G(r,x):=0.\end{eqnarray}$$

We can now deduce the following corollary.

Corollary 3.1. Let $f,g:\mathbb{N}\rightarrow \mathbb{U}$ be multiplicative functions. Suppose that $\mathbb{D}(1,f;\infty )<\infty$ , $\mathbb{D}(1,g;\infty )<\infty$ . Let $a,b\geqslant 1$ ,  $c,d$ be integers with $(a,c)=(b,d)=1$ and $ad\neq bc$ . Then

$$\begin{eqnarray}\displaystyle \frac{1}{x}\mathop{\sum }_{n\leqslant x}f(an+c)g(bn+d)=\mathop{\sum }_{r|ad-bc}\frac{G(f;g;r;x)}{r}+o(1) & & \displaystyle \nonumber\end{eqnarray}$$

when $x\rightarrow \infty$ and the error term $o(1)$ depends on the coefficients $a,b,c,d$ .

Proof. We note that

$$\begin{eqnarray}|\{n\leqslant x\mid \exists p^{k}\geqslant x,p^{k}|an+c\}|\ll \frac{x}{\log x}\end{eqnarray}$$

and thus the contribution of terms with large prime power factors can be absorbed into the error term. We can now apply Theorem 1.3 (using the same notations) with $P(n)=an+c$ and $Q(n)=bn+d$ and note that $\text{res}(P,Q)=ad-bc$ , $\unicode[STIX]{x1D714}_{P}(p^{k})=1$ for $p\nmid a$ and $\unicode[STIX]{x1D714}_{P}(p^{k})=0$ for $p|a$ , $\unicode[STIX]{x1D714}_{Q}(p^{k})=1$ for $p\nmid b$ and $\unicode[STIX]{x1D714}_{Q}(p^{k})=0$ for $p|b$ , $p^{k}\leqslant x$ . We are left to note that

$$\begin{eqnarray}F(d_{1},d_{2})=\frac{1}{[d_{1},d_{2}]}\end{eqnarray}$$

and the terms coming from small primes $p\leqslant y$ , such that $(r,(a,b))=1$

$$\begin{eqnarray}G_{s}(r)=\mathop{\sum }_{\substack{ d_{1},d_{2}\geqslant 1 \\ (d_{1},d_{2})=r \\ (d_{1},a)=1 \\ (d_{2},b)=1 \\ p|rd_{i}\Rightarrow p\leqslant y}}\frac{\unicode[STIX]{x1D703}_{s}(d_{1})\overline{\unicode[STIX]{x1D6FE}_{s}(d_{2})}}{[d_{1},d_{2}]},\end{eqnarray}$$

each has an Euler product

$$\begin{eqnarray}\displaystyle G_{s}(a):=\mathop{\prod }_{p^{k}\Vert a,~p\leqslant y}\biggl(\unicode[STIX]{x1D703}(p^{k})\unicode[STIX]{x1D6FE}(p^{k})+\unicode[STIX]{x1D6FF}_{b}\mathop{\sum }_{i>k}\frac{\unicode[STIX]{x1D703}(p^{k})\unicode[STIX]{x1D6FE}(p^{i})}{p^{i-k}}+\unicode[STIX]{x1D6FF}_{a}\mathop{\sum }_{i>k}\frac{\unicode[STIX]{x1D6FE}(p^{k})\unicode[STIX]{x1D703}(p^{i})}{p^{i-k}}\biggr) & & \displaystyle \nonumber\end{eqnarray}$$

and $\unicode[STIX]{x1D6FF}_{l}=0$ when $p|l$ and $\unicode[STIX]{x1D6FF}_{l}=1$ otherwise.◻

We will require the following extension of Corollary 3.1 to all ‘pretentious’ functions.

Corollary 1.4 . Let $f,g:\mathbb{N}\rightarrow \mathbb{U}$ be multiplicative functions with $\mathbb{D}(f,n^{it},\infty )$ , $\mathbb{D}(g,n^{iu},\infty )<\infty$ and write $f_{0}(n)=f(n)/n^{it}$ and $g_{0}(n)=g(n)/n^{iu}$ . Let $a,b\geqslant 1$ ,  $c,d$ be integers with $(a,c)=(b,d)=1$ and $ad\neq bc$ . As above we have

$$\begin{eqnarray}\frac{1}{x}\mathop{\sum }_{n\leqslant x}f(an+c)g(bn+d)=M_{i}(f(P),g(Q),x)\mathop{\prod }_{p\leqslant x}M_{p}(f_{0}(P),g_{0}(Q))+o(1)\end{eqnarray}$$

when $x\rightarrow \infty$ and the $o(1)$ term depends on the variables $a,b,c,d,t,u$ .

We have

$$\begin{eqnarray}M_{i}(f(P),g(Q),x)=\frac{a^{it}b^{iu}x^{i(t+u)}}{1+i(t+u)}+o(1)\end{eqnarray}$$

when $x\rightarrow \infty$ and the $o(1)$ term depends on $a,b,t,u$ . If $p|(a,b)$ , then $M_{p}(f_{0}(P),g_{0}(Q))=1$ . If $p\nmid ab(ad-bc)$ , then

$$\begin{eqnarray}M_{p}(f_{0}(P),g_{0}(Q))=M_{p}(f_{0}(P))+M_{p}(g_{0}(Q))-1=1+\biggl(1-\frac{1}{p}\biggr)\biggl(\mathop{\sum }_{j\geqslant 1}\frac{f_{0}(p^{j})}{p^{j}}+\mathop{\sum }_{j\geqslant 1}\frac{g_{0}(p^{j})}{p^{j}}\biggr).\end{eqnarray}$$

In general, if $p\nmid (a,b)$ , we have a more complicated formula

$$\begin{eqnarray}\displaystyle M_{p}(f_{0}(P),g_{0}(Q))=\mathop{\sum }_{\substack{ 0\leqslant i\leqslant k, \\ k\geqslant 0, \\ p^{k}\Vert ad-bc}}\biggl(\frac{\unicode[STIX]{x1D703}(p^{i})\unicode[STIX]{x1D6FE}(p^{i})}{p^{i}}+\unicode[STIX]{x1D6FF}_{b}\mathop{\sum }_{j>i}\frac{\unicode[STIX]{x1D703}(p^{i})\unicode[STIX]{x1D6FE}(p^{j})}{p^{j}}+\unicode[STIX]{x1D6FF}_{a}\mathop{\sum }_{j>i}\frac{\unicode[STIX]{x1D6FE}(p^{i})\unicode[STIX]{x1D703}(p^{j})}{p^{j}}\biggr) & & \displaystyle \nonumber\end{eqnarray}$$

and $\unicode[STIX]{x1D6FF}_{l}=0$ when $p|l$ and $\unicode[STIX]{x1D6FF}_{l}=1$ otherwise. Here, $f_{0}=1\ast \unicode[STIX]{x1D703}$ and $g_{0}=1\ast \unicode[STIX]{x1D6FE}$ .

Proof. We observe that $\mathbb{D}(f_{0},1,\infty )<\infty$ and $\mathbb{D}(g_{0},1,\infty )<\infty$ and let

$$\begin{eqnarray}M(x)=\mathop{\sum }_{n\leqslant x}f_{0}(an+c)g_{0}(bn+d).\end{eqnarray}$$

Corollary 3.1 implies that

$$\begin{eqnarray}M(y)=y\mathop{\sum }_{r|ad-bc}\frac{G(f_{0};g_{0};r;y)}{d}+o(y).\end{eqnarray}$$

Recall that for any $r\geqslant 1$ , $(r,(a,b))=1$ ,

$$\begin{eqnarray}\displaystyle G(f_{0};g_{0};r;x)=G(r,x):=\mathop{\prod }_{p^{k}\Vert r,~p\leqslant x}\biggl(\unicode[STIX]{x1D703}(p^{k})\unicode[STIX]{x1D6FE}(p^{k})+\unicode[STIX]{x1D6FF}_{b}\mathop{\sum }_{i>k}\frac{\unicode[STIX]{x1D703}(p^{k})\unicode[STIX]{x1D6FE}(p^{i})}{p^{i-k}}+\unicode[STIX]{x1D6FF}_{a}\mathop{\sum }_{i>k}\frac{\unicode[STIX]{x1D6FE}(p^{k})\unicode[STIX]{x1D703}(p^{i})}{p^{i-k}}\biggr). & & \displaystyle \nonumber\end{eqnarray}$$

Note that $\mathbb{D}(1,f_{0},\infty )<\infty$ together with the fact that $\text{Re}(\unicode[STIX]{x1D703}(p))\leqslant 0$ implies that

$$\begin{eqnarray}-\mathop{\sum }_{p\geqslant 1}\frac{\text{Re}(\unicode[STIX]{x1D703}(p))}{p}<\infty\end{eqnarray}$$

and thus for $y\gg r$ we have

$$\begin{eqnarray}G(r,y)\ll \exp \biggl(\mathop{\sum }_{p\geqslant 1}\frac{\text{Re}(\unicode[STIX]{x1D703}(p))}{p}+\frac{\text{Re}(\unicode[STIX]{x1D6FE}(p))}{p}\biggr)=O(1).\end{eqnarray}$$

Furthermore, since $\text{Re}(\unicode[STIX]{x1D703}(p))/p\leqslant 0$ and $\text{Re}(\unicode[STIX]{x1D6FE}(p))/p\leqslant 0$ , we use (7) to estimate

(11) $$\begin{eqnarray}\displaystyle G(r,x)-G(r,y) & = & \displaystyle G(r,y)\biggl[\mathop{\prod }_{y<p\leqslant x}\biggl(1+\mathop{\sum }_{k\geqslant 1}\frac{\unicode[STIX]{x1D703}(p^{k})}{p^{k}}+\mathop{\sum }_{k\geqslant 1}\frac{\unicode[STIX]{x1D6FE}(p^{k})}{p^{k}}\biggr)-1\biggr]\nonumber\\ \displaystyle & = & \displaystyle G(r,y)\biggl[\exp \biggl(\log \mathop{\sum }_{y<p\leqslant x}\biggl(1+\mathop{\sum }_{k\geqslant 1}\frac{\unicode[STIX]{x1D703}(p^{k})}{p^{k}}+\mathop{\sum }_{k\geqslant 1}\frac{\unicode[STIX]{x1D6FE}(p^{k})}{p^{k}}\biggr)\biggr)-1\biggr]\nonumber\\ \displaystyle & \ll & \displaystyle \biggl|\exp \biggl(\mathop{\sum }_{y\leqslant p\leqslant x}\frac{\text{Re}(\unicode[STIX]{x1D703}(p))}{p}+\frac{\text{Re}(\unicode[STIX]{x1D6FE}(p))}{p}\biggr)\biggl(1+O\biggl(\frac{1}{y}\biggr)\biggr)-1\biggr|\nonumber\\ \displaystyle & \ll & \displaystyle \biggl(\mathop{\sum }_{y<p\leqslant x}\frac{1}{p}\biggr)\ll \log \biggl(\frac{\log x}{\log y}\biggr).\end{eqnarray}$$

For $(r,(a,b))>1$ , we have $G(r,x)=G(r,y)=0$ and (11) holds. Hence,

$$\begin{eqnarray}\mathop{\sum }_{r|ad-bc}\frac{G(r,y)}{r}=\mathop{\sum }_{r|ad-bc}\frac{G(r,x)}{r}+O\biggl(\log \biggl(\frac{\log x}{\log y}\biggr)\biggr).\end{eqnarray}$$

Since

$$\begin{eqnarray}M(y)=y\mathop{\sum }_{r|ad-bc}\frac{G(r,y)}{r}+o(y),\end{eqnarray}$$

we have

$$\begin{eqnarray}\frac{M(y)}{y}=\frac{M(x)}{x}+O\biggl(\log \biggl(\frac{\log x}{\log y}\biggr)\biggr).\end{eqnarray}$$

Summation by parts yields

$$\begin{eqnarray}\displaystyle \mathop{\sum }_{n\leqslant x}f(an+c)g(bn+d) & = & \displaystyle \mathop{\sum }_{n\geqslant 1}(an+c)^{it}(bn+d)^{iu}f_{0}(an+c)g_{0}(bn+d)\nonumber\\ \displaystyle & = & \displaystyle \int _{1}^{x}(ay+c)^{it}(by+d)^{iu}d(M(y))\nonumber\\ \displaystyle & = & \displaystyle M(x)(ax+c)^{it}(bx+d)^{iu}-\int _{1}^{x}M(y)[(ay+c)^{it}(by+d)^{iu}]^{\prime }\,dy\nonumber\\ \displaystyle & = & \displaystyle M(x)(ax+c)^{it}(bx+d)^{iu}-\frac{1}{x}\int _{1}^{x}M(x)y[(ay+c)^{it}(by+d)^{iu}]^{\prime }\,dy\nonumber\\ \displaystyle & & \displaystyle +\,O\biggl(\int _{2}^{x}y\log \biggl(\frac{\log x}{\log y}\biggr)|[(ay+c)^{it}(by+d)^{iu}]^{\prime }|\,dy\biggr)\nonumber\\ \displaystyle & = & \displaystyle \frac{M(x)}{x}\int _{2}^{x}(ay+c)^{it}(by+d)^{iu}\,dy\nonumber\\ \displaystyle & & \displaystyle +\,O\biggl(\int _{2}^{x}y\log \biggl(\frac{\log x}{\log y}\biggr)|[(ay+c)^{it}(by+d)^{itu}]^{\prime }|\,dy\biggr).\nonumber\end{eqnarray}$$

Note that

$$\begin{eqnarray}y|[(ay+c)^{it}(by+d)^{iu}]^{\prime }|\ll \frac{y}{ay+c}+\frac{y}{by+d}=O(1)\end{eqnarray}$$

and so the error term is bounded by

$$\begin{eqnarray}\int _{2}^{x}\log \biggl(\frac{\log x}{\log y}\biggr)\,dy\ll \frac{x}{\log x}=o(x).\end{eqnarray}$$

Since $|(ay+c)^{it}-(ay)^{it}|=O(t/y)$ , we have

$$\begin{eqnarray}\int _{2}^{x}(ay+c)^{it}(by+d)^{iu}\,dy=\int _{2}^{x}(ay)^{it}(by)^{iu}\,dy+o(x).\end{eqnarray}$$

Evaluating the last integral and performing simple manipulations with the Euler factors, we conclude that

$$\begin{eqnarray}\mathop{\sum }_{r|ad-bc}\frac{G(f_{0};g_{0};r;x)}{r}=\mathop{\prod }_{p\leqslant x}M_{p}(f_{0}(P),g_{0}(Q))+o(1)\end{eqnarray}$$

and the result follows. ◻

Remark 3.2. Let $f_{k}(n)$ , $k=\overline{1,m}$ be multiplicative functions such that $|f_{k}(n)|\leqslant 1$ and $\mathbb{D}(f_{k}(n),n^{it_{k}};\infty )<\infty$ for all $n\in \mathbb{N}$ . Following the lines of the proof, one can generalize Corollary 1.4 to compute correlations of the form

$$\begin{eqnarray}\mathop{\sum }_{n\leqslant x}f_{1}(a_{1}n+b_{1})f_{2}(a_{2}n+b_{2})\cdot \cdots \cdot f_{m}(a_{m}n+b_{m}).\end{eqnarray}$$

Finally, we will require the following special case of Corollary 3.1.

Corollary 3.3. Let $f:\mathbb{N}\rightarrow \mathbb{U}$ be a multiplicative function such that $\mathbb{D}(1,f;\infty )<\infty$ , $m\in \mathbb{N}$ . Then

$$\begin{eqnarray}\frac{1}{x}\mathop{\sum }_{n\geqslant 1}f(n)\overline{f(n+m)}=\mathop{\sum }_{r|m}\frac{G_{0}(r)}{r}+o(1)\end{eqnarray}$$

when $x\rightarrow \infty$ and $o(1)$ depends on $m$ , where $f=1\ast \unicode[STIX]{x1D703}$ and

$$\begin{eqnarray}G_{0}(r):=\mathop{\prod }_{p^{k}||r}\biggl(|\unicode[STIX]{x1D703}(p^{k})|^{2}+2\mathop{\sum }_{i>k}\frac{\text{Re}(\unicode[STIX]{x1D703}(p^{k})\overline{\unicode[STIX]{x1D703}(p^{i})})}{p^{i-k}}\biggr).\end{eqnarray}$$

Proof. We apply Corollary 3.1 with $g=\overline{f}$ , $a=b=1$ , $d=0$ and $c=m$ and observe that

$$\begin{eqnarray}\displaystyle \mathop{\prod }_{p>x}\biggl(|\unicode[STIX]{x1D703}(p^{k})|^{2}+2\mathop{\sum }_{i>k}\frac{\text{Re}(\unicode[STIX]{x1D703}(p^{k})\overline{\unicode[STIX]{x1D703}(p^{i})})}{p^{i-k}}\biggr)=\mathop{\prod }_{p>x}\biggl(1+2\mathop{\sum }_{i\geqslant 1}\frac{\text{Re}(\overline{\unicode[STIX]{x1D703}(p^{i})})}{p^{i}}\biggr)\rightarrow 1. & & \displaystyle \nonumber\end{eqnarray}$$

Hence, the Euler factors

$$\begin{eqnarray}G(a):=\mathop{\prod }_{p^{k}||a,~p\leqslant x}\biggl(|\unicode[STIX]{x1D703}(p^{k})|^{2}+2\mathop{\sum }_{i>k}\frac{\text{Re}(\unicode[STIX]{x1D703}(p^{k})\overline{\unicode[STIX]{x1D703}(p^{i})})}{p^{i-k}}\biggr)\end{eqnarray}$$

converge to

$$\begin{eqnarray}G_{0}(a):=\mathop{\prod }_{p^{k}||a}\biggl(|\unicode[STIX]{x1D703}(p^{k})|^{2}+2\mathop{\sum }_{i>k}\frac{\text{Re}(\unicode[STIX]{x1D703}(p^{k})\overline{\unicode[STIX]{x1D703}(p^{i})})}{p^{i-k}}\biggr).\square\end{eqnarray}$$

Let $f$ be a multiplicative function such that $|f(n)|\leqslant 1$ and $\mathbb{D}(f(n),n^{it}\unicode[STIX]{x1D712}(n);\infty )<\infty$ for some $t\in \mathbb{R}$ , where $\unicode[STIX]{x1D712}$ is a primitive character of conductor $q$ . We define $F$ to be the multiplicative function such that

(12) $$\begin{eqnarray}F(p^{k})=\left\{\begin{array}{@{}ll@{}}f(p^{k})\overline{\unicode[STIX]{x1D712}(p^{k})}p^{-ikt}\quad & \text{if }p\nmid q,\\ 1\quad & \text{if }p\mid q\end{array}\right.\end{eqnarray}$$

and

$$\begin{eqnarray}M_{p}(F,\overline{F};d)=\lim _{x\rightarrow \infty }\frac{1}{x}\mathop{\sum }_{n\leqslant x}F_{p}(n)\overline{F_{p}(n+d)}.\end{eqnarray}$$

We are now ready to establish the formula for correlations when $f$ ‘pretends’ to be a modulated character.

Theorem 1.5 . Let $f:\mathbb{N}\rightarrow \mathbb{U}$ be a multiplicative function such that $\mathbb{D}(f(n),n^{it}\unicode[STIX]{x1D712}(n);\infty )<\infty$ for some $t\in \mathbb{R}$ and $\unicode[STIX]{x1D712}$ be a primitive character of conductor $q$ . Then, for any non-zero integer $d$ , we have

$$\begin{eqnarray}\frac{1}{x}\mathop{\sum }_{n\leqslant x}f(n)\overline{f(n+d)}=\mathop{\prod }_{\substack{ p\leqslant x \\ p\nmid q}}M_{p}(F,\overline{F};d)\mathop{\prod }_{p^{l}\Vert q}M_{p^{l}}(f,\overline{f},d)+o(1)\end{eqnarray}$$

when $x\rightarrow \infty$ . Here, the $o(1)$ term depends on $d,\unicode[STIX]{x1D712},t$ and

$$\begin{eqnarray}M_{p^{l}}(f,\overline{f},d)=\left\{\begin{array}{@{}ll@{}}0\quad & \text{if }p^{l-1}\nmid d,\\ \displaystyle 1-\frac{1}{p}\quad & \text{if }p^{l-1}\Vert d,\\ \displaystyle \biggl(1-\frac{1}{p}\biggr)\mathop{\sum }_{j=0}^{k}\frac{|f(p^{j})|^{2}}{p^{j}}-\frac{|f(p^{k})|^{2}}{p^{k}}\quad & \text{if }p^{l+k}\Vert d\end{array}\right.\end{eqnarray}$$

for any $k\geqslant 0$ and, if $p^{n}\Vert d$ for some $n\geqslant 0$ , then

$$\begin{eqnarray}M_{p}(F,\overline{F},d)=1-\frac{2}{p^{n+1}}+\biggl(1-\frac{1}{p}\biggr)\mathop{\sum }_{j>n}\biggl(\frac{F(p^{n})\overline{F(p^{j})}}{p^{j}}+\frac{\overline{F(p^{n})}F(p^{j})}{p^{j}}\biggr).\end{eqnarray}$$

In particular, the mean value is $o(1)$ if $q\nmid d\prod _{p|q}p$ .

Proof. We partition the sum according to $r,s\geqslant 1$ such that $r|n$ and $\text{rad}(r)|q$ , $(n/r,q)=1$ and $s|(n+d)$ and $\text{rad}(s)|q$ , $((n+d)/s,q)=1$ . Note that $(r,s)|d$ . We write

$$\begin{eqnarray}n=m\cdot \text{lcm}(r,s)+rb(r)\end{eqnarray}$$

such that $sb(s)-rb(r)=d$ for some integers $b(r),b(s)$ . The sum can now be rewritten as

$$\begin{eqnarray}\mathop{\sum }_{n\leqslant x}f(n)\overline{f(n+d)}=\mathop{\sum }_{r,s}f(r)\overline{f(s)}\mathop{\sum }_{\substack{ m^{\ast }\leqslant x/\text{lcm}(r,s) \\ }}f\biggl(m^{\ast }\frac{s}{(r,s)}+b(r)\biggr)\overline{f\biggl(m^{\ast }\frac{r}{(r,s)}+b(s)\biggr)},\end{eqnarray}$$

where the inner sum runs over $m^{\ast }$ such that

$$\begin{eqnarray}\biggl(m^{\ast }\frac{s}{(r,s)}+b(r),q\biggr)=1\end{eqnarray}$$

and

$$\begin{eqnarray}\biggl(m^{\ast }\frac{r}{(r,s)}+b(s),q\biggr)=1.\end{eqnarray}$$

We can therefore define the function $f_{1}$ such that $f_{1}(p^{k})=f(p^{k})$ for all primes $p\nmid q$ and $f_{1}(p^{k})=0$ otherwise. In this case Corollary 1.4 implies that

(13) $$\begin{eqnarray}\displaystyle & & \displaystyle \displaystyle \mathop{\sum }_{\substack{ m^{\ast }\leqslant x/\text{lcm}(r,s) \\ }}f\biggl(m^{\ast }\frac{s}{(r,s)}+b(r)\biggr)\overline{f\biggl(m^{\ast }\frac{r}{(r,s)}+b(s)\biggr)}\nonumber\\ \displaystyle & & \displaystyle \displaystyle \quad =\mathop{\sum }_{\substack{ m\leqslant x/\text{lcm}(r,s) \\ }}f_{1}\biggl(m\frac{s}{(r,s)}+b(r)\biggr)\overline{f_{1}\biggl(m\frac{r}{(r,s)}+b(s)\biggr)},\end{eqnarray}$$

where now $m$ runs over all integers up to $x/\text{lcm}(r,s)$ . We can now factor $f_{1}(n)=\unicode[STIX]{x1D712}(n)F(n)$ . Note that $\mathbb{D}(F,1,\infty )<\infty$ . Let $m=kq+a$ , where $a$ runs over residue classes $\text{mod}(q)$ . The sum in (13) can be rewritten as

$$\begin{eqnarray}\displaystyle & & \displaystyle \displaystyle \mathop{\sum }_{r,s}f(r)f(s)\mathop{\sum }_{a\,\text{mod}(q)}\unicode[STIX]{x1D712}\biggl(a\frac{s}{(r,s)}+b(r)\biggr)\overline{\unicode[STIX]{x1D712}\biggl(a\frac{r}{(s,r)}+b(s)\biggr)}\nonumber\\ \displaystyle & & \displaystyle \displaystyle \quad \times \,\mathop{\sum }_{k\leqslant x/q\,\text{lcm}(r,s)}F\biggl(kq\frac{s}{(r,s)}+a\frac{s}{(r,s)}+b(r)\biggr)\overline{F\biggl(kq\frac{r}{(r,s)}+a\frac{r}{(r,s)}+b(s)\biggr)}.\nonumber\end{eqnarray}$$

We apply Corollary 1.4 to the inner sum and observe that

$$\begin{eqnarray}a_{2}b_{1}-a_{1}b_{2}=\frac{dq}{(r,s)}\end{eqnarray}$$

and the asymptotic in Corollary 1.4 does not depend on $b_{1},b_{2}$ and consequently on the residue class $a~(\text{mod}(q))$ . Hence, up to a small error, the innermost sum is equal to

$$\begin{eqnarray}\mathop{\sum }_{m\leqslant x/q[s,r]}F\biggl(m\frac{s}{(r,s)}+b(r)\biggr)\overline{F\biggl(m\frac{r}{(r,s)}+b(s)\biggr)}.\end{eqnarray}$$

We now focus on the sum

(14) $$\begin{eqnarray}\mathop{\sum }_{a\,\text{mod}(q)}\unicode[STIX]{x1D712}\biggl(a\frac{s}{(r,s)}+b(r)\biggr)\overline{\unicode[STIX]{x1D712}\biggl(a\frac{r}{(s,r)}+b(s)\biggr)}.\end{eqnarray}$$

Let $q=p_{1}^{a_{1}}p_{2}^{a_{2}}\cdots p_{k}^{a_{k}}$ and $\unicode[STIX]{x1D712}=\unicode[STIX]{x1D712}_{p_{1}^{a_{1}}}\unicode[STIX]{x1D712}_{p^{a_{2}}}\cdot \cdots \cdot \unicode[STIX]{x1D712}_{p_{k}^{a_{k}}}$ , where each $\unicode[STIX]{x1D712}_{p_{i}^{a_{i}}}$ is a primitive character of conductor $p_{i}^{a_{i}}$ . By the Chinese remainder theorem, the sum (14) equals

$$\begin{eqnarray}\displaystyle & & \displaystyle \displaystyle \mathop{\sum }_{a\,\text{mod}(q)}\unicode[STIX]{x1D712}\biggl(a\frac{s}{(r,s)}+b(r)\biggr)\overline{\unicode[STIX]{x1D712}\biggl(a\frac{r}{(s,r)}+b(s)\biggr)}\nonumber\\ \displaystyle & & \displaystyle \displaystyle \quad =\mathop{\prod }_{p^{k}\Vert q}\mathop{\sum }_{a\,\text{mod}(p^{k})}\unicode[STIX]{x1D712}_{p^{k}}\biggl(a\frac{s}{(r,s)}+b(r)\biggr)\overline{\unicode[STIX]{x1D712}_{p^{k}}\biggl(a\frac{r}{(s,r)}+b(s)\biggr)}.\nonumber\end{eqnarray}$$

We claim that the last sum is zero unless $r=s$ . Indeed, if $r\neq s$ , then there exists a prime $p$ such that $p^{i}\Vert r$ and $p^{j}\Vert s$ for $j>i$ . Since $(r/(r,s),p)=1$ , we can make a change of variables

$$\begin{eqnarray}a\rightarrow \frac{ar}{(r,s)}~(\text{mod}(p^{k}))\end{eqnarray}$$

and the $p\text{th}$ factor can be rewritten as

$$\begin{eqnarray}\mathop{\sum }_{a\,\text{mod}(p^{k})}\unicode[STIX]{x1D712}_{p^{k}}(ap^{j-i}t+b_{1}(r))\overline{\unicode[STIX]{x1D712}_{p^{k}}(a+b_{1}(s))},\end{eqnarray}$$

where $(t,p)=1$ . If $j-i\geqslant k$ , then the first term is fixed and the second runs over all residues modulo $p^{k}$ . So, the sum is zero. If $j-i<k$ , we write $a=A+p^{k-(j-i)}L$ , where $A$ runs over residues modulo $p^{k-(j-l)}$ and $L$ runs over residues modulo $p^{j-i}$ . Then our sum becomes

$$\begin{eqnarray}\mathop{\sum }_{A\,\text{mod}(p^{k-(j-l)})}\unicode[STIX]{x1D712}_{p^{k}}(Ap^{j-i}t+b_{1}(r))\mathop{\sum }_{L\,\text{mod}\,p^{j-i}}\overline{\unicode[STIX]{x1D712}_{p^{k}}(A+b_{1}(s)+p^{k-j+i}L)}.\end{eqnarray}$$

It is easy to show that the inner sum

$$\begin{eqnarray}\mathop{\sum }_{L\,\text{mod}\,p^{j-i}}\overline{\unicode[STIX]{x1D712}(A+b_{1}(s)+p^{k-j+i}L)}=0.\end{eqnarray}$$

Thus, the main contribution comes from the terms $r=s=R$ . In this case we have $R(b(s)-b(r))=d=bR$ and we can take $b(r)=0$ , $b(s)=b$ . Our character sum can be rewritten as

$$\begin{eqnarray}\mathop{\sum }_{a\,\text{mod}(q)}\unicode[STIX]{x1D712}(a)\overline{\unicode[STIX]{x1D712}(a+b)}.\end{eqnarray}$$

To evaluate the last sum, we split it into prime powers. Now, if $p^{k}\Vert q$ and $p^{i}\Vert b$ (possibly $i=0$ ), then we have a non-zero contribution if and only if $i\geqslant k-1$ . Indeed, let $b=p^{i}b_{1}$ , $(b_{1},p)=1$ . We note that

$$\begin{eqnarray}\mathop{\sum }_{a\,\text{mod}(p^{k})}\unicode[STIX]{x1D712}_{p^{k}}(a)\overline{\unicode[STIX]{x1D712}_{p^{k}}(a+b)}=\mathop{\sum }_{\substack{ c\,\text{mod}(p^{k}), \\ (c,p)=1}}\unicode[STIX]{x1D712}_{p^{k}}(p^{i}c+1).\end{eqnarray}$$

This sum is $0$ if $i\leqslant k-2$ and equals $-p^{k-1}$ whenever $i=k-1$ and $\unicode[STIX]{x1D719}(p^{k})$ whenever $i\geqslant k$ . We thus have

$$\begin{eqnarray}\mathop{\sum }_{a\,\text{mod}(q)}\unicode[STIX]{x1D712}(a)\overline{\unicode[STIX]{x1D712}(a+b)}=\mathop{\prod }_{\substack{ p^{k}\Vert q \\ p^{i}\Vert b \\ i\leqslant k-1}}\unicode[STIX]{x1D707}(p^{k-i})p^{i}\mathop{\prod }_{\substack{ p^{k}\Vert q \\ p^{k}|b}}\unicode[STIX]{x1D719}(p^{k})\end{eqnarray}$$

and the result follows by combining this with Corollary 1.4 and easy manipulations with the Euler products. ◻

Combining the last proposition with Corollary 3.3, we deduce the following result.

Corollary 3.4. Let $f:\mathbb{N}\rightarrow \mathbb{U}$ be a multiplicative function with $\mathbb{D}(f(n),n^{it}\unicode[STIX]{x1D712}(n);\infty )<\infty$ for some primitive character $\unicode[STIX]{x1D712}$ of conductor $q$ . Then

$$\begin{eqnarray}\displaystyle \frac{1}{x}\mathop{\sum }_{n\leqslant x}f(n)\overline{f(n+1)} & = & \displaystyle \frac{\unicode[STIX]{x1D707}(q)}{q}\mathop{\prod }_{\substack{ p\geqslant 1 \\ p\nmid q}}\biggl(2\operatorname{Re}\biggl(1-\frac{1}{p}\biggr)\biggl(\mathop{\sum }_{k\geqslant 0}\frac{f(p^{k})\overline{\unicode[STIX]{x1D712}(p^{k})}p^{-ikt}}{p^{k}}\biggr)-1\biggr)+o(1)\nonumber\end{eqnarray}$$

when $x\rightarrow \infty$ and $o(1)$ depends on $\unicode[STIX]{x1D712},t$ .

We remark that using the same arguments one may establish the formula for the correlations

$$\begin{eqnarray}\mathop{\sum }_{n\leqslant x}f(n)g(n+m)\end{eqnarray}$$

for $\mathbb{D}(f(n),n^{it_{1}}\unicode[STIX]{x1D712}(n),\infty )<\infty$ and $\mathbb{D}(g(n),n^{it_{2}}\unicode[STIX]{x1D713}(n),\infty )<\infty$ . We state here one particular case when $m=1$ .

Proposition 3.5. Let $f,g:\mathbb{N}\rightarrow \mathbb{U}$ be two multiplicative functions with $\mathbb{D}(f(n),n^{it_{1}}\unicode[STIX]{x1D712}(n),\infty )<\infty$ and $\mathbb{D}(g(n),n^{it_{2}}\unicode[STIX]{x1D713}(n),\infty )<\infty$ for some primitive characters $\unicode[STIX]{x1D712},\unicode[STIX]{x1D713}$ . Let $R=q_{\unicode[STIX]{x1D713}}/(q_{\unicode[STIX]{x1D712}},q_{\unicode[STIX]{x1D713}})$ and $S=q_{\unicode[STIX]{x1D712}}/(q_{\unicode[STIX]{x1D712}},q_{\unicode[STIX]{x1D713}})$ , $Q=[q_{\unicode[STIX]{x1D712}},q_{\unicode[STIX]{x1D713}}]$ . Then

$$\begin{eqnarray}\displaystyle \frac{1}{x}\mathop{\sum }_{n\leqslant x}f(n)g(n+1) & = & \displaystyle \frac{R^{it_{1}}S^{it_{2}}}{i(t_{1}+t_{2})+1}f(R)g(S)\mathop{\sum }_{a\,\text{mod}(Q)}\unicode[STIX]{x1D712}(aS+b(R))\unicode[STIX]{x1D713}(aR+b(S))\nonumber\\ \displaystyle & & \displaystyle \times \,\mathop{\prod }_{\substack{ p\leqslant x \\ p\nmid Q}}\biggl(\biggl(1-\frac{1}{p}\biggr)\biggl(\mathop{\sum }_{k\geqslant 0}\frac{f(p^{k})p^{-ikt_{1}}}{p^{k}}\biggr)\nonumber\\ \displaystyle & & \displaystyle +\,\biggl(1-\frac{1}{p}\biggr)\biggl(\mathop{\sum }_{k\geqslant 0}\frac{g(p^{k})p^{-ikt_{2}}}{p^{k}}\biggr)-1\biggr)+o(1)\nonumber\end{eqnarray}$$

when $x\rightarrow \infty$ and $o(1)$ depends on the parameters $t_{1},t_{2},\unicode[STIX]{x1D712},\unicode[STIX]{x1D713}$ .

Proof. We follow the lines of the proof of Proposition 1.5 and note that in this case $(r,s)=1$ and the only term that contributes is

$$\begin{eqnarray}r=R=\frac{q_{\unicode[STIX]{x1D713}}}{(q_{\unicode[STIX]{x1D712}},q_{\unicode[STIX]{x1D713}})}\end{eqnarray}$$

and

$$\begin{eqnarray}s=S=\frac{q_{\unicode[STIX]{x1D712}}}{(q_{\unicode[STIX]{x1D712}},q_{\unicode[STIX]{x1D713}})}.\square\end{eqnarray}$$

4 Application to the Erdős–Coons–Tao conjecture

In this section, we are going to study multiplicative functions $f:\mathbb{N}\rightarrow \mathbb{T}$ such that

(15) $$\begin{eqnarray}\limsup _{x\rightarrow \infty }\biggl|\mathop{\sum }_{n\leqslant x}f(n)\biggr|<\infty .\end{eqnarray}$$

We first focus on the complex-valued case and the proof of Theorem 1.7. The key tool is the following recent result by Tao [Tao16b].

Theorem 4.1 (Tao).

Let $a_{1},a_{2}$ be natural numbers and let $b_{1},b_{2}$ be integers such that $a_{1}b_{2}-a_{2}b_{1}\neq 0$ . Let $\unicode[STIX]{x1D700}>0$ and suppose that $A$ is sufficiently large depending on $\unicode[STIX]{x1D700},a_{1},a_{2},b_{1},b_{2}$ . Let $x\geqslant \unicode[STIX]{x1D714}\geqslant A$ and let $g_{1},g_{2}:\mathbb{N}\rightarrow \mathbb{U}$ be multiplicative functions with $g_{1}$ non-pretentious in the sense that

$$\begin{eqnarray}\mathop{\sum }_{p\leqslant x}\frac{1-\operatorname{Re}(g_{1}(p)\unicode[STIX]{x1D712}(p)p^{it})}{p}\geqslant A\end{eqnarray}$$

for all Dirichlet characters $\unicode[STIX]{x1D712}$ of period at most $A$ and all real numbers $|t|\leqslant Ax$ . Then

$$\begin{eqnarray}\biggl|\mathop{\sum }_{x/\unicode[STIX]{x1D714}<n\leqslant x}\frac{g_{1}(a_{1}n+b_{1})g_{2}(a_{2}n+b_{2})}{n}\biggr|\leqslant \unicode[STIX]{x1D700}\log \unicode[STIX]{x1D714}.\end{eqnarray}$$

We will require the following technical lemma.

Lemma 4.2. Let $a>1$ be given and let $x_{n}$ be an increasing sequence such that $x_{n}<x_{n+1}\leqslant x_{n}^{a}$ . Suppose that for each $x_{m}$ , there exist a primitive character $\unicode[STIX]{x1D712}_{m}$ of conductor $O(1)$ and a real $t_{m}$ with $|t_{m}|\ll x_{m}$ such that $\mathbb{D}(f(n),n^{it_{m}}\unicode[STIX]{x1D712}_{m}(n),x_{m})=O(1)$ . Then there exist $t\in \mathbb{R}$ and a primitive character $\unicode[STIX]{x1D712}$ such that $\mathbb{D}(f(n),n^{it}\unicode[STIX]{x1D712}(n),\infty )<\infty$ .

Proof. Without loss of generality, we may assume that $x_{n+1}=x_{n}^{a}$ (otherwise we can choose a suitable subsequence and modify the values of $a$ if necessary). We note that there exists $k=O(1)$ such that for all $n\geqslant 1$ , $\unicode[STIX]{x1D712}_{n}^{k}(p)=1$ for all but finitely many primes $p$ . The triangle inequality now implies that

$$\begin{eqnarray}\mathbb{D}(f^{k}(n),n^{ikt_{m}},x_{m})=\mathbb{D}(f^{k}(n),n^{ikt_{m}}\unicode[STIX]{x1D712}_{m}^{k}(n),x_{m})+O(1)\geqslant k\mathbb{D}(f(n),n^{it_{m}}\unicode[STIX]{x1D712}_{m}(n),x_{m})=O(1).\end{eqnarray}$$

Moreover,

$$\begin{eqnarray}\mathbb{D}^{2}(f^{k}(n),n^{ikt_{m}},x_{m+1})\leqslant O(1)+\mathop{\sum }_{x_{m}\leqslant p\leqslant x_{m+1}}\frac{2}{p}\leqslant O(1)+2\log \frac{\log x_{m+1}}{\log x_{m}}=O(1)\end{eqnarray}$$

and therefore applying the triangle inequality once again we end up with

$$\begin{eqnarray}\displaystyle O(1)\geqslant \mathbb{D}(f^{k}(n),n^{ikt_{m}},x_{m+1})+\mathbb{D}(f^{k}(n),n^{ikt_{m+1}},x_{m+1})\geqslant \mathbb{D}(1,n^{ik(t_{m+1}-t_{m})},x_{m+1}). & & \displaystyle \nonumber\end{eqnarray}$$

Clearly, $k|t_{m+1}-t_{m}|\ll x_{m+1}$ and therefore by the classical zero-free region we get

$$\begin{eqnarray}|t_{m+1}-t_{m}|\ll \frac{1}{\log x_{m+1}}.\end{eqnarray}$$

Iterating the last inequality, we conclude that there exists $t$ such that

$$\begin{eqnarray}|t_{m}-t|\ll \frac{1}{\log x_{m+1}}\end{eqnarray}$$

for all $m\geqslant 1$ . Since there are only finitely many options of characters $\unicode[STIX]{x1D712}_{m}$ , we can pass to the subsequence and assume that $\unicode[STIX]{x1D712}_{m}=\unicode[STIX]{x1D712}$ is fixed. The triangle inequality now implies that

$$\begin{eqnarray}\mathbb{D}(f(n),n^{it_{m}}\unicode[STIX]{x1D712}(n),x_{m})+\mathbb{D}(1,n^{i(t-t_{m})},x_{m})\geqslant \mathbb{D}(f(n),n^{it}\unicode[STIX]{x1D712}(n),x_{m})+O(1).\end{eqnarray}$$

We are left to note that

$$\begin{eqnarray}\mathbb{D}(1,n^{i(t-t_{m})},x_{m})=O(1)\end{eqnarray}$$

as long as $|t_{m}-t|\ll 1/\text{log}\,x_{m}$ and we can replace $t_{m}$ with $t$ at a cost of $O(1)$ . This completes the proof of the lemma.◻

Lemma 4.3. Suppose that for a multiplicative $f:\mathbb{N}\rightarrow \mathbb{T}$ , (15) holds. Then there exist a primitive character $\unicode[STIX]{x1D712}$ and $t\in \mathbb{R}$ such that $\mathbb{D}(f(n),\unicode[STIX]{x1D712}(n)n^{it},\infty )<\infty$ .

Proof. Let $H\in \mathbb{N}$ . Suppose that for each $1\leqslant h\leqslant H$ , we have

$$\begin{eqnarray}\frac{1}{\log x}\mathop{\sum }_{n\leqslant x}\frac{f(n)\overline{f(n+h)}}{n}\leqslant \frac{1}{2H}.\end{eqnarray}$$

Consider

$$\begin{eqnarray}T(x):=\frac{1}{\log x}\mathop{\sum }_{n\leqslant x}\frac{1}{n}\biggl|\mathop{\sum }_{k=n+1}^{n+H+1}f(k)\biggr|^{2}.\end{eqnarray}$$

Expanding the square, we get

$$\begin{eqnarray}T(x)=\mathop{\sum }_{1\leqslant h_{1}\neq h_{2}\leqslant H}\frac{1}{\log x}\mathop{\sum }_{n\leqslant x}\frac{f(n+h_{1})\overline{f(n+h_{2})}}{n}.\end{eqnarray}$$

The diagonal contribution $h_{1}=h_{2}$ is $1+o(1)$ . For $h_{2}>h_{1}$ , we introduce $h=h_{2}-h_{1}$ and replace $n$ in the denominator by $N=n+h_{1}$ at a cost of $\ll H/\text{log}\,x$ . We change the range for $N$ from $1+h_{1}\leqslant N\leqslant x+h_{1}$ to $1\leqslant n\leqslant x$ at a cost of $\ll \log H/\text{log}\,x$ . Therefore,

$$\begin{eqnarray}\displaystyle T(x) & = & \displaystyle H+o(1)-\mathop{\sum }_{|h|\leqslant H}(H-|h|)\cdot \frac{1}{\log x}\mathop{\sum }_{N\leqslant x}\frac{f(N)\overline{f(N+h)}}{N}\nonumber\\ \displaystyle & {\geqslant} & \displaystyle H-(H^{2}-H)\cdot \frac{1}{2H}+o(1)=\frac{H}{2}+O(1)\nonumber\end{eqnarray}$$

for $x\rightarrow \infty$ . This contradicts (15) for sufficiently large $H\geqslant 1$ . Thus, for a fixed $H\geqslant 1$ and every large $x\gg 1$ , there exists $1\leqslant h_{x}\leqslant H$  such that

$$\begin{eqnarray}\frac{1}{\log x}\mathop{\sum }_{n\leqslant x}\frac{f(n)\overline{f(n+h_{x})}}{n}\gg 1.\end{eqnarray}$$

Since $h_{x}\leqslant H$ , we can apply Theorem 4.1 to conclude that there exists $A=A(H)\geqslant 0$ such that for any sufficiently large $x$ , there exist $t_{x}\in \mathbb{R}$ , $|t_{x}|\leqslant Ax$ and a primitive character $\unicode[STIX]{x1D712}$ of modulus $D\leqslant A$ such that $\mathbb{D}(f(n),n^{it_{x}}\unicode[STIX]{x1D712}(n);x)\leqslant A$ , namely

$$\begin{eqnarray}\mathop{\sum }_{p\leqslant x}\frac{1-\operatorname{Re}(f(p)p^{-it_{x}}\overline{\unicode[STIX]{x1D712}(p)})}{p}\leqslant A^{2}.\end{eqnarray}$$

Since the latter holds uniformly for all large $x$ , Lemma 4.2 implies the result.◻

We now refine the result of Lemma 4.3.

Theorem 1.7 . Suppose for a multiplicative $f:\mathbb{N}\rightarrow \mathbb{T}$ , (15) holds. Then there exist a primitive character $\unicode[STIX]{x1D712}$ of an odd conductor $q$ and $t\in \mathbb{R}$ such that $\mathbb{D}(f(n),\unicode[STIX]{x1D712}(n)n^{it};\infty )<\infty$ and $f(2^{k})=-\unicode[STIX]{x1D712}^{k}(2)2^{-ikt}$ for all $k\geqslant 1$ .

Proof. Applying Lemma 4.3, we can find a primitive character $\unicode[STIX]{x1D712}$ of conductor $q$ and $t\in \mathbb{R}$ such that $\mathbb{D}(f(n),\unicode[STIX]{x1D712}(n)n^{it};\infty )<\infty$ . Theorem 1.5 implies that for any $d\geqslant 0$ , we have

$$\begin{eqnarray}S_{d}=\lim _{x\rightarrow \infty }\frac{1}{x}\mathop{\sum }_{n\leqslant x}f(x)\overline{f(x+d)}=\mathop{\prod }_{\substack{ p\leqslant x \\ p\nmid q}}M_{p}(F,\overline{F};d)\mathop{\prod }_{p^{l}\Vert q}M_{p^{l}}(f,\overline{f},d).\end{eqnarray}$$

For fixed $H\geqslant 1$ , we can now write

$$\begin{eqnarray}\displaystyle \lim _{x\rightarrow \infty }\frac{1}{x}\mathop{\sum }_{n\leqslant x}\biggl|\mathop{\sum }_{k=n+1}^{n+H+1}f(k)\biggr|^{2} & = & \displaystyle \lim _{x\rightarrow \infty }\frac{1}{x}\biggl[\mathop{\sum }_{h=0,~n\leqslant x}Hf(n)\overline{f(n+h)}+2\mathop{\sum }_{1\leqslant h\leqslant H}(H-h)\mathop{\sum }_{n\leqslant x}f(n)\overline{f(n+h)}\biggr]\nonumber\\ \displaystyle & = & \displaystyle HS_{0}+2\mathop{\sum }_{h=1}^{H}(H-h)S_{h}=H+2\mathop{\sum }_{N=1}^{H-1}\mathop{\sum }_{n=1}^{N}S_{m}.\nonumber\end{eqnarray}$$

We note that all $S_{m}\leqslant 1$ and Theorem 1.5 implies that each $S_{m}$ behaves like a scaled multiplicative function, since it is given by the Euler product. We are going to show that there exists $\lim _{N\rightarrow \infty }(1/N)\sum _{n\leqslant N}S_{n}=c$ and so

$$\begin{eqnarray}H+2\mathop{\sum }_{N=1}^{H-1}\mathop{\sum }_{n=1}^{N}S_{m}=O(1)\sim H+2\mathop{\sum }_{N=1}^{H}cn=cH^{2}+O(H).\end{eqnarray}$$

The latter would imply that $c=0$ . We turn to the computations of the corresponding mean values. Clearly,

$$\begin{eqnarray}\lim _{N\rightarrow \infty }\frac{1}{N}\mathop{\sum }_{n\leqslant N}S_{n}=\mathop{\prod }_{p\leqslant N}S(p),\end{eqnarray}$$

where $S(p)$ denotes the local factor that corresponds to the prime $p$ . If $p\nmid q$ , then, using Theorem 1.5 and simple computations,

$$\begin{eqnarray}\displaystyle S_{p}=\mathop{\sum }_{k\geqslant 0}\biggl(\frac{1}{p^{k}}-\frac{1}{p^{k+1}}\biggr)M_{p}(F,\overline{F},p^{k})=\biggl|\biggl(1-\frac{1}{p}\biggr)\mathop{\sum }_{k\geqslant 0}\frac{F(p^{k})}{p^{k}}\biggr|^{2}. & & \displaystyle \nonumber\end{eqnarray}$$

If $p^{l}\Vert q$ , then again using Theorem 1.5 we get

$$\begin{eqnarray}\displaystyle S_{p}=\mathop{\sum }_{k\geqslant 0}\biggl(\frac{1}{p^{k}}-\frac{1}{p^{k+1}}\biggr)M_{p^{l}}(f,\overline{f},p^{k})=\frac{1}{p^{l-1}}\biggl(1-\frac{1}{p}\biggr)^{2}. & & \displaystyle \nonumber\end{eqnarray}$$

Since $c=0$ , one of the Euler factors has to be $0$ . The only possibility then is $S_{2}=0$ and $2\nmid q$ and $F(2^{k})=-1$ for all $k\geqslant 1$ . This completes the proof.◻

Proof of the Erdős–Coons–Tao conjecture

We now move on to the proof of Theorem 1.6. It turns out that periodic multiplicative functions with zero mean have the following equivalent characterization that we will use throughout the proof.

Proposition 4.4. Suppose that $f$ is multiplicative with each $|f(n)|\leqslant 1$ for all $n\in \mathbb{N}$ . Then there exists an integer $m$ such that $f(n+m)=f(n)$ for all $n\in \mathbb{N}$ and $\sum _{n=1}^{m}f(n)=0$ if and only if $f(2^{k})=-1$ for all $k\geqslant 1$ and there exists an integer $M$ such that if the prime power $p^{k}\geqslant M$ , then $f(p^{k})=f(p^{k-1})$ .

Proof. Suppose that $f(n+m)=f(n)$ for all $n\geqslant 1$ and $\sum _{n=1}^{m}f(n)=0$ . From periodicity, we have $f(km)=f(m)$ for all $k\geqslant 1$ and so, if $p^{a}\Vert m$ , then $f(p^{b})=f(p^{a})$ for all $b\geqslant a$ . In particular, if $p$ does not divide $m$ , then $f(p^{b})=1$ . Hence,

$$\begin{eqnarray}\mathop{\sum }_{n=1}^{m}f(n)=\mathop{\sum }_{d|m}f(d)\unicode[STIX]{x1D719}\biggl(\frac{m}{d}\biggr)=\mathop{\prod }_{p^{a}\Vert m}\biggl(p^{a}\biggl(1-\frac{1}{p}\biggr)\biggl(\mathop{\sum }_{1\leqslant k\leqslant a-1}\frac{f(p^{k})}{p^{k}}\biggr)+f(p^{a})\biggr).\end{eqnarray}$$

Consequently, some factor has to be $0$ . The only possibility is then $p=2$ and $f(2^{k})=-1$ for all $k\geqslant 1$ . The other direction immediately follows from the Chinese remainder theorem.◻

Our starting point is the following result.

Theorem 4.5 (Tao, 2015).

If, for a multiplicative $f:\mathbb{N}\rightarrow \{-1,1\}$ ,

$$\begin{eqnarray}\limsup _{x\rightarrow \infty }\biggl|\mathop{\sum }_{n\leqslant x}f(n)\biggr|<\infty ,\end{eqnarray}$$

then $f(2^{j})=-1$ for all $j\geqslant 1$ and

$$\begin{eqnarray}\mathop{\sum }_{p}\frac{1-f(p)}{p}<\infty .\end{eqnarray}$$

In what follows we restrict ourselves to the multiplicative functions $f:\mathbb{N}\rightarrow \{-1,1\}$ such that $\mathbb{D}(1,f,\infty )<\infty$ , $f=1\ast g$ and $f(2^{j})=-1$ for all $j\geqslant 1$ . For such such functions, we are going to drop the subscript and set

(16) $$\begin{eqnarray}G_{0}(a)=G(a):=\mathop{\prod }_{p^{k}||a}\biggl(|g(p^{k})|^{2}+2\mathop{\sum }_{i\geqslant k+1}\frac{g(p^{k})g(p^{i})}{p^{i-k}}\biggr).\end{eqnarray}$$

Here, we allow $k=0$ if $p\nmid a$ . The following lemma summarizes properties of $G(a)$ that we will use throughout the proof.

Lemma 4.6. Let $G(a)$ be as above. Then:

1. (i) $G(4a)=0$ , $a\in \mathbb{N}$ ;

2. (ii) $G(2a)=-4G(a)$ for odd $a$ ;

3. (iii) $\sum _{a\geqslant 1}(G(a)/a^{2})=0$ ;

4. (iv) if $f(3)=1$ , then $G(a)\leqslant 0$ for all odd $a$ ;

5. (v) $\sum _{a\geqslant 1}(G(a)/a)=1$ .

Proof. Note that $g(2)=-2$ and $g(2^{i})=f(2^{i})-f(2^{i-1})=0$ for $i\geqslant 2$ . Thus, $G(4a)=0$ and $G(2a)=-4G(a)$ for odd $a$ . The third part immediately follows from

$$\begin{eqnarray}\displaystyle \mathop{\sum }_{a\geqslant 1}\frac{G(a)}{a^{2}}=\mathop{\sum }_{a\geqslant 1,\,a\,\text{odd}}\frac{G(a)}{a^{2}}+\mathop{\sum }_{a\geqslant 1,\,a\,\text{odd}}\frac{G(2a)}{(2a)^{2}}=0. & & \displaystyle \nonumber\end{eqnarray}$$

To prove (iv), fix $p$ and suppose that $p^{k}\Vert a$ . We note that for $k=0$ , the Euler factor

$$\begin{eqnarray}E_{p}(a)=1+2\mathop{\sum }_{i\geqslant 1}\frac{g(p^{i})}{p^{i}}\geqslant 1-\frac{4}{p-1}\geqslant 0\end{eqnarray}$$

for $p\geqslant 5$ . Note that $E_{2}(a)=1-2=-1$ . If $3^{0}\Vert a$ , then $g(3)=f(3)-1=0$ and $E_{3}(a)\geqslant 1-\frac{4}{9}\cdot \frac{3}{2}=\frac{1}{3}>0$ . Suppose that $p^{k}\Vert a$ and $k\geqslant 1$ . Then

$$\begin{eqnarray}E_{p}(a)=|g(p^{k})|^{2}+2\mathop{\sum }_{i\geqslant k+1}\frac{g(p^{k})g(p^{i})}{p^{i-k}}\geqslant 4-\frac{8}{p-1}\geqslant 0\end{eqnarray}$$

for $p\geqslant 3$ . Hence, the only negative Euler factor is $E_{2}$ and (iv) follows. To prove (v), we take $m=0$ in Corollary 3.3 to arrive at

$$\begin{eqnarray}\lim _{x\rightarrow \infty }\frac{1}{x}\mathop{\sum }_{n\leqslant x}f(n)\overline{f(n+0)}=1=\mathop{\sum }_{a|0}\frac{G(a)}{a}=\mathop{\sum }_{a\geqslant 1}\frac{G(a)}{a}.\square\end{eqnarray}$$

Lemma 4.7. Suppose that $G(a)\neq 0$ . Then

$$\begin{eqnarray}|G(a)|\gg ({\textstyle \frac{5}{4}})^{\unicode[STIX]{x1D714}(a)-1}\cdot {\textstyle \frac{2}{5}}\cdot |G(1)|.\end{eqnarray}$$

Proof. Recall that

$$\begin{eqnarray}\displaystyle & \displaystyle G(a)=\mathop{\prod }_{p^{k}||a}\biggl(|g(p^{k})|^{2}+2\mathop{\sum }_{i\geqslant k+1}\frac{g(p^{k})g(p^{i})}{p^{i-k}}\biggr). & \displaystyle \nonumber\end{eqnarray}$$

Note that $g(p^{k})g(p^{k+1})\leqslant 0$ and so if $p^{k}\Vert a$ and $k\geqslant 1$ we have

$$\begin{eqnarray}E_{p}(a)=|g(p^{k})|^{2}+2\mathop{\sum }_{i\geqslant k+1}\frac{g(p^{k})g(p^{i})}{p^{i-k}}\geqslant 4-\frac{8}{p}\cdot \frac{1}{1-1/p^{2}}=4-\frac{8p}{p^{2}-1}.\end{eqnarray}$$

For $p=3$ , the last bound reduces to $E_{3}(a)\geqslant 1$ and for $p\geqslant 5$ we clearly have $E_{p}(a)\geqslant 2$ . For $k=0$ , we have

$$\begin{eqnarray}E_{p}(1)=1+2\mathop{\sum }_{i\geqslant 1}\frac{g(p^{i})}{p^{i}}\leqslant 1+\frac{4}{p}\cdot \frac{1}{1-1/p^{2}}=1+\frac{4p}{p^{2}-1}.\end{eqnarray}$$

Consequently, for $k\geqslant 1$ and $p>3$ ,

$$\begin{eqnarray}E_{p}(p^{k})\geqslant {\textstyle \frac{5}{4}}E_{p}(1).\end{eqnarray}$$

Taking into account $p=3$ , we conclude that

$$\begin{eqnarray}|G(a)|=\biggl|\mathop{\prod }_{p^{k}||a,~k\geqslant 1}\biggl(|g(p^{k})|^{2}+2\mathop{\sum }_{i\geqslant k+1}\frac{g(p^{k})g(p^{i})}{p^{i-k}}\biggr)\biggr|\geqslant \biggl(\frac{5}{4}\biggr)^{\unicode[STIX]{x1D714}(a)-1}\cdot \frac{2}{5}\cdot |G(1)|.\square\end{eqnarray}$$

In fact, it is easy to check that $G(1)\neq 0$ and thus the last lemma provides a non-trivial lower bound for $G(a)$ . In the next lemma we compute the second moment of the partial sums over the interval of fixed length.

Lemma 4.8. Let $H\in \mathbb{N}$ . Then

$$\begin{eqnarray}\frac{1}{x}\mathop{\sum }_{n\leqslant x}\biggl(\mathop{\sum }_{k=n+1}^{n+H+1}f(k)\biggr)^{2}=-2\mathop{\sum }_{a\geqslant 1,a\,\text{odd}}G(a)\biggl\|\frac{H}{2a}\biggr\|+o_{x\rightarrow \infty }(1).\end{eqnarray}$$

Proof. Note that

$$\begin{eqnarray}\displaystyle \frac{1}{x}\mathop{\sum }_{n\leqslant x}\biggl(\mathop{\sum }_{k=n+1}^{n+H+1}f(k)\biggr)^{2} & = & \displaystyle \frac{1}{x}\biggl[\mathop{\sum }_{h=0,~n\leqslant x}Hf(n)f(n+h)+2\mathop{\sum }_{1\leqslant h\leqslant H}(H-h)\mathop{\sum }_{n\leqslant x}f(n)f(n+h)\biggr]+o(1)\nonumber\\ \displaystyle & = & \displaystyle \mathop{\sum }_{a\geqslant 1}\frac{G(a)}{a}\biggl(H+2\mathop{\sum }_{\substack{ 1\leqslant h\leqslant H, \\ a|h}}(H-h)\biggr)+o_{x\rightarrow \infty }(1).\nonumber\end{eqnarray}$$

To compute the corresponding coefficient, we write $H=ra+s$ , $0\leqslant s<a$ , to arrive at

$$\begin{eqnarray}\displaystyle ra+s+2\mathop{\sum }_{1\leqslant m\leqslant r}(ra+s-ma) & = & \displaystyle ra+s+ar(r-1)+2rs\nonumber\\ \displaystyle & = & \displaystyle \frac{(ra+s)^{2}}{a}+a\biggl(\frac{s}{a}-\biggl(\frac{s}{a}\biggr)^{2}\biggr).\nonumber\end{eqnarray}$$

Inserting this into our formula and using (iii), (i) and (ii) from Lemma 4.6, we get

$$\begin{eqnarray}\displaystyle & & \displaystyle \displaystyle H^{2}\mathop{\sum }_{a\geqslant 1}\frac{G(a)}{a^{2}}+\mathop{\sum }_{a\geqslant 1}G(a)\biggl(\biggl\{\frac{H}{a}\biggr\}-\biggl\{\frac{H}{a}\biggr\}^{2}\biggr)\nonumber\\ \displaystyle & & \displaystyle \displaystyle \quad =\mathop{\sum }_{a\geqslant 1}G(a)\biggl(\biggl\{\frac{H}{a}\biggr\}-\biggl\{\frac{H}{a}\biggr\}^{2}\biggr)\nonumber\\ \displaystyle & & \displaystyle \displaystyle \quad =\mathop{\sum }_{a\geqslant 1,a\,\text{odd}}G(a)\biggl[\biggl(\biggl\{\frac{H}{a}\biggr\}-\biggl\{\frac{H}{a}\biggr\}^{2}\biggr)-4\biggl(\biggl\{\frac{H}{2a}\biggr\}-\biggl\{\frac{H}{2a}\biggr\}^{2}\biggr)\biggr]\nonumber\\ \displaystyle & & \displaystyle \displaystyle \quad =-2\mathop{\sum }_{a\geqslant 1,a\,\text{odd}}G(a)\biggl\|\frac{H}{2a}\biggr\|,\nonumber\end{eqnarray}$$

since

$$\begin{eqnarray}\biggl(\biggl\{\frac{H}{a}\biggr\}-\biggl\{\frac{H}{a}\biggr\}^{2}\biggr)-4\biggl(\biggl\{\frac{H}{2a}\biggr\}-\biggl\{\frac{H}{2a}\biggr\}^{2}\biggr)=-2\biggl\|\frac{H}{2a}\biggr\|,\end{eqnarray}$$

where $\Vert x\Vert$ denotes the distance from $x$ to the nearest integer.◻

We are now ready to prove Theorem 1.6.

Theorem 1.6 . Let $f:\mathbb{N}\rightarrow \{-1,1\}$ be a multiplicative function. Then

$$\begin{eqnarray}\displaystyle \limsup _{x\rightarrow \infty }\biggl|\mathop{\sum }_{n\leqslant x}f(n)\biggr|<\infty & & \displaystyle \nonumber\end{eqnarray}$$

if and only if there exists an integer $m\geqslant 1$ such that $f(n+m)=f(n)$ for all $n\geqslant 1$ and $\sum _{n=1}^{m}f(n)=0$ .

Proof. If $f$ satisfies $\sum _{i=1}^{m}f(i)=0$ and $f(n)=f(n+m)$ for some $m\geqslant 1$ , then, for all $x\geqslant 1$ ,

$$\begin{eqnarray}\biggl|\mathop{\sum }_{n\leqslant x}f(n)\biggr|\leqslant m\end{eqnarray}$$

and the claim follows. In the other direction, we assume that $|\!\sum _{n\leqslant x}f(n)|=O_{x\rightarrow \infty }(1)$ . By Theorem 4.5, we must have $f(2^{i})=-1$ for all $i\geqslant 1$ and $\mathbb{D}(1,f,\infty )<\infty$ . By Lemma 4.8, we must have that for all $H\geqslant 1$ ,

$$\begin{eqnarray}\frac{1}{x}\mathop{\sum }_{n\leqslant x}\biggl(\mathop{\sum }_{k=n+1}^{n+H+1}f(k)\biggr)^{2}=-2\mathop{\sum }_{a\geqslant 1,a\,\text{odd}}G(a)\biggl\|\frac{H}{2a}\biggr\|+o_{x\rightarrow \infty }(1)=O_{x\rightarrow \infty }(1).\end{eqnarray}$$

Suppose that there is an infinite sequence of odd numbers $\{a_{n}\}_{n\geqslant 1}$ such that $g(a_{n})\neq 0$ . Observe that $|G(a_{n})|\gg 1$ . Choose $H=\text{lcm}[a_{1},\ldots ,a_{M}]$ . If $f(3)=1$ , then, by Lemma 4.6, part (iv), we have

$$\begin{eqnarray}-2\mathop{\sum }_{a\geqslant 1,a\,\text{odd}}G(a)\biggl\|\frac{H}{2a}\biggr\|\geqslant -2\mathop{\sum }_{1\leqslant n\leqslant M}G(a_{n})\biggl\|\frac{H}{2a_{n}}\biggr\|\gg M.\end{eqnarray}$$

This is clearly impossible if $M$ is sufficiently large.

Suppose that $f(3)=-1$ . Let

$$\begin{eqnarray}G^{\ast }(a)=\mathop{\prod }_{p^{k}\Vert a,~p>3}\biggl(|g(p^{k})|^{2}+2\mathop{\sum }_{i\geqslant k+1}\frac{g(p^{k})g(p^{i})}{p^{i-k}}\biggr)\end{eqnarray}$$

and

$$\begin{eqnarray}S(H)=-2\mathop{\sum }_{a\geqslant 1,~(a,6)=1}G^{\ast }(a)\biggl\|\frac{H}{2a}\biggr\|.\end{eqnarray}$$

Note that

(17) $$\begin{eqnarray}-2\mathop{\sum }_{a\geqslant 1,a\,\text{odd}}G(a)\biggl\|\frac{H}{2a}\biggr\|=\mathop{\sum }_{i\geqslant 0}E_{3}(3^{i})S\biggl(\frac{H}{3^{i}}\biggr)=O(1).\end{eqnarray}$$

If $E_{3}(1)\geqslant 0$ , then we proceed as in the previous case. If $E_{3}(1)<0$ , then $g(3)=f(3)-1=-2$ . Since $g(p^{k})g(p^{k+1})\leqslant 0$ for all $k\geqslant 0$ , we get

$$\begin{eqnarray}E_{3}(3)\geqslant 4-\frac{8}{9}\cdot \frac{1}{1-1/9}\geqslant 3\end{eqnarray}$$

and

$$\begin{eqnarray}0>E_{3}(1)=1+2\mathop{\sum }_{i\geqslant 1}\frac{g(3^{i})}{3^{i}}\geqslant 1-\frac{4}{3}\cdot \frac{1}{1-1/9}=-\frac{1}{2}.\end{eqnarray}$$

Since $E_{3}(3^{k})\geqslant 0$ for all $k\geqslant 1$ , applying the triangle inequality in (17) yields

(18) $$\begin{eqnarray}S(H)\geqslant \frac{E_{3}(3)S(H/3)}{-E_{3}(1)}+O(1)\geqslant 6S\biggl(\frac{H}{3}\biggr)-M.\end{eqnarray}$$

If there is an infinite sequence $\{b_{n}\}_{n\geqslant 1}$ such that $g(b_{n})\neq 0$ and $(b_{n},6)=1$ , then we select $H_{0}$ as before such that $S(H_{0})\geqslant M$ and $S(3H_{0})\geqslant M$ . Then (18) yields $S(3H_{0})\geqslant 5S(H_{0})$ . By induction, one easily gets that for all $n\geqslant 1$ ,

$$\begin{eqnarray}S(3^{n}H_{0})\geqslant 5^{n}S(H_{0}).\end{eqnarray}$$

This implies that, for the sequence $H_{n}=3^{n}H_{0}$ , we have $S(H_{n})\gg H_{n}^{1+c}$ . On the other hand,

$$\begin{eqnarray}\mathop{\sum }_{a\geqslant H,~(a,6)=1}\frac{G^{\ast }(a)}{a}=o_{H\rightarrow \infty }(1)\end{eqnarray}$$

and so

$$\begin{eqnarray}\displaystyle S(H) & = & \displaystyle -2\mathop{\sum }_{a\geqslant 1,\,(a,6)=1}G^{\ast }(a)\biggl\|\frac{H}{2a}\biggr\|\ll \mathop{\sum }_{a\leqslant H,\,(a,6)=1}G^{\ast }(a)+H\mathop{\sum }_{a\geqslant H,\,(a,6)=1}\frac{G^{\ast }(a)}{a}\nonumber\\ \displaystyle & \ll & \displaystyle \sqrt{H}\mathop{\sum }_{a\leqslant \sqrt{H},\,(a,6)=1}\frac{G^{\ast }(a)}{a}+H\mathop{\sum }_{\sqrt{H}\leqslant a\leqslant H,\,(a,6)=1}\frac{G^{\ast }(a)}{a}+o(H)\nonumber\end{eqnarray}$$

and so $S(H)=o(H)$ .

To finish the proof, we are left to handle the case $g(3^{k})\neq 0$ for infinitely many $k\geqslant 1$ and there exists finitely many $b_{1},b_{2},\ldots ,b_{m}$ $(b_{i},6)=1$ , $i\geqslant 1$ and $g(b_{i})\neq 0$ . In this case we have

$$\begin{eqnarray}S(H)\leqslant \mathop{\sum }_{i=1}^{m}G^{\ast }(b_{i}):=M.\end{eqnarray}$$

Choose $H_{0}=\text{lcm}[b_{1},\ldots ,b_{m}]$ and observe that $S(3^{k}H_{0})\geqslant M/2$ for $k=1,\ldots ,K$ . Then

$$\begin{eqnarray}\displaystyle -\!2\mathop{\sum }_{a\geqslant 1,a\,\text{odd}}G(a)\biggl\|\frac{3^{K}H_{0}}{2a}\biggr\| & = & \displaystyle \mathop{\sum }_{i\geqslant 0}E_{3}(3^{i})S\biggl(\frac{3^{K}H_{0}}{3^{i}}\biggr)\nonumber\\ \displaystyle & {\geqslant} & \displaystyle \mathop{\sum }_{1\leqslant i\leqslant K}E_{3}(3^{i})S\biggl(\frac{3^{K}H_{0}}{3^{i}}\biggr)-E_{3}(1)S(H_{0})\nonumber\\ \displaystyle & {\geqslant} & \displaystyle \frac{M}{2}\mathop{\sum }_{1\leqslant i\leqslant K}E_{3}(3^{k})-M.\nonumber\end{eqnarray}$$

The last sum is bounded if $E_{3}(3^{k})=0$ for all $k\geqslant K_{0}$ . Consequently, $f(3^{k})=f(3^{k+1})$ for $k\geqslant K_{0}$ and the result follows.◻

5 Applications to the conjecture of Kátai

Let $f:\mathbb{N}\rightarrow \mathbb{C}$ be a multiplicative function and $\triangle f(n)=f(n+1)-f(n)$ . In this section, we focus on proving the following result.

Theorem 1.8 . If $f:\mathbb{N}\rightarrow \mathbb{C}$ is a multiplicative function and

(19) $$\begin{eqnarray}\lim _{x\rightarrow \infty }\frac{1}{x}\mathop{\sum }_{n\leqslant x}|\triangle f(n)|=0,\end{eqnarray}$$

then either

$$\begin{eqnarray}\lim _{x\rightarrow \infty }\frac{1}{x}\mathop{\sum }_{n\leqslant x}|f(n)|=0\end{eqnarray}$$

or $f(n)=n^{s}$ for some $\operatorname{Re}(s)<1$ .

In [Kát00], Kátai, building on the ideas of Mauclaire and Murata [MM80], showed that in order to prove Theorem 1.8, it is enough to consider a multiplicative $f$ with $|f(n)|=1$ for all $n\geqslant 1$ . Observe that, if we denote

$$\begin{eqnarray}S(x)=\frac{1}{x}\mathop{\sum }_{n\leqslant x}|\triangle (n)|,\end{eqnarray}$$

then (19) implies that

$$\begin{eqnarray}\mathop{\sum }_{n\leqslant x}\frac{|\triangle f(n)|^{2}}{n}\leqslant \mathop{\sum }_{n\leqslant x}\frac{2|\triangle f(n)|}{n}\ll \int _{1}^{x}\frac{S(t)}{t^{2}}\,dt=o(\log x).\end{eqnarray}$$

We begin by proving the following lemma.

Lemma 5.1. Suppose that $f:\mathbb{N}\rightarrow \mathbb{T}$ is multiplicative and

$$\begin{eqnarray}\mathop{\sum }_{n\leqslant x}\frac{|\triangle f(n)|^{2}}{n}\leqslant 2(1-\unicode[STIX]{x1D700})\log x\end{eqnarray}$$

for $x$ sufficiently large and some $0<\unicode[STIX]{x1D700}<1$ . Then there exist a primitive character $\unicode[STIX]{x1D712}_{1}(n)$ and $t_{\unicode[STIX]{x1D712}_{1}}\in \mathbb{R}$ such that $\mathbb{D}(f(n),\unicode[STIX]{x1D712}_{1}(n)n^{it_{\unicode[STIX]{x1D712}_{1}}};\infty )<\infty$ .

Proof. We note that

$$\begin{eqnarray}\operatorname{Re}f(n)\overline{f(n+1)}=1-\frac{|\triangle f(n)|^{2}}{2}\end{eqnarray}$$

and therefore

$$\begin{eqnarray}\mathop{\sum }_{n\leqslant x}\frac{\operatorname{Re}f(n)\overline{f(n+1)}}{n}\geqslant \unicode[STIX]{x1D700}\log x+O(1).\end{eqnarray}$$

We can now apply Lemma 4.3, since the only fact that was used in the proof is that the logarithmic correlation is large, to conclude the result. ◻

Remark 5.2. The conclusion of the lemma also holds if $f:\mathbb{N}\rightarrow \mathbb{T}$ satisfies

$$\begin{eqnarray}\mathop{\sum }_{n\leqslant x}\frac{|\triangle f(n)|^{2}}{n}\geqslant 2(1+\unicode[STIX]{x1D700})\log x\end{eqnarray}$$

for some $\unicode[STIX]{x1D700}>0$ . In other words, if $\sum _{n\leqslant x}(|\triangle f(n)|^{2}/n)$ is bounded away from $2\log x$ , then

$$\begin{eqnarray}\mathbb{D}(f(n),\unicode[STIX]{x1D712}_{1}(n)n^{it_{\unicode[STIX]{x1D712}_{1}}};\infty )<\infty .\end{eqnarray}$$

Proposition 5.3. Let $f:\mathbb{N}\rightarrow \mathbb{T}$ be a multiplicative function and $\mathbb{D}(f,n^{it}\unicode[STIX]{x1D712}(n);\infty )<\infty$ for some $t\in \mathbb{R}$ and a primitive character $\unicode[STIX]{x1D712}$ of conductor $q$ . Then

$$\begin{eqnarray}\mathop{\sum }_{n\leqslant x}\frac{|\triangle f(n)|^{2}}{n}=2(1-E(f)+o(1))\log x,\end{eqnarray}$$

where

$$\begin{eqnarray}E(f)=\frac{\unicode[STIX]{x1D707}(q)}{q}\mathop{\prod }_{\substack{ p\geqslant 1 \\ p\nmid q}}\biggl(2\operatorname{Re}\biggl(1-\frac{1}{p}\biggr)\biggl(\mathop{\sum }_{k\geqslant 0}\frac{f(p^{k})\overline{\unicode[STIX]{x1D712}(p^{k})}p^{-ikt}}{p^{k}}\biggr)-1\biggr).\end{eqnarray}$$

Proof. Applying Corollary 3.4, we have that

$$\begin{eqnarray}M(y)=\mathop{\sum }_{n\leqslant y}f(n)\overline{f(n+1)}=y\frac{\unicode[STIX]{x1D707}(q)}{q}\mathop{\prod }_{\substack{ p\geqslant 1 \\ p\nmid q}}\biggl(2\operatorname{Re}\biggl(1-\frac{1}{p}\biggr)\biggl(\mathop{\sum }_{k\geqslant 0}\frac{f(p^{k})\overline{\unicode[STIX]{x1D712}(p^{k})}p^{-ikt}}{p^{k}}\biggr)-1\biggr)+o(y).\end{eqnarray}$$

Consequently,

$$\begin{eqnarray}\mathop{\sum }_{n\leqslant x}\frac{\operatorname{Re}f(n)\overline{f(n+1)}}{n}=\frac{M(x)}{x}+\int _{1}^{x}\frac{M(y)}{y^{2}}\,dy=\log x\cdot E(f)+o(\log x)\end{eqnarray}$$

and

$$\begin{eqnarray}\mathop{\sum }_{n\leqslant x}\frac{|\triangle f(n)|^{2}}{n}=2\log x-2\mathop{\sum }_{n\leqslant x}\frac{\operatorname{Re}f(n)\overline{f(n+1)}}{n}+O(1)=2(1-E(f)+o(1))\log x.\square\end{eqnarray}$$

Corollary 5.4. Let $f:\mathbb{N}\rightarrow \mathbb{T}$ be a multiplicative function such that $\mathbb{D}(f,n^{it}\unicode[STIX]{x1D712}(n);\infty )<\infty$ for some $t\in \mathbb{R}$ and a primitive character $\unicode[STIX]{x1D712}$ of conductor $q$ . Suppose that

$$\begin{eqnarray}\mathop{\sum }_{n\leqslant x}\frac{|\triangle f(n)|^{2}}{n}=o(\log x).\end{eqnarray}$$

Then $f(n)=n^{it}$ .

Proof. Proposition 5.3 implies that $E(f)=1$ . We have that for all $p\geqslant 2$ , $p\nmid q$ , each Euler factor

$$\begin{eqnarray}E_{p}(f)=2\biggl(1-\frac{1}{p}\biggr)\mathop{\sum }_{k\geqslant 0}\frac{\operatorname{Re}f(p^{k})\overline{\unicode[STIX]{x1D712}(p^{k})}p^{-ikt}}{p^{k}}-1\geqslant 2\biggl(1-\frac{1}{p}\biggr)\biggl(1-\mathop{\sum }_{k\geqslant 1}\frac{1}{p^{k}}\biggr)-1=\frac{p-4}{p}\geqslant -1\end{eqnarray}$$

with the possible equality only at $p=2$ . On the other hand,

$$\begin{eqnarray}E_{p}(f)\leqslant 2\biggl(1-\frac{1}{p}\biggr)\biggl(\mathop{\sum }_{k\geqslant 0}\frac{1}{p^{k}}\biggr)-1=1.\end{eqnarray}$$

Consequently, we must have $q=1$ and $|E_{p}(f)|=1$ for all $p\geqslant 2$ . Since $E(f)=1>0$ , we have $E_{2}(f)\neq -1$ and

$$\begin{eqnarray}2\biggl(1-\frac{1}{p}\biggr)\mathop{\sum }_{k\geqslant 0}\frac{\operatorname{Re}f(p^{k})p^{-ikt}}{p^{k}}-1=1.\end{eqnarray}$$

This is possible if and only if $f(p^{k})=p^{kit}$ for all $p\geqslant 2$ and $k\geqslant 1$ . The result follows.◻

Theorem 1.8 now follows from the following result.

Proposition 5.5. Let $f:\mathbb{N}\rightarrow \mathbb{T}$ be a multiplicative function such that

$$\begin{eqnarray}\mathop{\sum }_{n\leqslant x}\frac{|\triangle f(n)|^{2}}{n}=o(\log x).\end{eqnarray}$$

Then $f(n)=n^{it}$ for some $t\in \mathbb{R}$ .

Proof. Applying Lemma 5.1, we can find a primitive character $\unicode[STIX]{x1D712}$ and $t\in \mathbb{R}$ such that

$$\begin{eqnarray}\mathbb{D}(f(n),\unicode[STIX]{x1D712}(n)n^{it};\infty )<\infty .\end{eqnarray}$$

We now apply Corollary 5.4 to conclude that $f(n)=n^{it}$ .◻

6 Applications to the binary additive problems

As was mentioned in the introduction, Brüdern established the following result.

Theorem 1.9  (Brüdern, 2008). Suppose that $A$ and $B$ are multiplicative sequences of positive densities $\unicode[STIX]{x1D70C}_{A}$ and $\unicode[STIX]{x1D70C}_{B}$ , respectively. For $k\geqslant 1$ , let

$$\begin{eqnarray}a(p^{k})=\unicode[STIX]{x1D70C}_{A}(p^{k})/p^{k}-\unicode[STIX]{x1D70C}_{A}(p^{k-1})/p^{k-1}.\end{eqnarray}$$

Define $b(h)$ in the same fashion. Then $r(n)=\unicode[STIX]{x1D70C}_{A}\unicode[STIX]{x1D70C}_{B}\unicode[STIX]{x1D70E}(n)n+o(n)$ when $n\rightarrow \infty$ , where

$$\begin{eqnarray}\unicode[STIX]{x1D70E}(n)=\mathop{\prod }_{p^{m}\Vert n}\biggl(1+\mathop{\sum }_{k=1}^{m}\frac{p^{k-1}a(p^{k})b(p^{k})}{p-1}-\frac{p^{m}a(p^{m+1})b(p^{m+1})}{(p-1)^{2}}\biggr).\end{eqnarray}$$

We now sketch how one can derive this from our main result.

Proof. Let $f(n)=\text{I}_{A}(n)$ and $g(n)=\text{I}_{B}(n)$ . Clearly, both $f$ and $g$ are multiplicative, taking values $\{0,1\}$ . Since $\unicode[STIX]{x1D70C}_{A}>0$ , we have

$$\begin{eqnarray}\limsup _{x}\frac{1}{x}\mathop{\sum }_{n\leqslant x}f(n)>0.\end{eqnarray}$$

The theorem of Delange readily implies that $\mathbb{D}(1,f;\infty )<\infty$ . By analogy, $\mathbb{D}(1,g;\infty )<\infty$ . Furthermore,

$$\begin{eqnarray}\unicode[STIX]{x1D70C}_{A}=\lim _{x\rightarrow \infty }\frac{1}{x}\mathop{\sum }_{n\leqslant x}f(n)=\mathfrak{P}(f,1,\infty )\end{eqnarray}$$

and

$$\begin{eqnarray}\unicode[STIX]{x1D70C}_{B}=\lim _{x\rightarrow \infty }\frac{1}{x}\mathop{\sum }_{n\leqslant x}g(n)=\mathfrak{P}(g,1,\infty ).\end{eqnarray}$$

Notice that

$$\begin{eqnarray}r(m)=\mathop{\sum }_{n\leqslant m}f(n)g(m-n).\end{eqnarray}$$

We note that following the proof of Corollary 1.4, we may let $a=1$ , $c=0$ , $b=-1$ and $d=m$ . Despite the fact that $d=m\rightarrow \infty$ , the error term is still bounded by (8). Corollary 1.4 gives

$$\begin{eqnarray}r(m)=\mathop{\sum }_{l|m}\frac{G(f;g;l;\infty )}{l}m+o(m).\end{eqnarray}$$

A straightforward manipulation with the Euler factors shows that the latter has the Euler product described above. ◻

Remark 6.1. In case one of the sets $A,B$ has density zero, say $\unicode[STIX]{x1D70C}_{A}=0$ , we can apply Delange’s theorem to conclude that

$$\begin{eqnarray}r(m)=\mathop{\sum }_{n\leqslant m}f(n)g(m-n)\leqslant \mathop{\sum }_{n\leqslant m}f(n)=o(m).\end{eqnarray}$$