Hostname: page-component-cd9895bd7-dzt6s Total loading time: 0 Render date: 2024-12-26T08:08:22.618Z Has data issue: false hasContentIssue false
  • English
  • Français

Cycles in the de Rham cohomology of abelian varieties over number fields

Part of: Arithmetic algebraic geometry (Co)homology theory Abelian varieties and schemes Arithmetic problems. Diophantine geometry

Published online by Cambridge University Press:  08 March 2018

Yunqing Tang
Yunqing Tang*
Affiliation:
Department of Mathematics, Princeton University, Fine Hall, Washington Road, Princeton, NJ 08540, USA email yunqingt@math.princeton.edu
Get access Rights & Permissions [Opens in a new window]

Abstract

In his 1982 paper, Ogus defined a class of cycles in the de Rham cohomology of smooth proper varieties over number fields. This notion is a crystalline analogue of 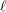$\ell$-adic Tate cycles. In the case of abelian varieties, this class includes all the Hodge cycles by the work of Deligne, Ogus, and Blasius. Ogus predicted that such cycles coincide with Hodge cycles for abelian varieties. In this paper, we confirm Ogus’ prediction for some families of abelian varieties. These families include geometrically simple abelian varieties of prime dimension that have non-trivial endomorphism ring. The proof uses a crystalline analogue of Faltings’ isogeny theorem due to Bost and the known cases of the Mumford–Tate conjecture.

Keywords

absolute Tate cyclesHodge cyclesMumford–Tate conjecturealgebraization theorems

MSC classification

Primary: 11G10: Abelian varieties of dimension $> 1$ 14F40: de Rham cohomology 14G40: Arithmetic varieties and schemes; Arakelov theory; heights 14K15: Arithmetic ground fields
Type
Research Article
Information
Compositio Mathematica , Volume 154 , Issue 4 , April 2018 , pp. 850 - 882
Copyright
© The Author 2018 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

André, Y., Une introduction aux motifs (motifs purs, motifs mixtes, périodes), Panoramas et Synthèses [Panoramas and Syntheses], vol. 17 (Société Mathématique de France, Paris, 2004); (French, with English and French summaries).Google Scholar
Baker, A. and Wüstholz, G., Logarithmic forms and diophantine geometry, New Mathematical Monographs, vol. 9 (Cambridge University Press, Cambridge, 2007).Google Scholar
Banaszak, G., Gajda, W. and Krasoń, P., On the image of l-adic Galois representations for abelian varieties of type I and II , Doc. Math. Extra Vol. (2006), 35–75; (electronic).Google Scholar
Banaszak, G., Gajda, W. and Krasoń, P., On the image of Galois l-adic representations for abelian varieties of type III , Tohoku Math. J. (2) 62 (2010), 163–189.CrossRefGoogle Scholar
Blasius, D., A p-adic property of Hodge classes on abelian varieties, Motives (Seattle, WA, 1991), Proceedings of Symposia in Pure Mathematics, vol. 55 (American Mathematical Society, Providence, RI, 1994), 293–308.Google Scholar
Bost, J.-B., Algebraic leaves of algebraic foliations over number fields , Publ. Math. Inst. Hautes Études Sci. 93 (2001), 161–221; English, with English and French summaries.CrossRefGoogle Scholar
Bost, J.-B., Evaluation maps, slopes, and algebraicity criteria, International Congress of Mathematicians, vol. II (European Mathematical Society, Zürich, 2006), 537–562.Google Scholar
Cantoral-Farfán, V., Points de torsion pour les variétés abéliennes de type III, PhD thesis, (2017).Google Scholar
Chambert-Loir, Antoine, Théorèmes d’algébricité en géométrie diophantienne (d’après J.-B. Bost, Y. André, D. & G. Chudnovsky) , Astérisque 282 (2002), Exp. No. 886, viii, 175–209 (French, with French summary). Séminaire Bourbaki, Vol. 2000/2001.Google Scholar
Chavdarov, N., The generic irreducibility of the numerator of the zeta function in a family of curves with large monodromy , Duke Math. J. 87 (1997), 151–180.CrossRefGoogle Scholar
Chi, W. C., On the l-adic representation attached to simple abelian varieties of type IV , Bull. Austral. Math. Soc. 44 (1991), 71–78.CrossRefGoogle Scholar
Chi, W. C., l-adic and 𝜆-adic representations associated to abelian varieties defined over number fields , Amer. J. Math. 114 (1992), 315–353.Google Scholar
de Jong, R., Gauss map on the theta divisor and Green’s functions , in Modular forms on Schiermonnikoog (Cambridge University Press, Cambridge, 2008), 67–78.Google Scholar
Deligne, P., Hodge cycles on abelian varieties , inHodge cycles, motives, and Shimura varieties, Lecture Notes in Mathematics, vol. 900 (Springer, Berlin–New York, 1982).CrossRefGoogle Scholar
Gasbarri, C., Analytic subvarieties with many rational points , Math. Ann. 346 (2010), 199–243.CrossRefGoogle Scholar
Griffiths, P. and King, J., Nevanlinna theory and holomorphic mappings between algebraic varieties , Acta Math. 130 (1973), 145–220.Google Scholar
Herblot, M., Algebraic points on meromorphic curves, Preprint (2012), arXiv:1204.6336.Google Scholar
Katz, N. M., Algebraic solutions of differential equations (p-curvature and the Hodge filtration) , Invent. Math. 18 (1972), 1–118.Google Scholar
Katz, N. M. and Messing, W., Some consequences of the Riemann hypothesis for varieties over finite fields , Invent. Math. 23 (1974), 73–77.Google Scholar
Kisin, M., Integral models for Shimura varieties of abelian type , J. Amer. Math. Soc. 23 (2010), 967–1012.Google Scholar
Kisin, M., mod p points on Shimura varieties of abelian type , J. Amer. Math. Soc. 30 (2017), 819–914.CrossRefGoogle Scholar
Lombardo, D., On the ℓ-adic Galois representations attached to non-simple abelian varieties , Ann. Inst. Fourier (Grenoble) 66 (2016), 1217–1245.Google Scholar
Mazur, B., Frobenius and the Hodge filtration (estimates) , Ann. of Math. (2) 98 (1973), 58–95.Google Scholar
Messing, W., The crystals associated to Barsotti-Tate groups: with applications to abelian schemes, Lecture Notes in Mathematics, vol. 264 (Springer, Berlin–New York, 1972).Google Scholar
Mumford, D., Abelian varieties, Tata Institute of Fundamental Research Studies in Mathematics, vol. 5 (Published for the Tata Institute of Fundamental Research, Bombay; by Hindustan Book Agency, New Delhi, 2008), With appendices by C. P. Ramanujam and Yuri Manin; Corrected reprint of the second (1974) edition.Google Scholar
Noot, R., Models of Shimura varieties in mixed characteristic , J. Algebraic Geom. 5 (1996), 187–207.Google Scholar
Noot, R., Classe de conjugaison du Frobenius d’une variété abélienne sur un corps de nombres , J. Lond. Math. Soc. (2) 79 (2009), 53–71; French, with English and French summaries.Google Scholar
Ogus, A., Hodge cycles and crystalline cohomology , in Hodge cycles, motives, and Shimura varieties, Lecture Notes in Mathematics, vol. 900 (Springer, Berlin–New York, 1982).Google Scholar
Pink, R., l-adic algebraic monodromy groups, cocharacters, and the Mumford-Tate conjecture , J. Reine Angew. Math. 495 (1998), 187–237.CrossRefGoogle Scholar
Serre, J.-P., A letter to Ken Ribet , inOeuvres/Collected papers. IV. 1985–1998, Springer Collected Works in Mathematics (Springer, Heidelberg, 2013), reprint of the 2000 edition.Google Scholar
Serre, J.-P., Lectures on N X (p), Chapman & Hall/CRC Research Notes in Mathematics, vol. 11 (CRC Press, Boca Raton, FL, 2012).Google Scholar
Vasiu, A., Some cases of the Mumford–Tate conjecture and Shimura varieties , Indiana Univ. Math. J. 57 (2008), 1–75.Google Scholar
Zarhin, Y. G., Abelian varieties having a reduction of K3 type , Duke Math. J. 65 (1992), 511–527.CrossRefGoogle Scholar