Hostname: page-component-78c5997874-xbtfd Total loading time: 0 Render date: 2024-11-10T11:29:28.011Z Has data issue: false hasContentIssue false
  • English
  • Français

Finiteness properties of cubulated groups

Part of: Special aspects of infinite or finite groups

Published online by Cambridge University Press:  10 March 2014

G. C. Hruska  and
Daniel T. Wise
G. C. Hruska
Affiliation:
Department of Mathematical Sciences, University of Wisconsin–Milwaukee, PO Box 413, Milwaukee, WI 53201, USA email chruska@uwm.edu
Daniel T. Wise
Affiliation:
Department of Mathematics & Statistics, McGill University, Montreal, QC, Canada H3A 0B9 email wise@math.mcgill.ca
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We give a generalized and self-contained account of Haglund–Paulin’s wallspaces and Sageev’s construction of the CAT(0) cube complex dual to a wallspace. We examine criteria on a wallspace leading to finiteness properties of its dual cube complex. Our discussion is aimed at readers wishing to apply these methods to produce actions of groups on cube complexes and understand their nature. We develop the wallspace ideas in a level of generality that facilitates their application. Our main result describes the structure of dual cube complexes arising from relatively hyperbolic groups. 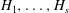Let $H_1,\ldots, H_s$ be relatively quasiconvex codimension-1 subgroups of a group 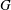$G$ that is hyperbolic relative to $P_1, \ldots, P_r$. We prove that 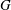$G$ acts relatively cocompactly on the associated dual CAT(0) cube complex $C$. This generalizes Sageev’s result that 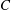$C$ is cocompact when 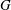$G$ is hyperbolic. When 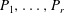$P_1,\ldots, P_r$ are abelian, we show that the dual CAT(0) cube complex $C$ has a 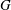$G$-cocompact CAT(0) truncation.

Keywords

cube complexwallspacerelatively hyperbolic group

MSC classification

Secondary: 20F65: Geometric group theory 20F67: Hyperbolic groups and nonpositively curved groups
Type
Research Article
Information
Compositio Mathematica , Volume 150 , Issue 3 , March 2014 , pp. 453 - 506
Copyright
© The Author(s) 2014 

References

Aitchison, I. R. and Rubinstein, J. H., An introduction to polyhedral metrics of nonpositive curvature on 3-manifolds, in Geometry of low-dimensional manifolds: 2. Symplectic manifolds and Jones–Witten theory Durham, 1989, London Mathematical Society Lecture Note Series, vol. 151, eds Donaldson, S. K. and Thomas, C. B., (Cambridge University Press, Cambridge, 1990), 127–161.Google Scholar
Alibegović, E. and Bestvina, M., Limit groups are CAT(0), J. Lond. Math. Soc. (2) 74 (2006), 259–272.Google Scholar
Barré, S. and Pichot, M., Removing chambers in Bruhat–Tits buildings, Preprint (2010),arXiv:1003.4614 [math.MG].Google Scholar
Bergeron, N., Haglund, F. and Wise, D. T., Hyperplane sections in arithmetic hyperbolic manifolds, J. Lond. Math. Soc. (2) 83 (2011), 431–448.Google Scholar
Bergeron, N. and Wise, D. T., A boundary criterion for cubulation, Amer. J. Math. 134 (2012), 843–859.Google Scholar
Bowditch, B. H., Relatively hyperbolic groups, Internat. J. Algebra Comput. 22 (2012), doi:10.1142/S0218196712500166.Google Scholar
Bridson, M. R. and Haefliger, A., Metric spaces of non-positive curvature (Springer, Berlin, 1999).Google Scholar
Brink, B. and Howlett, R. B., A finiteness property and an automatic structure for Coxeter groups, Math. Ann. 296 (1993), 179–190.Google Scholar
Caprace, P.-E. and Przytycki, P., Bipolar Coxeter groups, J. Algebra 338 (2011), 35–55.Google Scholar
Caprace, P.-E. and Sageev, M., Rank rigidity for CAT(0) cube complexes, Geom. Funct. Anal. 21 (2011), 851–891.Google Scholar
Chatterji, I. and Niblo, G., From wall spaces to CAT(0) cube complexes, Internat. J. Algebra Comput. 15 (2005), 875–885.Google Scholar
Cherix, P.-A., Cowling, M., Jolissaint, P., Julg, P. and Valette, A.,Groups with the Haagerup property: Gromov’s a-T-menability, Progress in Mathematics, vol. 197 (Birkhäuser, Basel, 2001).Google Scholar
Dahmani, F., Combination of convergence groups, Geom. Topol. 7 (2003), 933–963.Google Scholar
Druţu, C. and Sapir, M., Tree-graded spaces and asymptotic cones of groups, Topology 44 (2005), 959–1058; With an appendix by Denis Osin and Mark Sapir.Google Scholar
Farley, D. S., Finiteness and CAT(0) properties of diagram groups, Topology 42 (2003), 1065–1082.CrossRefGoogle Scholar
Gautero, F., A non-trivial example of a free-by-free group with the Haagerup property, Groups Geom. Dyn. 6 (2012), 677–699.Google Scholar
Geoghegan, R.,Topological methods in group theory, Graduate Texts in Mathematics, vol. 243 (Springer, New York, 2008).Google Scholar
Gerasimov, V. N., Semi-splittings of groups and actions on cubings, in Algebra, geometry, analysis and mathematical physics (Russian) Novosibirsk, 1996, Izdat. Ross. Akad. Nauk Sib. Otd. Inst. Mat., eds Reshetnyak, Yu. G., Bokut’, L. A., Vodop’yanov, S. K. and Taĭmanov, I. A., (1997), 91–109; Engl. transl. Fixed-point-free actions on cubings, Siberian Adv. Math. 8 (1998), 36–58.Google Scholar
Gitik, R., Mitra, M., Rips, E. and Sageev, M., Widths of subgroups, Trans. Amer. Math. Soc. 350 (1998), 321–329.Google Scholar
Gromov, M., Hyperbolic groups, in Essays in group theory, Mathematical Sciences Research Institute Publications, vol. 8, ed Gersten, S. M., (Springer, New York, 1987), 75–263.Google Scholar
Guba, V. S. and Sapir, M. V., Diagram groups and directed 2-complexes: homotopy and homology, J. Pure Appl. Algebra 205 (2006), 1–47.Google Scholar
Guralnik, D., Coarse decompositions of boundaries for CAT(0) groups, Preprint (2006),arXiv:math/0611006 [math.GR].Google Scholar
Guralnik, D., Local finiteness for cubulations of CAT(0) groups, Preprint (2006),arXiv:math/0610950 [math.GR].Google Scholar
Hagen, M. F., Weak hyperbolicity of cube complexes and quasi-arboreal groups, J. Topology, published online 23 August 2013, doi:10.1112/jtopol/jtt027, 34 pages.Google Scholar
Haglund, F. and Paulin, F., Simplicité de groupes d’automorphismes d’espaces à courbure négative, in The Epstein birthday schrift, Geometry & Topology Monographs, vol. 1, eds Rivin, I., Rourke, C. and Series, C., (Geometry & Topology Publications, Coventry, 1998), 181–248.Google Scholar
Haglund, F. and Wise, D. T., Special cube complexes, Geom. Funct. Anal. 17 (2008), 1551–1620.Google Scholar
Haglund, F. and Wise, D. T., Coxeter groups are virtually special, Adv. Math. 224 (2010), 1890–1903.Google Scholar
Hass, J. and Scott, P., Homotopy equivalence and homeomorphism of 3-manifolds, Topology 31 (1992), 493–517.Google Scholar
Hruska, G. C., Relative hyperbolicity and relative quasiconvexity for countable groups, Algebr. Geom. Topol. 10 (2010), 1807–1856.Google Scholar
Hruska, G. C. and Wise, D. T., Packing subgroups in relatively hyperbolic groups, Geom. Topol. 13 (2009), 1945–1988.CrossRefGoogle Scholar
Hsu, T. and Wise, D. T., Cubulating graphs of free groups with cyclic edge groups, Amer. J. Math. 132 (2010), 1153–1188.Google Scholar
Janzen, D. and Wise, D. T., Cubulating rhombus groups, Groups Geom. Dyn. 7 (2013), 419–442.CrossRefGoogle Scholar
Kapovich, M. and Kleiner, B., Coarse Alexander duality and duality groups, J. Differential Geom. 69 (2005), 279–352.Google Scholar
Kropholler, P. H. and Roller, M. A., Relative ends and duality groups, J. Pure Appl. Algebra 61 (1989), 197–210.Google Scholar
Lauer, J. and Wise, D. T., Cubulating one-relator groups with torsion, submitted, 2011.Google Scholar
Leary, I. J., A metric Kan–Thurston theorem, Preprint (2010), arXiv:1009.1540 [math.GR].Google Scholar
McCammond, J. P., Constructing non-positively curved spaces and groups, in Geometric and cohomological methods in group theory Durham, 2003, London Mathematical Society Lecture Note Series, vol. 358, eds Bridson, M. R., Kropholler, P. H. and Leary, I. J., (Cambridge University Press, Cambridge, 2009), 162–224.Google Scholar
Moussong, G., Hyperbolic coxeter groups. PhD thesis, Ohio State University, (1988).Google Scholar
Nevo, A. and Sageev, M., The Poisson boundary of CAT(0) cube complex groups, Preprint (2011), arXiv:1105.1675 [math.GT].Google Scholar
Nica, B., Cubulating spaces with walls, Algebr. Geom. Topol. 4 (2004), 297–309.CrossRefGoogle Scholar
Niblo, G. and Reeves, L., Groups acting on CAT(0) cube complexes, Geom. Topol. 1 (1997), 1–7.Google Scholar
Niblo, G. A. and Reeves, L. D., The geometry of cube complexes and the complexity of their fundamental groups, Topology 37 (1998), 621–633.Google Scholar
Niblo, G. A. and Reeves, L. D., Coxeter groups act on CAT(0) cube complexes, J. Group Theory 6 (2003), 399–413.CrossRefGoogle Scholar
Niblo, G. A. and Roller, M. A., Groups acting on cubes and Kazhdan’s property (T), Proc. Amer. Math. Soc. 126 (1998), 693–699.Google Scholar
Ollivier, Y. and Wise, D. T., Cubulating random groups at density less than 1/6, Trans. Amer. Math. Soc. 363 (2011), 4701–4733.Google Scholar
Osin, D. V., Relatively hyperbolic groups: intrinsic geometry, algebraic properties, and algorithmic problems, Mem. Amer. Math. Soc. 179 (2006).Google Scholar
Przytycki, P. and Wise, D. T., Mixed 3-manifolds are virtually special, Preprint (2012),arXiv:1205.6742 [math.GR].Google Scholar
Roller, M. A., Poc sets, median algebras and group actions: an extended study of Dunwoody’s construction and Sageev’s theorem, Preprint (1998), University of Southampton.Google Scholar
Rubinstein, H. and Sageev, M., Intersection patterns of essential surfaces in 3-manifolds, Topology 38 (1999), 1281–1291.Google Scholar
Rubinstein, J. H. and Wang, S., π 1-injective surfaces in graph manifolds, Comment. Math. Helv. 73 (1998), 499–515.Google Scholar
Sageev, M., Ends of group pairs and non-positively curved cube complexes, Proc. Lond. Math. Soc. (3) 71 (1995), 585–617.Google Scholar
Sageev, M., Codimension-1 subgroups and splittings of groups, J. Algebra 189 (1997), 377–389.Google Scholar
Sageev, M. and Wise, D. T., The Tits alternative for CAT(0) cubical complexes, Bull. Lond. Math. Soc. 37 (2005), 706–710.Google Scholar
Scott, P., Ends of pairs of groups, J. Pure Appl. Algebra 11 (1977), 179–198.Google Scholar
Scott, P., There are no fake Seifert fibre spaces with infinite π 1, Ann. of Math. (2) 117 (1983), 35–70.Google Scholar
Serre, J.-P.,Arbres, amalgames, SL 2, Astérisque, vol. 46 (Société Mathématique de France, Paris, 1977); written in collaboration with H. Bass.Google Scholar
Wise, D. T., Cubulating small cancellation groups, Geom. Funct. Anal 14 (2004), 150–214.Google Scholar
Wise, D. T., Subgroup separability of the figure 8 knot group, Topology 45 (2006), 421–463.Google Scholar
Wise, D. T., Research announcement: the structure of groups with a quasiconvex hierarchy, Electron. Res. Announc. Amer. Math. Soc. 16 (2009), 44–55.Google Scholar
Wise, D. T., Recubulating free groups, Israel J. Math. 191 (2012), 337–345.Google Scholar
Wright, N., Finite asymptotic dimension for CAT(0) cube complexes, Geom. Topol. 16 (2012), 527–554.Google Scholar