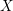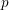Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Creutz, Brendan
2020.
There are No Transcendental Brauer–Manin Obstructions on Abelian Varieties.
International Mathematics Research Notices,
Vol. 2020,
Issue. 9,
p.
2684.