Hostname: page-component-cd9895bd7-q99xh Total loading time: 0 Render date: 2024-12-26T09:45:48.106Z Has data issue: false hasContentIssue false
  • English
  • Français

The homotopy theory of polyhedral products associated with flag complexes

Part of: Algebraic combinatorics Homotopy theory

Published online by Cambridge University Press:  23 November 2018

Taras Panov  and
Stephen Theriault
Taras Panov
Affiliation:
Department of Mathematics and Mechanics, Moscow State University, Leninskie Gory, 119991 Moscow, Russia Institute for Theoretical and Experimental Physics, Moscow, Russia Institute for Information Transmission Problems, Russian Academy of Sciences, Russia email tpanov@mech.math.msu.su
Stephen Theriault
Affiliation:
Mathematical Sciences, University of Southampton, Southampton SO17 1BJ, UK email S.D.Theriault@soton.ac.uk
Get access Rights & Permissions [Opens in a new window]

Abstract

If 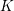$K$ is a simplicial complex on 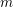$m$ vertices, the flagification of 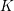$K$ is the minimal flag complex 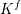$K^{f}$ on the same vertex set that contains 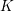$K$. Letting 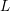$L$ be the set of vertices, there is a sequence of simplicial inclusions 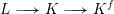$L\stackrel{}{\longrightarrow }K\stackrel{}{\longrightarrow }K^{f}$. This induces a sequence of maps of polyhedral products 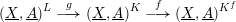$(\text{}\underline{X},\text{}\underline{A})^{L}\stackrel{g}{\longrightarrow }(\text{}\underline{X},\text{}\underline{A})^{K}\stackrel{f}{\longrightarrow }(\text{}\underline{X},\text{}\underline{A})^{K^{f}}$. We show that 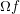$\unicode[STIX]{x1D6FA}f$ and $\unicode[STIX]{x1D6FA}f\circ \unicode[STIX]{x1D6FA}g$ have right homotopy inverses and draw consequences. For a flag complex 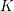$K$ the polyhedral product of the form 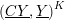$(\text{}\underline{CY},\text{}\underline{Y})^{K}$ is a co-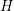$H$-space if and only if the 1-skeleton of 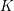$K$ is a chordal graph, and we deduce that the maps 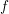$f$ and 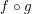$f\circ g$ have right homotopy inverses in this case.

Keywords

polyhedral productmoment-angle complexflag complex

MSC classification

Primary: 55P35: Loop spaces
Secondary: 05E45: Combinatorial aspects of simplicial complexes
Type
Research Article
Information
Compositio Mathematica , Volume 155 , Issue 1 , January 2019 , pp. 206 - 228
Copyright
© The Authors 2018 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Bahri, A., Bendersky, M., Cohen, F. R. and Gitler, S., The polyhedral product functor: a method of decomposition for moment-angle complexes, arrangements and related spaces , Adv. Math. 225 (2010), 1634–1668.Google Scholar
Bahri, A., Bendersky, M., Cohen, F. R. and Gitler, S., Cup-products for the polyhedral product functor , Math. Proc. Cambridge Philos. Soc. 153 (2012), 457–469.Google Scholar
Buchstaber, V. M. and Panov, T. E., Torus actions and their applications in topology and combinatorics, University Lecture Series, vol. 24 (American Mathematical Society, Providence, RI, 2002).Google Scholar
Buchstaber, V. M. and Panov, T. E., Toric topology, Mathematical Surveys and Monographs, vol. 204 (American Mathematical Society, Providence, RI, 2015).Google Scholar
Félix, Y. and Tanré, D., Rational homotopy of the polyhedral product functor , Proc. Amer. Math. Soc. 137 (2009), 891–898.Google Scholar
Fulkerson, D. R. and Gross, O., Incidence matrices and interval graphs , Pacific J. Math 15 (1965), 835–855.Google Scholar
Ganea, T., Cogroups and suspensions , Invent. Math. 9 (1970), 185–197.Google Scholar
Grbić, J., Panov, T., Theriault, S. and Wu, J., The homotopy types of moment-angle complexes for flag complexes , Trans. Amer. Math. Soc. 368 (2016), 6663–6682.Google Scholar
Grbić, J. and Theriault, S., The homotopy type of the complement of a coordinate subspace arrangement , Topology 46 (2007), 357–396.Google Scholar
Grbić, J. and Theriault, S., The homotopy type of the polyhedral product for shifted complexes , Adv. Math. 245 (2013), 690–715.Google Scholar
Grbić, J. and Theriault, S., Homotopy theory in toric topology , Russian Math. Surveys 71 (2016), 185–251.Google Scholar
Iriye, K. and Kishimoto, D., Fat wedge filtration and decomposition of polyhedral products, Kyoto J. Math., doi:10.1215/21562261-2017-0038.Google Scholar
Mather, M., Pull-backs in homotopy theory , Canad. J. Math. 28 (1976), 225–263.Google Scholar
Milnor, J., On spaces having the homotopy type of a CW-complex , Trans. Amer. Math. Soc. 90 (1959), 272–280.Google Scholar
Panov, T. and Veryovkin, Y., Polyhedral products and commutator subgroups of right-angled Artin and Coxeter groups , Mat. Sb. 207 (2016), 105–126 (in Russian); Sb. Math. 207 (2016), 1582–1600 (Engl. transl.).Google Scholar
Porter, G. J., The homotopy groups of wedges of suspensions , Amer. J. Math. 88 (1966), 655–663.Google Scholar
Selick, P., Introduction to homotopy theory, Fields Institute Monographs, vol. 9 (American Mathematical Society, Providence, RI, 1997).Google Scholar
Spanier, E. H., Algebraic topology (McGraw-Hill, New York, Toronto, London, 1966).Google Scholar
Theriault, S., The dual polyhedral product, cocategory and nilpotence , Adv. Math. 340 (2018), 138–192.Google Scholar