Hostname: page-component-78c5997874-8bhkd Total loading time: 0 Render date: 2024-11-10T20:53:49.675Z Has data issue: false hasContentIssue false
  • English
  • Français

A Lie-theoretic interpretation of multivariate hypergeometric polynomials

Part of: Lie algebras and Lie superalgebras Difference equations Difference and functional equations

Published online by Cambridge University Press:  20 March 2012

Plamen Iliev
Plamen Iliev*
Affiliation:
School of Mathematics, Georgia Institute of Technology, Atlanta, GA 30332-0160, USA (email: iliev@math.gatech.edu)
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

In 1971, Griffiths used a generating function to define polynomials in d variables orthogonal with respect to the multinomial distribution. The polynomials possess a duality between the discrete variables and the degree indices. In 2004, Mizukawa and Tanaka related these polynomials to character algebras and the Gelfand hypergeometric series. Using this approach, they clarified the duality and obtained a new proof of the orthogonality. In the present paper, we interpret these polynomials within the context of the Lie algebra . 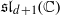Our approach yields yet another proof of the orthogonality. It also shows that the polynomials satisfy d independent recurrence relations each involving d2+d+1 terms. This, combined with the duality, establishes their bispectrality. We illustrate our results with several explicit examples.

Keywords

multivariate Krawtchouk polynomialsthe Lie algebra 𝔰𝔩d+1partial difference operatorsthe bispectral problem

MSC classification

Secondary: 33C80: Connections with groups and algebras, and related topics 17B10: Representations, algebraic theory (weights) 39A14: Partial difference equations
Type
Research Article
Information
Compositio Mathematica , Volume 148 , Issue 3 , May 2012 , pp. 991 - 1002
Copyright
Copyright © Foundation Compositio Mathematica 2012

References

[BI84]Bannai, E. and Ito, T., Algebraic combinatorics. I. Association schemes (Benjamin/Cummings, Menlo Park, CA, 1984).Google Scholar
[DS02]Dougherty, S. T. and Skriganov, M. M., MacWilliams duality and the Rosenbloom–Tsfasman metric, Mosc. Math. J. 2 (2002), 81–97.Google Scholar
[DG86]Duistermaat, J. J. and Grünbaum, F. A., Differential equations in the spectral parameter, Comm. Math. Phys. 103 (1986), 177–240.Google Scholar
[DX01]Dunkl, C. F. and Xu, Y., Orthogonal polynomials of several variables, Encyclopedia of Mathematics and its Applications, vol. 81 (Cambridge University Press, Cambridge, 2001).CrossRefGoogle Scholar
[GI10]Geronimo, J. and Iliev, P., Bispectrality of multivariable Racah–Wilson polynomials, Constr. Approx. 31 (2010), 417–457.CrossRefGoogle Scholar
[Gri71]Griffiths, R. C., Orthogonal polynomials on the multinomial distribution, Austral. J. Statist. 13 (1971), 27–35.Google Scholar
[Gru07]Grünbaum, F. A., The Rahman polynomials are bispectral, SIGMA Symmetry Integrability Geom. Methods Appl. 3 (2007) 11 (Paper 065).Google Scholar
[GR10]Grünbaum, F. A. and Rahman, M., On a family of 2-variable orthogonal Krawtchouk polynomials, SIGMA Symmetry Integrability Geom. Methods Appl. 6 (2010) 12 (Paper 090).Google Scholar
[HR08]Hoare, M. R. and Rahman, M., A probabilistic origin for a new class of bivariate polynomials, SIGMA Symmetry Integrability Geom. Methods Appl. 4 (2008) 18 (Paper 089).Google Scholar
[IT]Iliev, P. and Terwilliger, P., The Rahman polynomials and the Lie algebra , Trans. Amer. Math. Soc., to appear.Google Scholar
[IX07]Iliev, P. and Xu, Y., Discrete orthogonal polynomials and difference equations of several variables, Adv. Math. 212 (2007), 1–36.CrossRefGoogle Scholar
[Mil68]Milch, P. R., A multi-dimensional linear growth birth and death process, Ann. Math. Statist. 39 (1968), 727–754.Google Scholar
[MT04]Mizukawa, H. and Tanaka, H., (n+1,m+1)-hypergeometric functions associated to character algebras, Proc. Amer. Math. Soc. 132 (2004), 2613–2618.CrossRefGoogle Scholar
[Ter01]Terwilliger, P., Two linear transformations each tridiagonal with respect to an eigenbasis of the other, Linear Algebra Appl. 330 (2001), 149–203.Google Scholar
[Ter06]Terwilliger, P., An algebraic approach to the Askey scheme of orthogonal polynomials, in Orthogonal polynomials and special functions, Lecture Notes in Mathematics, vol. 1883 (Springer, Berlin, 2006), 255–330.Google Scholar