Hostname: page-component-cd9895bd7-gxg78 Total loading time: 0 Render date: 2024-12-26T09:44:26.209Z Has data issue: false hasContentIssue false
  • English
  • Français

Local Langlands correspondence and ramification for Carayol representations

Part of: Algebraic number theory: local and $p$-adic fields Lie groups

Published online by Cambridge University Press:  06 September 2019

Colin J. Bushnell  and
Guy Henniart
Colin J. Bushnell
Affiliation:
King’s College London, Department of Mathematics, Strand, London WC2R 2LS, UK email colin.bushnell@kcl.ac.uk
Guy Henniart
Affiliation:
Laboratoire de Mathématiques d’Orsay, Université Paris-Sud, CNRS, Université Paris-Saclay, 91405 Orsay, France email Guy.Henniart@math.u-psud.fr
Get access Rights & Permissions [Opens in a new window]

Abstract

Let 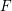$F$ be a non-Archimedean locally compact field of residual characteristic 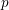$p$ with Weil group 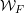${\mathcal{W}}_{F}$. Let $\unicode[STIX]{x1D70E}$ be an irreducible smooth complex representation of 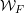${\mathcal{W}}_{F}$, realized as the Langlands parameter of an irreducible cuspidal representation $\unicode[STIX]{x1D70B}$ of a general linear group over 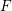$F$. In an earlier paper we showed that the ramification structure of $\unicode[STIX]{x1D70E}$ is determined by the fine structure of the endo-class 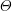$\unicode[STIX]{x1D6E9}$ of the simple character contained in $\unicode[STIX]{x1D70B}$, in the sense of Bushnell and Kutzko. The connection is made via the Herbrand function $\unicode[STIX]{x1D6F9}_{\unicode[STIX]{x1D6E9}}$ of 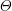$\unicode[STIX]{x1D6E9}$. In this paper we concentrate on the fundamental Carayol case in which $\unicode[STIX]{x1D70E}$ is totally wildly ramified with Swan exponent not divisible by 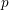$p$. We show that, for such $\unicode[STIX]{x1D70E}$, the associated Herbrand function satisfies a certain functional equation, and that this property essentially characterizes this class of representations. We calculate $\unicode[STIX]{x1D6F9}_{\unicode[STIX]{x1D6E9}}$ explicitly, in terms of a classical Herbrand function arising naturally from the classification of simple characters. We describe exactly the class of functions arising as Herbrand functions 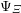$\unicode[STIX]{x1D6F9}_{\unicode[STIX]{x1D6EF}}$, as 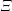$\unicode[STIX]{x1D6EF}$ varies over the set of totally wild endo-classes of Carayol type. In a separate argument, we derive a complete description of the restriction of $\unicode[STIX]{x1D70E}$ to any ramification subgroup and hence a detailed interpretation of the Herbrand function. This gives concrete information concerning the Langlands correspondence.

Keywords

local Langlands correspondencecuspidal representationsimple characterendo-classramification groupHerbrand functionCarayol representation

MSC classification

Primary: 22E50: Representations of Lie and linear algebraic groups over local fields 11S37: Langlands-Weil conjectures, nonabelian class field theory 11S15: Ramification and extension theory
Type
Research Article
Information
Compositio Mathematica , Volume 155 , Issue 10 , October 2019 , pp. 1959 - 2038
Copyright
© The Authors 2019 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Arthur, J. and Clozel, L., Simple algebras, base change, and the advanced theory of the trace formula, Annals of Mathematics Studies, vol. 129 (Princeton University Press, Princeton, NJ, 1989).Google Scholar
Bushnell, C. J., Effective local Langlands correspondence , in Automorphic forms and Galois representations, Vol. 1, London Mathematical Society Lecture Notes, vol. 414, eds Diamond, F., Kassei, P. L. and Kim, M. (Cambridge University Press, Cambridge, 2014), 102–134.Google Scholar
Bushnell, C. J. and Henniart, G., Local tame lifting for GL(N) I: Simple characters , Publ. Math. Inst. Hautes Études Sci. 83 (1996), 105–233.Google Scholar
Bushnell, C. J. and Henniart, G., Local tame lifting for GL(n) II: Wildly ramified supercuspidals , Astérisque 254 (1999).Google Scholar
Bushnell, C. J. and Henniart, G., Local tame lifting for GL(n) IV: Simple characters and base change , Proc. Lond. Math. Soc. 87 (2003), 337–362.Google Scholar
Bushnell, C. J. and Henniart, G., The essentially tame local Langlands correspondence, I , J. Amer. Math. Soc. 18 (2005), 685–710.Google Scholar
Bushnell, C. J. and Henniart, G., The essentially tame local Langlands correspondence, II: Totally ramified representations , Compos. Math. 141 (2005), 979–1011.Google Scholar
Bushnell, C. J. and Henniart, G., The local Langlands conjecture for GL(2), Grundlehren der Mathematischen Wissenschaften, vol. 335 (Springer, Berlin, 2006).Google Scholar
Bushnell, C. J. and Henniart, G., The essentially tame local Langlands correspondence, III: The general case , Proc. Lond. Math. Soc. (3) 101 (2010), 497–553.Google Scholar
Bushnell, C. J. and Henniart, G., Intertwining of simple characters in GL(n) , Int. Math. Res. Not. IMRN 17 (2013), 3977–3987.Google Scholar
Bushnell, C. J. and Henniart, G., Langlands parameters for epipelagic representations of GLn , Math. Ann. 358 (2014), 433–463.Google Scholar
Bushnell, C. J. and Henniart, G., To an effective local Langlands correspondence , Mem. Amer. Math. Soc. 231 (2014), no. 1087.Google Scholar
Bushnell, C. J. and Henniart, G., Higher ramification and the local Langlands correspondence , Ann. of Math. (2) 185 (2017), 919–955.Google Scholar
Bushnell, C. J., Henniart, G. and Kutzko, P. C., Local Rankin-Selberg convolutions for GLn: Explicit conductor formula , J. Amer. Math. Soc. 11 (1998), 703–730.Google Scholar
Bushnell, C. J. and Kutzko, P. C., The admissible dual of GL(N) via compact open subgroups, Annals of Mathematics Studies, vol. 129 (Princeton University Press, Princeton, NJ, 1993).Google Scholar
Bushnell, C. J. and Kutzko, P. C., Simple types in GL(N): computing conjugacy classes , Contemp. Math. 177 (1994), 107–135.Google Scholar
Carayol, H., Représentations cuspidales du groupe linéaire , Ann. Sci. Éc. Norm. Supér. (4) 17 (1984), 191–225.Google Scholar
Deligne, P., Les corps locaux de caractéristique p, limite de corps locaux de caractéristique 0. Appendice: théorie de la ramification, et fonctions de Herbrand, pour des extensions non galoisiennes , in Représentations des groupes réductifs sur un corps local (Hermann, Paris, 1984), 150–157.Google Scholar
Gorenstein, D., Finite groups (AMS Chelsea Publishing, Providence, RI, 2000).Google Scholar
Harris, M. and Taylor, R., On the geometry and cohomology of some simple Shimura varieties, Annals of Mathematics Studies, vol. 151 (Princeton University Press, Princeton, NJ, 2001).Google Scholar
Heiermann, V., Sur l’espace des représentations irréductibles du groupe de Galois d’un corps local , C. R. Acad. Sci. Paris Sér. I Math. 323 (1996), 571–576.Google Scholar
Henniart, G., Représentations du groupe de Weil d’un corps local , Enseign. Math. Sér II 26 (1980), 155–172.Google Scholar
Henniart, G., La Conjecture de Langlands pour GL(3) , Mém. Soc. Math. Fr. (N.S.) 11–12 (1984).Google Scholar
Henniart, G., Caractérisation de la correspondance de Langlands par les facteurs 𝜀 de paires , Invent. Math. 113 (1993), 339–350.Google Scholar
Henniart, G., Une preuve simple des conjectures locales de Langlands pour GLn sur un corps p-adique , Invent. Math. 139 (2000), 439–455.Google Scholar
Henniart, G. and Herb, R., Automorphic induction for GL(n) (over local non-archimedean fields) , Duke Math. J. 78 (1995), 131–192.Google Scholar
Henniart, G. and Lemaire, B., Formules de caractères pour l’induction automorphe , J. Reine Angew. Math. 645 (2010), 41–84.Google Scholar
Henniart, G. and Lemaire, B., Changement de base et induction automorphe pour GLn en caractéristique non nulle , Mém. Soc. Math. Fr. (N.S.) 124 (2011).Google Scholar
Jacquet, H., Piatetski-Shapiro, I. and Shalika, J., Rankin-Selberg convolutions , Amer. J. Math. 105 (1983), 367–483.Google Scholar
Kazhdan, D., On lifting , in Lie group representations II, Lecture Notes in Mathematics, vol. 1041 (Springer, New York, 1984), 209–249.Google Scholar
Kutzko, P. C., The irreducible imprimitive local Galois representations of prime dimension , J. Algebra 57 (1979), 101–110.Google Scholar
Kutzko, P. C., The Langlands conjecture for GL2 of a local field , Ann. of Math. (2) 112 (1980), 381–412.Google Scholar
Kutzko, P. C., The exceptional representations of GL2 , Compos. Math. 51 (1984), 3–14.Google Scholar
Kutzko, P. C. and Moy, A., On the local Langlands conjecture in prime dimension , Ann. of Math. (2) 121 (1985), 495–517.Google Scholar
Laumon, G., Rapoport, M. and Stuhler, U., 𝓓-elliptic sheaves and the Langlands correspondence , Invent. Math. 113 (1993), 217–338.Google Scholar
Mœglin, C., Sur la correspondance de Langlands-Kazhdan , J. Math. Pures Appl. (9) 69 (1990), 175–226.Google Scholar
Scholze, P., The local Langlands correspondence for GLn over p-adic fields , Invent. Math. 192 (2013), 663–715.Google Scholar
Serre, J.-P., Corps locaux (Hermann, Paris, 1968).Google Scholar
Shahidi, F., Fourier transforms of intertwining operators and Plancherel measures for GL(n) , Amer. J. Math. 106 (1984), 67–111.Google Scholar
Zink, E.-W., U 1 -Konjugationsklassen in lokalen Divisionsalgebren , Math. Nachr. 137 (1988), 283–320.Google Scholar
Zink, E.-W., Irreducible polynomials over local fields and higher ramification theory in local Langlands theory , Contemp. Math. 131 (1992 (part 2)), 529–563.Google Scholar