Article contents
Most odd-degree binary forms fail to primitively represent a square
Published online by Cambridge University Press: 08 February 2024
Abstract
Let  $F$ be a separable integral binary form of odd degree
$F$ be a separable integral binary form of odd degree 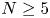 $N \geq 5$. A result of Darmon and Granville known as ‘Faltings plus epsilon’ implies that the degree-
$N \geq 5$. A result of Darmon and Granville known as ‘Faltings plus epsilon’ implies that the degree-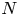 $N$ superelliptic equation
$N$ superelliptic equation 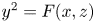 $y^2 = F(x,z)$ has finitely many primitive integer solutions. In this paper, we consider the family
$y^2 = F(x,z)$ has finitely many primitive integer solutions. In this paper, we consider the family  $\mathscr {F}_N(f_0)$ of degree-
$\mathscr {F}_N(f_0)$ of degree-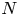 $N$ superelliptic equations with fixed leading coefficient
$N$ superelliptic equations with fixed leading coefficient 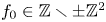 $f_0 \in \mathbb {Z} \smallsetminus \pm \mathbb {Z}^2$, ordered by height. For every sufficiently large
$f_0 \in \mathbb {Z} \smallsetminus \pm \mathbb {Z}^2$, ordered by height. For every sufficiently large 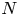 $N$, we prove that among equations in the family
$N$, we prove that among equations in the family 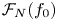 $\mathscr {F}_N(f_0)$, more than
$\mathscr {F}_N(f_0)$, more than  $74.9\,\%$ are insoluble, and more than
$74.9\,\%$ are insoluble, and more than 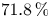 $71.8\,\%$ are everywhere locally soluble but fail the Hasse principle due to the Brauer–Manin obstruction. We further show that these proportions rise to at least
$71.8\,\%$ are everywhere locally soluble but fail the Hasse principle due to the Brauer–Manin obstruction. We further show that these proportions rise to at least 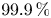 $99.9\,\%$ and
$99.9\,\%$ and 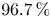 $96.7\,\%$, respectively, when
$96.7\,\%$, respectively, when  $f_0$ has sufficiently many prime divisors of odd multiplicity. Our result can be viewed as a strong asymptotic form of ‘Faltings plus epsilon’ for superelliptic equations and constitutes an analogue of Bhargava's result that most hyperelliptic curves over
$f_0$ has sufficiently many prime divisors of odd multiplicity. Our result can be viewed as a strong asymptotic form of ‘Faltings plus epsilon’ for superelliptic equations and constitutes an analogue of Bhargava's result that most hyperelliptic curves over 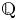 $\mathbb {Q}$ have no rational points.
$\mathbb {Q}$ have no rational points.
Information
- Type
- Research Article
- Information
- Copyright
- © 2024 The Author(s). The publishing rights in this article are licensed to Foundation Compositio Mathematica under an exclusive licence
References
- 1
- Cited by


