Published online by Cambridge University Press: 09 December 2019
We compute the 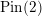 $\text{Pin}(2)$-equivariant Seiberg–Witten Floer homology of Seifert rational homology three-spheres in terms of their Heegaard Floer homology. As a result of this computation, we prove Manolescu’s conjecture that
$\text{Pin}(2)$-equivariant Seiberg–Witten Floer homology of Seifert rational homology three-spheres in terms of their Heegaard Floer homology. As a result of this computation, we prove Manolescu’s conjecture that 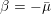 $\unicode[STIX]{x1D6FD}=-\bar{\unicode[STIX]{x1D707}}$ for Seifert integral homology three-spheres. We show that the Manolescu invariants
$\unicode[STIX]{x1D6FD}=-\bar{\unicode[STIX]{x1D707}}$ for Seifert integral homology three-spheres. We show that the Manolescu invariants  $\unicode[STIX]{x1D6FC},\unicode[STIX]{x1D6FD},$ and
$\unicode[STIX]{x1D6FC},\unicode[STIX]{x1D6FD},$ and 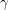 $\unicode[STIX]{x1D6FE}$ give new obstructions to homology cobordisms between Seifert fiber spaces, and that many Seifert homology spheres
$\unicode[STIX]{x1D6FE}$ give new obstructions to homology cobordisms between Seifert fiber spaces, and that many Seifert homology spheres  $\unicode[STIX]{x1D6F4}(a_{1},\ldots ,a_{n})$ are not homology cobordant to any
$\unicode[STIX]{x1D6F4}(a_{1},\ldots ,a_{n})$ are not homology cobordant to any 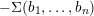 $-\unicode[STIX]{x1D6F4}(b_{1},\ldots ,b_{n})$. We then use the same invariants to give an example of an integral homology sphere not homology cobordant to any Seifert fiber space. We also show that the
$-\unicode[STIX]{x1D6F4}(b_{1},\ldots ,b_{n})$. We then use the same invariants to give an example of an integral homology sphere not homology cobordant to any Seifert fiber space. We also show that the 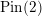 $\text{Pin}(2)$-equivariant Seiberg–Witten Floer spectrum provides homology cobordism obstructions distinct from
$\text{Pin}(2)$-equivariant Seiberg–Witten Floer spectrum provides homology cobordism obstructions distinct from 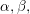 $\unicode[STIX]{x1D6FC},\unicode[STIX]{x1D6FD},$ and
$\unicode[STIX]{x1D6FC},\unicode[STIX]{x1D6FD},$ and 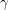 $\unicode[STIX]{x1D6FE}$. In particular, we identify an
$\unicode[STIX]{x1D6FE}$. In particular, we identify an 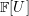 $\mathbb{F}[U]$-module called connected Seiberg–Witten Floer homology, whose isomorphism class is a homology cobordism invariant.
$\mathbb{F}[U]$-module called connected Seiberg–Witten Floer homology, whose isomorphism class is a homology cobordism invariant.
MS was supported by NSF Grant DMS-1702532.