Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Templier, Nicolas
and
Tsimerman, Jacob
2013.
Non-split sums of coefficients of GL(2)-automorphic forms.
Israel Journal of Mathematics,
Vol. 195,
Issue. 2,
p.
677.
Burungale, Ashay A.
and
Tian, Ye
2020.
Horizontal non-vanishing of Heegner points and toric periods.
Advances in Mathematics,
Vol. 362,
Issue. ,
p.
106938.
Nordentoft, Asbjørn Christian
2024.
Wide moments of L-functions I : Twists by class
group characters of imaginary quadratic fields.
Algebra & Number Theory,
Vol. 18,
Issue. 4,
p.
735.
Constantinescu, Petru
and
Nordentoft, Asbjørn Christian
2025.
Non-vanishing of Geodesic Periods of Automorphic Forms.
Geometric and Functional Analysis,
Vol. 35,
Issue. 4,
p.
1108.

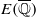 contains Heegner points of discriminant
contains Heegner points of discriminant  contient des points de Heegner de discriminant
contient des points de Heegner de discriminant 