Article contents
XLIV.—On the Theory of Isomeric Compounds
Published online by Cambridge University Press: 17 January 2013
Extract
In the following remarks I intend to confine myself to the consideration of those compounds which have not only the same composition per cent., and the same molecular weight, but also the same constitutional formula. Such compounds may be termed absolutely isomeric. As the constitutional formula of few substances is fully known, this class is of course a small one, or rather there are few substances of which we can certainly say that they belong to this class.
- Type
- Research Article
- Information
- Earth and Environmental Science Transactions of The Royal Society of Edinburgh , Volume 23 , Issue 3 , 1864 , pp. 707 - 719
- Copyright
- Copyright © Royal Society of Edinburgh 1864
References
page 708 note * I may here shortly explain the graphic notation which I employ to express constitutional formulæ, and by which, it is scarcely necessary to remark, I do not mean to indicate the physical, but merely the chemical position of the atoms. An atom is represented by its usual symbol, surrounded by a circle with as many lines proceeding from it as the atom contains equivalents, thus an unequivalent atom is represented by ![]() , a biequivalent atom by
, a biequivalent atom by ![]() , and so on of the others. When equivalents mutually saturate one another, the two lines representing the equivalents are made continuations of one another, thus water is
, and so on of the others. When equivalents mutually saturate one another, the two lines representing the equivalents are made continuations of one another, thus water is ![]() . Formic acid
. Formic acid

This method seems to me to present advantages over the methods used by Professors Kekulé and Erlenmeyer; and while it is no doubt liable, when not explained, to be mistaken for a representation of the physical position of the atoms, this misunderstanding can easily be prevented.
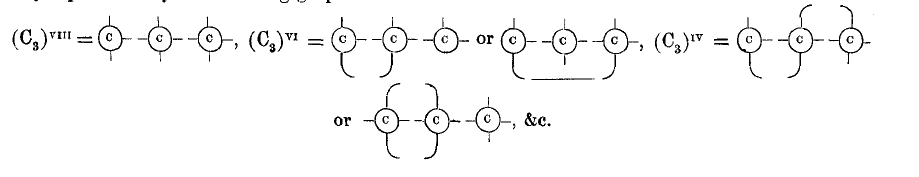
page 709 note * I do not intend to deny the possibility of this, but all we know of such “non-saturated” substances leads to the belief that the atomicity of the carbon radical Cn is reduced, not by one or more of the carbon atoms becoming diatomic, but by the union of the carbon atoms taking place in the way represented by the following graphic formulæ:—
page 711 note * It would be interesting to compare the properties of the tartaric acid formed from isobibromo-succinic acid with that from bibromo-succinic, and with the varieties obtained from the grape.
page 714 note * I do not here notice the remarks of Professor Kolbe (Zeitschrift, vi. 13) on the same subject, as his object is rather to prove the metamerism than to explain the isomerism of these bodies.
page 715 note * Butlerow notices this disturbing influence (Zeitschrift, vi. 516) as opposing an obstacle, which he seems to regard as for the present insuperable, in the way of determining whether a difference. exist or not among the equivalents of a multequivalent atom.
page 716 note * The term component is, of course, not used here in its strictly dynamical sense, what is meant is, that the total force uniting a pair of equivalents, is a function of two quantities, the one depending on the structure of the molecule, and the position in it of the two equivalents, and the other on the chemical nature of the two equivalents.
page 717 note * I use the terms intra- and extra- radical affinities as abbreviations for the carbon affinities, with which the intra- and extra- radical oxygen atoms are combined.
page 717 note † Loc. cit.
page 717 note ‡ In order to elucidate this passage as much as possible, I append the graphic representations referred to:—

page 718 note * “It may of course equally well be assumed, that the carbon atoms are, as it were, pushed together (zusammengeschoben), so that two carbon atoms are united by two affinities of each. This is only another form of the same idea.”
page 718 note † See Erlenmeyer, Zeitschrift, vi. 21.
page 718 note ‡ See Butlerow, Zeitschrift, vi. 524.
- 59
- Cited by


