Refine listing
Actions for selected content:
Most cited
This page lists all time most cited articles for this title. Please use the publication date filters on the left if you would like to restrict this list to recently published content, for example to articles published in the last three years. The number of times each article was cited is displayed to the right of its title and can be clicked to access a list of all titles this article has been cited by.
Contents
- Cited by 16
A Bounds Test for Equality Between Sets of Coefficients in Two Linear Regression Models Under Heteroscedasticity
-
- Published online by Cambridge University Press:
- 18 October 2010, pp. 220-231
-
- Article
- Export citation
- Cited by 16
On the Asymptotic Optimality of Alternative Minimum-Distance Estimators in Linear Latent-Variable Models
-
- Published online by Cambridge University Press:
- 11 February 2009, pp. 867-883
-
- Article
- Export citation
- Cited by 16
POINT DECISIONS FOR INTERVAL–IDENTIFIED PARAMETERS
-
- Published online by Cambridge University Press:
- 29 January 2014, pp. 334-356
-
- Article
- Export citation
- Cited by 16
ROBUST INFERENCE FOR THE MEAN IN THE PRESENCE OF SERIAL CORRELATION AND HEAVY-TAILED DISTRIBUTIONS
-
- Published online by Cambridge University Press:
- 17 July 2002, pp. 1019-1039
-
- Article
- Export citation
- Cited by 16
ON THE JACKKNIFE-AFTER-BOOTSTRAP METHOD FOR DEPENDENT DATA AND ITS CONSISTENCY PROPERTIES
-
- Published online by Cambridge University Press:
- 06 March 2002, pp. 79-98
-
- Article
- Export citation
- Cited by 16
A DIALOGUE CONCERNING A NEW INSTRUMENT FOR ECONOMETRIC MODELING
-
- Published online by Cambridge University Press:
- 08 February 2005, pp. 278-297
-
- Article
- Export citation
- Cited by 16
Mirror-Image and Invariant Distributions in ARMA Models
-
- Published online by Cambridge University Press:
- 18 October 2010, pp. 36-52
-
- Article
- Export citation
- Cited by 16
WORLDWIDE INSTITUTIONAL RANKINGS IN ECONOMETRICS: 1989–1995
-
- Published online by Cambridge University Press:
- 01 February 1998, pp. 1-43
-
- Article
- Export citation
- Cited by 16
ALTERNATIVE FREQUENCY AND TIME DOMAIN VERSIONS OF FRACTIONAL BROWNIAN MOTION
-
- Published online by Cambridge University Press:
- 06 September 2007, pp. 256-293
-
- Article
- Export citation
- Cited by 16
SEMIPARAMETRIC ESTIMATION OF NONSTATIONARY CENSORED PANEL DATA MODELS WITH TIME VARYING FACTOR LOADS
-
- Published online by Cambridge University Press:
- 14 May 2008, pp. 1149-1173
-
- Article
- Export citation
- Cited by 16
WEAK CONVERGENCE TO STOCHASTIC INTEGRALS FOR ECONOMETRIC APPLICATIONS
-
- Published online by Cambridge University Press:
- 24 July 2015, pp. 1349-1375
-
- Article
- Export citation
- Cited by 16
ESTIMATION OF CHANGE-POINTS IN LINEAR AND NONLINEAR TIME SERIES MODELS
-
- Published online by Cambridge University Press:
- 04 December 2014, pp. 402-430
-
- Article
- Export citation
- Cited by 16
EFFICIENT SEMIPARAMETRIC SEEMINGLY UNRELATED QUANTILE REGRESSION ESTIMATION
-
- Published online by Cambridge University Press:
- 01 October 2009, pp. 1392-1414
-
- Article
- Export citation
- Cited by 16
EFFICIENCY IN LARGE DYNAMIC PANEL MODELS WITH COMMON FACTORS
-
- Published online by Cambridge University Press:
- 16 April 2014, pp. 961-1020
-
- Article
- Export citation
- Cited by 16
IDENTIFYING LATENT GROUPED PATTERNS IN COINTEGRATED PANELS
-
- Published online by Cambridge University Press:
- 22 July 2019, pp. 410-456
-
- Article
- Export citation
- Cited by 16
Econometric Analysis of Panel DataBadi H. Baltagi Wiley, 1995
-
- Published online by Cambridge University Press:
- 11 February 2009, pp. 747-754
-
- Article
- Export citation
- Cited by 16
A SMOOTH TEST FOR THE EQUALITY OF DISTRIBUTIONS
-
- Published online by Cambridge University Press:
- 30 July 2012, pp. 419-446
-
- Article
- Export citation
- Cited by 16
NONPARAMETRIC IDENTIFICATION OF ACCELERATED FAILURE TIME COMPETING RISKS MODELS
-
- Published online by Cambridge University Press:
- 21 February 2013, pp. 905-919
-
- Article
- Export citation
- Cited by 16
ROBUST FORECAST COMPARISON
-
- Published online by Cambridge University Press:
- 27 October 2016, pp. 1306-1351
-
- Article
- Export citation
- Cited by 16
PARTIALLY LINEAR MODELS WITH UNIT ROOTS
-
- Published online by Cambridge University Press:
- 22 August 2005, pp. 877-906
-
- Article
- Export citation
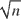 -consistent and asymptotically normal. The estimation procedure is also useful in the group effect setting, where estimation of the factor load would be empirically relevant in the study of the intergenerational correlation in income, explored in Solon (1992,
-consistent and asymptotically normal. The estimation procedure is also useful in the group effect setting, where estimation of the factor load would be empirically relevant in the study of the intergenerational correlation in income, explored in Solon (1992, 